Enhancement of feasibility of macroscopic quantum superposition state with the quantum Rabi-Stark model

Jing-Jing Wang, Ming-Song Ding, Li Xiong and Li Zheng

1 Basic Courses Teaching Department, Dalian Polytechnic University, Dalian 116034, China

2 Research Institute of Photonics, Dalian Polytechnic University, Dalian 116034, China

Abstract We propose an efficient scheme to generate a macroscopical quantum superposition state with a cavity optomechanical system,which is composed of a quantum Rabi-Stark model coupling to a mechanical oscillator.In a low-energy subspace of the Rabi-Stark model,the dressed states and then the effective Hamiltonian of the system are given.Due to the coupling of the mechanical oscillator and the atom-cavity system, if the initial state of the atom-cavity system is one of the dressed states,the mechanical oscillator will evolve into a corresponding coherent state.Thus,if the initial state of the atom-cavity system is a superposition of two dressed states,a coherent state superposition of the mechanical oscillator can be generated.The quantum coherence and their distinguishable properties of the two coherent states are exhibited by Wigner distribution.We show that the Stark term can enhance significantly the feasibility and quantum coherence of the generated macroscopic quantum superposition state of the oscillator.

Keywords: macroscopic quantum superposition, quantum optomechanics, Rabi-Stark model,Wigner function, quantum coherence

1.Introduction

Quantum optomechanics techniques now provide a new approach for mechanical resonators as novel systems for quantum science.During the 1990s, several aspects of quantum cavity optomechanical systems started to be explored theoretically [1].It was also pointed out that for extremely strong optomechanical coupling the resulting quantum nonlinearities could give rise to nonclassical and entangled states of the light field and the mechanics [2].

Recently optomechanical systems have been used to study the quantum effects of macroscopic objects.A scheme to prepare a macroscopic mechanical oscillator in a cat-like state is proposed using state-of-the-art photonic crystal optomechanical system[3].The investigation discussed in[4]shows that the use of squeezed states can significantly lengthen the lifetime of the cat states and improves the feasibility of generating cat states of large amplitude and with a greater degree of quantum macroscopic coherence.They show that the thermal decoherence of the dynamical cat states can be inhibited by a careful control of the squeezing of the reservoir.A feasible scheme in pulsed cavity optomechanics to generate the cat-like states of a macroscopic mechanical resonator is proposed by [5].This approach can be used to produce non-Gaussian mechanical states, like optical-catalysis nonclassical states.Qin et al [6]proposed to create and stabilize long-lived macroscopic quantum superposition states in atomic ensembles,which opens up a new possibility towards the long-standing goal of generating large-size and long-lived cat states.

In the cavity quantum electrodynamics (QED), the interaction between a two-level atom and a quantum light field can be described by the quantum Rabi model(QRM).If the coupling strength between the atom and the field is quite weak,the Jaynes–Cummings model can be efficient.With the progress of the experimental techniques, the ultra-strong coupling strength regimes can be implemented in some solidstate devices such as superconducting circuits [7–11],quantum wells[12],cold atoms[13].Recently,the QRM with arbitrary parameters has been realized in quantum simulations[14, 15], and the proposed scheme [14] shows that for ultrastrong coupling,a nonlinear coupling can also emerge,beside the linear dipole coupling between atom and field.This generalized model is called the quantum Rabi-Stark model(QRSM), for the nonlinear coupling term is associated with the dynamical Stark shift in the quantum optics.

QRSM has also attracted much attention in recent years[16–21].Theoretically, the QRSM has been studied by the Bargmann space approach[16]and solved by the Bogoliubov operator approach [17].Ref.[17] have given the G-function for the quantum Rabi-Stark model in a compact way, and declared that the regular spectrum can be determined by the zeros of the G-function.

In one of our previous works,we have proposed a scheme to prepare a macroscopically distinct mechanical superposition states of the mechanical oscillator using the QRM [22].However, the distinguishable feature of the generated state is not strong.In this paper,we propose a new scheme to generate large-size cat states using the QRSM.The rest of this paper is organized as follows.In section 2, we will introduce the physical model and its Hamiltonian.An effective Hamiltonian will be derived to depict the evolution of the system.In section 3,we will study the preparation of the macroscopic quantum superposition state of the mechanical oscillator.Moreover, we will give a demonstration of the enhancement of the generated states by Wigner Function in section 4.Finally, we will give some discussions in section 5.

2.Model

Considering a cavity-QED system which consists of a twolevel atom,single-mode Fabry–Perot cavity and a mechanical oscillator.There is a fixed mirror and a movable mirror in the cavity, the movable mirror coupled to the mechanical oscillator by opto-mechanical coupling.Resonance interaction occurs between spin and electromagnetic models restricted in the cavity.The Hamiltonian is given by (ħ = 1)

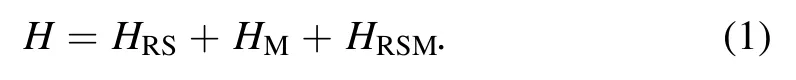
Here,

is the Rabi-Stark Hamiltonian.

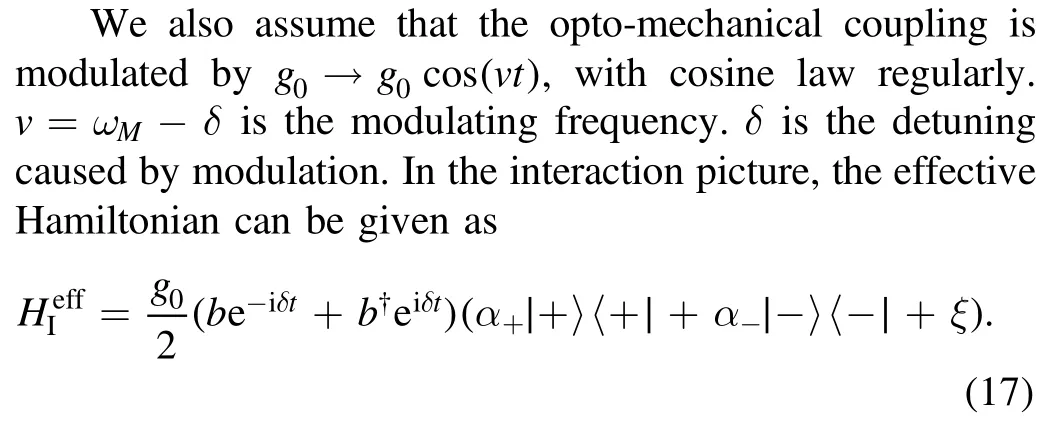
HRis the quantum Rabi Hamiltonian of the light–matter system written as

HStarkis the atom-cavity Stark interaction term with

γ a†aσ zis the nonlinear coupling term added to the coupling of the atom-cavity [14, 15].γis the non-linear Stark coupling strength.

is the Hamiltonian of the mechanical oscillator.b†(b) is the creation (annihilation) operator of the mechanical oscillator separately, with the free frequency of the springωM.And

is the opto-mechanical interaction term.g0denotes the vacuum opto-mechanical coupling.

ForHRin equation(3), a low-energy effective model was described [23].And there are three energy eigen states with lowest energies, which were given by using quasi degenerate perturbation theory,at second order inη.These eigenstates are:

And the revised ground state wave function and excited state wave function are respectively

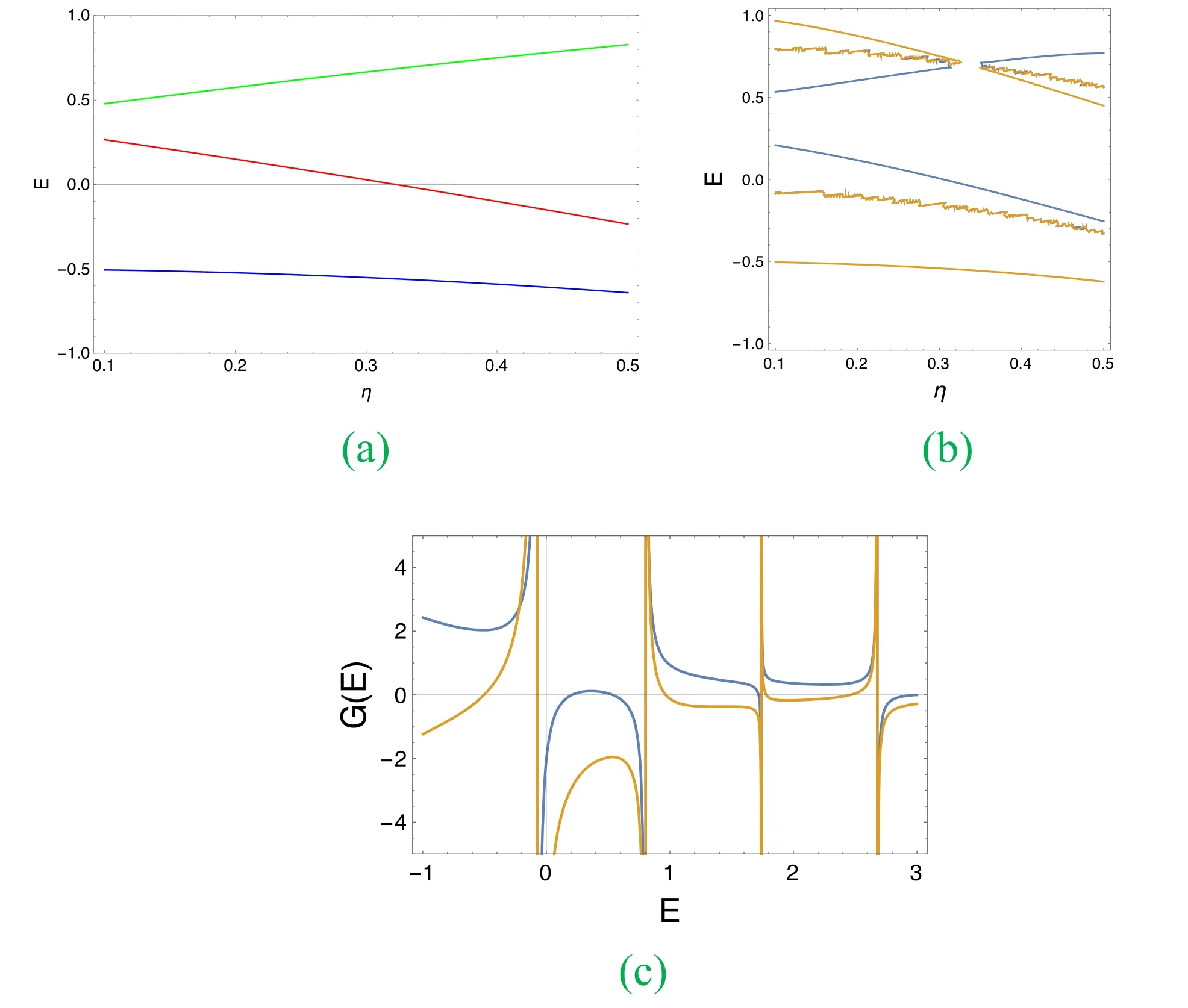
Figure 1.(a)The lowest three eigen energies of QRSM for γ = 0.25,η = 0.1.Green line and Red line are for E+ and E- respectively.The Blue line is forE 0.(b) Energy spectra deduced from [17] for γ = 0.25.The irregular broken lines are for the poles of the G-function.(c)G-curves for γ = 0.25,η = 0.1.

3.Macroscopic quantum superposition state of the mechanical oscillator

At timet= 0, we assume that the initial state of the whole system to be∣±〉 ⊗ ∣0m,where∣0〉mis the vacuum state of the mechanical oscillator.by applying the propagator associated withon the initial state, the evolution state of the whole system at timetin the interaction picture can be derived as

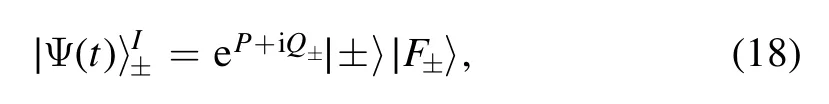
with

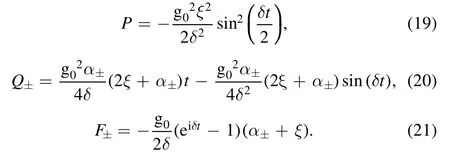
∣ 〉±Fare the coherent states of the mechanical oscillator.Transformingnto the Schrödinger picture,we can get the evolution stateof the total system in the Schrödinger picture as

with

and

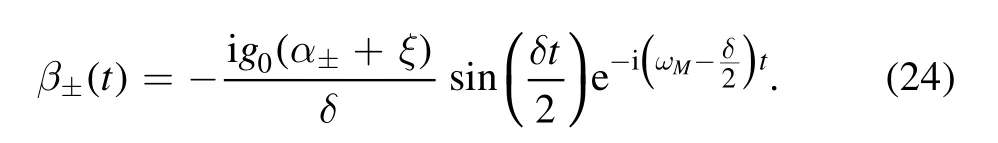
Now we assume that for timet= 0,the initial state of the atom-cavity to be∣0,e,and the mechanical oscillator to be in the state of∣0〉m,i.e., the initial state of the whole system is

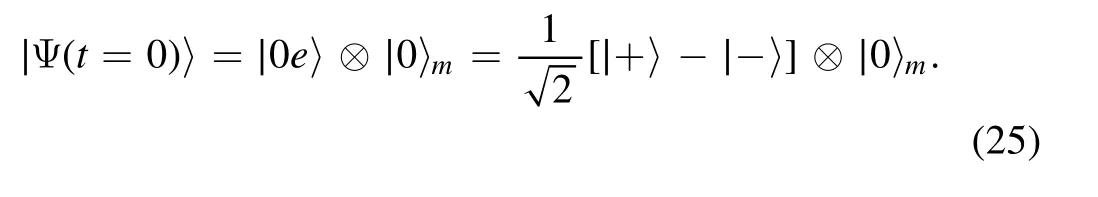
According to equation (22), the evolution state of the whole system at timetin the Schrödinger picture can be obtained as

Taking equation (13) into the equation (26), we can rewrite∣ Ψ(t) as

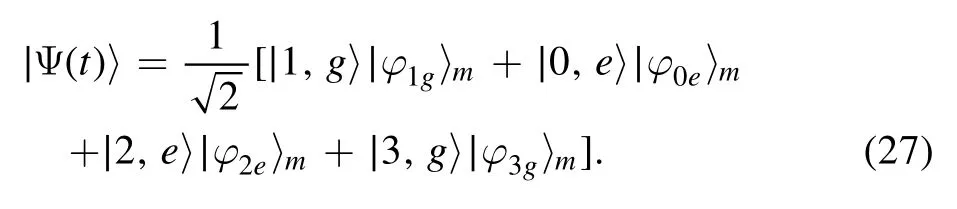
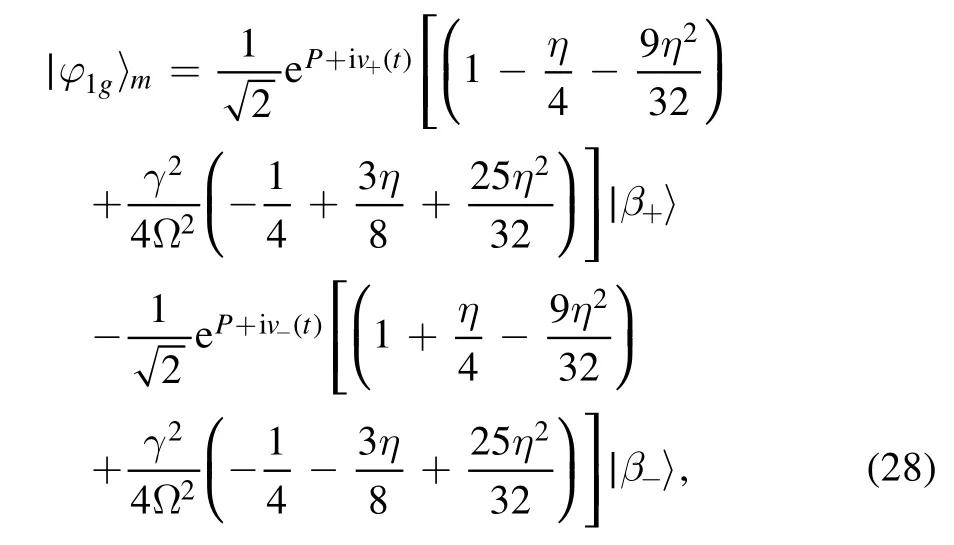
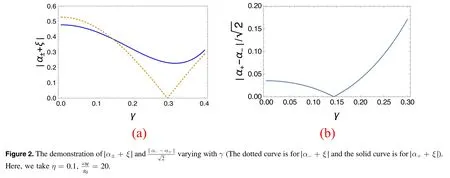
In figure 3, we give the demonstration of the evolution curves of〈β+∣β-〉 withtforγ= 0(which corresponds to the circumstances using the Rabi model [22]) andγ= 0.25.It can be seen from the two curves that the minimum of〈β+∣β-〉 forγ= 0.25 can get down to zero faster than the case forγ= 0,and sustaining zero for a much longer time,following which would improve the distinguishability of the macroscopically distinct coherent state in the Rabi-Stark model.

4.Wigner function

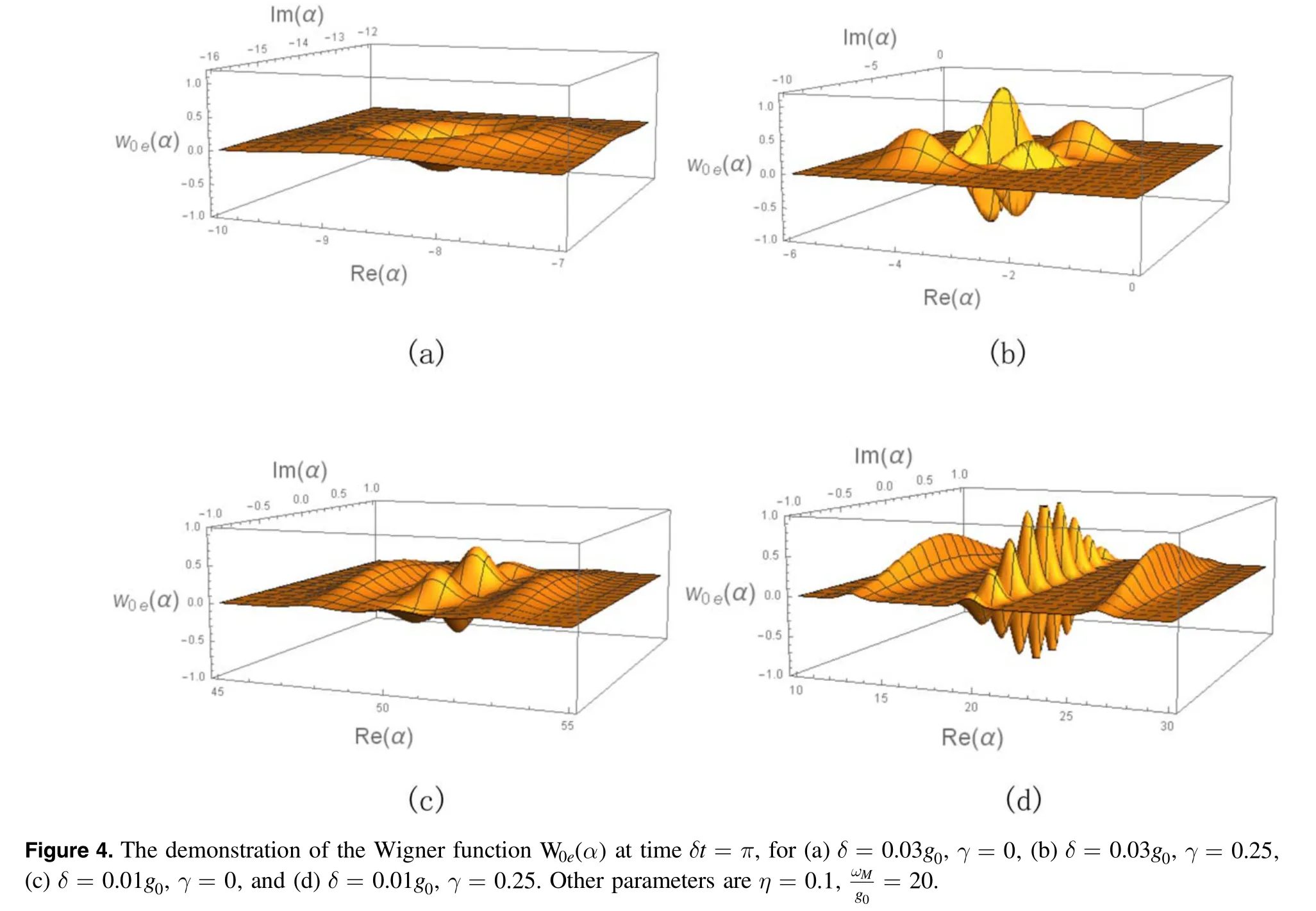
In figure 4, we give a demonstration ofW0e(α).Figures 4(a) and (b) are forγ= 0 andγ= 0.25 respectively withδ=0.03g0.It can be seen that in figure 4(a), the two

Compared with the QRM, we have shown that the Stark coupling can induce larger energy level differences between the dressed states.This movement of the energy levels can induce stronger coupling between the atom-cavity subsystem and the quantum mechanical oscillator, which would further enhance the generated macroscopic quantum coherence states to have a stronger quantum coherence effect, and therefore better able to be distinguished.coherent states wave packets are so close that it is difficult to distinguish them, and their coherence is very weak.While in figure 4(b), there is a large distinct and well coherence between the two states wave packets.

Also, figures 4(c) and (d) are for the demonstration ofW0e(α),with a smallerδ=0.01g0respectively forγ= 0 andγ=0.25.It can be seen that in figure 4(c), the two coherent states have slightly more obvious distinguishability and slightly stronger coherence than figure 4(a).This is due to the reduction of δ from0.03g0to0.01g0.While it can be seen that in figure 4(d), whenγincreases from 0 to 0.25, the distinguishability and the coherence between the two states will be significantly enhanced than figure 4(c).

5.Conclusion

In this article,we have proposed a method to further improve the preparation of macroscopically distinct quantum superposition state, by a Rabi-Stark coupling opto-mechanical system, compared with the scheme by a Rabi model.Firstly,in a low-energy subspace of the QRSM,we revised the eigen energies and eigen states of the QRM by using quasi degenerate perturbation theory, and then we got the effective Hamiltonian of the whole system.