Geometric quantities of lower doubly excited bound states of helium

Chengdong Zhou(周成栋) Yuewu Yu(余岳武) Sanjiang Yang(杨三江) and Haoxue Qiao(乔豪学)

1School of Physics and Technology,Wuhan University,Wuhan 430072,China

2College of Physics and Electronic Science,Hubei Normal University,Huangshi 435002,China

Keywords: doubly excited states,unnatural parity,Hylleraas-B-spline,geometric quantities

1. Introduction

The first evidence of existence of doubly excited states(DESs)is the observation of a line of 32.079 nm in the emission spectrum of helium by Comptom and Boyce.[1]Kruger attributed it to the transition between the 2p23Peand 1s2p3Postates two years later,[2]and it was confirmed by Wu soon.[3]The existence of DESs of helium has stimulated plenty of research. For example, Hilgeret al.using the Hylleraas basis presented precise energy levels of1,3Pestates of helium below the second ionization threshold of He+.[4]Using the complex-rotation method,Ho and Bhatia calculated resonance parameters of1,3Peand1,3Dostates of helium-like systems below theN= 3-5 threshold of He+.[5,6]These3Pestates were also calculated by Saha and Mukherjee using the stabilization method.[7]However, there are some discrepancies between the results presented by Sahaet al.[7]and by Hilgeret al.[4]and Hoet al.[5]To make the reason of discrepancies clear,Kar and Ho[8]recalculated resonance parameters of3Pestates of helium using both complex-rotation method and stabilization method with correlated exponential wavefunctions.Their results are consistent with Hilgeret al.[4]and Hoet al.[5]Using spectral approach,Eilglsperger presented a wide range of energy levels for meta-stable bound states1,3Lwith total angular momentumL=1-9,and of resonance parameters for resonance1,3Pestates belowN=3-5 threshold of He+.[9,10]

Besides the resonance parameters, geometric quantities are also important physical quantities describing information of states, such as〈r〉,〈r12〉,〈r＜〉,〈r＞〉,〈cosθ12〉and〈θ12〉,which represent electron-nucleus radial distance, interelectronic radial distance, radial distance between inner electron and nucleus, radial distance between outer electron and nucleus, cosine of interelectronic angle and interelectronic angle,respectively. The〈r＞〉could be used to estimate the resonance general size and to distinguish the difference resonance states series of H-.[11,12]In addition,〈r＜〉,〈r＞〉and〈cosθ12〉could be used to construct the Lewis structures for resonance states.[13]Angular quantities〈θ12〉,〈cosθ12〉could be used to study the resonance parameters of plasma-embedded helium.[14]The interference phenomena between difference resonance series could also be described by these geometic quantities.[13]Systemically investigations on expectation values of these geometric quantities for ground and singly excited states of two-electron systems was completed based on the HF and MCHF methods by Koga group,[15-18]and based on full STO-CI framework and Hylleraas-CI method by Jiao.[19,20]Kogaet al.also pointed out that for singlet and triplet singly excited states,〈r〉and〈r12〉could be fitted into nearly linear law formulas.[18]However, to our best knowledge, the works focusing on geometric quantities of DESs of two-electron systems are relatively rare. In this work, we calculate the geometric quantities of1,3Peand1,3Dostates of helium based on Hylleraas configuration interaction approach using the Hylleraas-B-spline(H-B-spline)basis. These states are doubly excited, which indicates that the wavefunctions distribute in a wider radial range. The variational basis should have good fitting abilities. On another hand, precise calculations on lower lying states needs large partialwave expansion. Recently, Yanget al.introduced a convenient basis set,called the H-B-spline basis,by integrating ther12=|r1-r2|factor in theB-spline basis.[21]This basis inherits the virtue of traditionalB-spline basis,the high localization of each spline, and also has the ability of describing the behavior of wavefunctions at two-electron coalescences, which improves greatly the rate of convergence in partial-wave expansion. The H-B-spline basis was successfully applied to calculation on polarizabilities, magic wavelengths and Bethe logarithm of helium.[21-23]Here we will use this basis to calculate DESs, 2pnp1Pe(3≤n ≤5),2pnp3Pe(2≤n ≤5)and 2pnd1,3Do(3≤n ≤5),of helium.

This article is organized as follows. Section 2 includes a brief introduction of the H-B-spline basis and the computational methodology in calculating geometric quantities. Convergence studies of energy levels and geometric quantities of 2pnp1Pe(3≤n ≤5),2pnp3Pe(2≤n ≤5) and 2pnd1,3Do(3≤n ≤5)states are presented in Section 3. Discussion are made in this section as well. Finally, a summary is given in Section 4. Atomic units are used throughout this article.

2. Theoretical method

2.1. Hylleraas-B-spine basis

The nonrelativistic Hamiltonian of helium in center-ofmass frame after eliminating system global motion is given by

Hereqandkare summation indexes which rank from zero toc/2 and zero toc/2-qfor evencor from zero to infinity and zero to(c+1)/2 for oddc;tis continued product index,Sqc=min(q-1,(c+1)/2)is the upper limit value of continued product, andG1represents angular integral parts which are reduced from the summation of Wigner 3jsymbol,

Herebis equal tol2+1 whenT1=l2-1,or equal to-l2whenT2=l2+1,T1andT2are summation indexes, and (T1,T2) is brief denotation of(2T1+1)(2T2+1).

2.2. Geometric quantities

The evaluation of quantities〈r〉,〈r12〉,〈r＜〉,〈r＞〉,〈cosθ12〉and〈θ12〉could be dealt by including corresponding term intoOIJ. For angular quantities〈θ12〉,the multipole expansion method is adopted to investigate its expectation values

This angular integral part is similar to Eq. (10), which just needs to adjust the number 1 tokin all Wigner 3jand 6jsymbols.

3. Results and discussions

3.1. Energy levels

Table 1 shows a convergence study of energy level for doubly excited 2p23Pestate of helium with increasing total number ofB-splineNand partial-wave expansion lengthlmax.As shown in this table, the results of this state converge toE=-0.7105001556 whenlmax=4. The extrapolated energy level of this state isE=-0.71050015567(1), which has 11 significant digits and are consistent with precise valuesE=-0.7105001556783 andE=-0.71050015567833 obtained from the exponential correlated basis[25]and the extensive Hylleraas-CI method.[4]

Table 1. Convergence study of the energy level for the 2p23Pe state of helium as the total number of B-splines N and the partial-wave expansion length lmax increase. The numbers in parentheses of the extrapolated values are the computational uncertainties. Here all the data are in units of a.u.

Table 2 displays our extrapolated energy levels for 2pnp1Pe(3≤n ≤5) and 2pnp3Pe(2≤n ≤5) states. Comparisons with other precise results are available. Reference data a come from the results of Eiglspergeret al.calculated with Coulomb-Sturmian basis CI method.[9]Reference data b represent results from Kar and Ho using exponential correlated wave functions.[25]Reference data c are obtained by Hilger using extensive Hylleraas-CI calculation.[4]Our results are more consistent with reference data c which are the most accurate known results for these nonautoionization states. Compared to reference data a, Our results have 2 significant digits more precise for 2pnp1Pewithn=3,4,and 4 significant digits for 2p4p3Pestates.

Table 2. Extrapolated energy levels of doubly excited bound 2pnp1Pe(3 ≤n ≤5)and 2pnp3Pe(2 ≤n ≤5)states of helium in comparison with reference data. The numbers in parentheses of the extrapolated values are the computational uncertainties. Here all the data are in units of a.u.

Table 3 displays a comparison of energy levels for 2pnd1,3Do(3≤n ≤5) states. In this table, reference data d come from results of Bhattacharyya calculated with an extended Hylleraas basis.[26]For these 2pnd1,3Do(3≤n ≤5)states, our results are more consistent with reference data b,the most precise known results of these energy levels. Our results have at least 2 significant digits more accurate than reference data a and d for all 2pnd1,3Do(3≤n ≤5)states. These results indicate that for higher doubly excited bound states,precise energy levels could be acquired using the H-B-spline basis without too large increase of total number ofB-splineN. As we can see,the energy levels for higher excited states,either for1,3Peor1,3Dostates,have more convergency significant digits than lower excited states,mainly due to the knots ofB-spline functions we choose to make the H-B-spline basis more appropriate to fit wavefunctions of higher excited states,which distribute in a broader radial range, and the interelectronic correlated effect is weak. The results also provide the precise wavefunctions for the following calculations.

Table 3. Extrapolated energy levels of doubly excited bound 2pnd1,3De(3 ≤n ≤5)states of helium in comparison with reference data. The numbers in parentheses of the extrapolated values are the computational uncertainties. Here all the data are in units of a.u.

3.2. Geometric quantities

The H-B-spline basis has shown its well capacity in calculation on energy levels for lower lying doubly excited bound states of helium. Here we use this basis to calculate the expectation values of geometric quantities〈r〉,〈r12〉,〈r＜〉,〈r＞〉,〈cosθ12〉and〈θ12〉in detail.As mentioned in Section 2,the investigation on〈θ12〉is carried out by multipole expansion. Table 4 presents the contributions of the first 17 order expansion terms to the expectation value〈θ12〉for 2p23Pestate under total number ofB-splineN=50 and partial-wave expansion lengthlmax=4. As displayed in this table, the contribution of〈Pk(cosθ12)〉reaches the magnitude of 10-9atk=17,and the changes of values of〈θ12〉are quite small. We truncate the summation(11)tokmax=17 in our calculations and extrapolate these values to give the computational uncertainties.

Table 4. Contributions of the first 17 order expansion terms to the interelectronic angle expectation value 〈θ12〉 for doubly excited bound 2p23Pe state of helium. Here all the data are in units of a.u.

Table 5. Expectation values of radial geometric quantities for doubly excited bound 2pnp1Pe(3 ≤n ≤5),2pnp3Pe(2 ≤n ≤5) and 2pnd1,3Do(3 ≤n ≤5) states of helium. The numbers in parentheses of the extrapolated values are the computational uncertainties. Here all the data are in units of a.u.

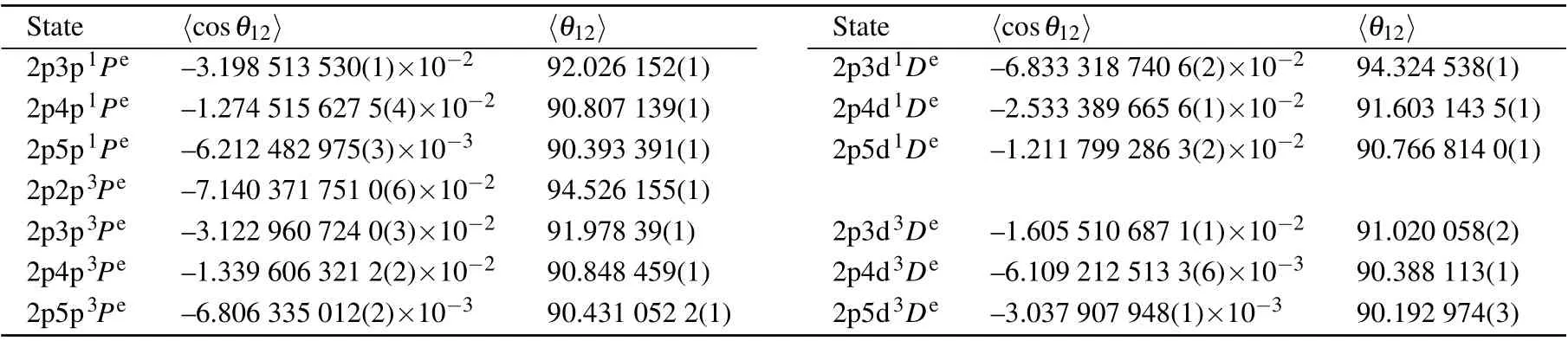
Table 6. Expectation values of angular geometric quantities for doubly excited bound 2pnp1Pe(3 ≤n ≤5),2pnp3Pe(2 ≤n ≤5) and 2pnd1,3Do(3 ≤n ≤5) states of helium. The numbers in parentheses of the extrapolated values are the computational uncertainties. Here all the data are in units of a.u.

4. Summary

In this article, the Hylleraas-B-spline basis is imported into the calculation of energy levels for doubly excited 2pnp1Pe(3≤n ≤5), 2pnp3Pe(2≤n ≤5) and 2pnd1,3Do(3≤n ≤5) states of helium. Good agreement is achieved between our results and the precise values obtained by Kar and Ho[25]and Hilgeret al.[4]Geometric quantities〈r＜〉,〈r＞〉,〈r〉,〈r12〉,〈cosθ12〉and〈θ12〉of these states are investigated in detail as well and precise results are reported.Our results of these geometric quantities provide a precise reference for future studies.These calculations have shown the good capacity of the H-B-spline basis in calculation on lower lying doubly excited bound states. It would be appealing to an extend H-B-spline basis to the calculations of doubly excited resonance states of helium accompanied with stabilization and complex-rotation method.

Acknowledgements

Project supported by the National Natural Science Foundation of China (Grant No. 12074295). The authors thank Yongbo Tang for the meaningful discussion. The numerical calculations in this article were performed on the supercomputing system in the Supercomputing Center of Wuhan University.