基于响应面法的碳管纳米流体稳定性

闫素英，张田歌，袁 雪，高 虹，张慧颖，赵晓燕

基于响应面法的碳管纳米流体稳定性

闫素英1,2，张田歌1，袁 雪1，高 虹1，张慧颖1，赵晓燕1

（1.内蒙古工业大学能源与动力工程学院，呼和浩特 010051；2. 内蒙古自治区太阳能高效转化及综合利用重点实验室，呼和浩特 010051）

为了获得稳定性更好的纳米流体，基于响应面法对添加优选后分散剂的碳管纳米流体进行了稳定性的优化。通过“两步法”制备碳管纳米流体，比较了4种不同类型的表面活性剂对碳管纳米流体稳定性和导热性的影响。以碳管纳米流体的粒径为评价指标，选取碳管纳米流体质量分数、超声振荡时间、放置时间3个影响因素，设计了三因素三水平Box-Behnken试验，利用响应面法考察了各因素对纳米流体稳定性交互作用的影响。结果表明，含有十二烷基甜菜碱Dodecyl Betaine分散剂的碳管纳米流体，在碳管与分散剂比例为1∶2时综合性能最好。试验数据建立的二次多项数学模型显著性极高（<0.000 1），相关系数2=0.964 4，预测值与实际值具有很好的拟合度。通过二次元回归模型得到影响碳管纳米流体稳定性因素的条件为：碳管纳米流体质量分数0.27%，超声时间83.45 min，放置时间8 h。在此条件下的粒径平均值为121.58 nm，与预测值120.60 nm有0.016%的误差，说明此模型优化碳管纳米流体稳定性是可行的。

纳米流体；稳定性；导热性；分散剂；响应面法

0 引 言

纳米流体作为新型的高导热传热介质[1-3]，已经被广泛的应用于各个领域中。其性能受到众多因素的影响。纳米流体稳定性可通过加入分散剂[4]、调节纳米颗粒浓度[5]、超声时间处理[6]等来改善。故制备出稳定的纳米流体是纳米流体能否广泛应用的关键因素。

目前，大量学者对纳米流体的稳定性进行了研究。Iwaa等[7]研究比较了3种不同类型分散剂对纳米流体稳定性和热物性的影响。Zareei等[8]研究了3种表面活性剂对氧化铝纳米流体的改善程度，但未涉及分散剂与纳米流体用量的最佳比例。传统的正交优化设计方法采用线性数学模型进行试验设计，具有精度不高、预测性能不佳的缺点，而响应面法采用非线性模型对试验数据进行高精度的回归拟合，可以有效地安排和优化试验。Esfe等[9]通过响应面法对纳米流体的热物性进行了优化，使其粘度和导热系数达到最优值。Danish等[10]采用响应面法研究了不同因素对二氧化钛水纳米流体粘度和导热系数的影响，并对建立的模型进行了验证试验。Abdulrahman等[11]采用基于多变量设计的响应面法，建立了优化铜纳米流体粘度的可行方案，可以较为准确预测铜纳米流体粘度的变化。Esfe等[12]利用杂项设计模型进行响应面法研究了不同因素对纳米流体热物性的影响，预测值与试验数据基本一致。但是使用响应面法对纳米流体稳定性优化研究较少，并且对纳米流体粒径的优化还未涉及。

本文通过“两步法”制备碳管纳米流体，对4种不同表面活性剂进行了优选，以粒径大小为评价指标，考察了各因素对碳管纳米流体稳定性的影响规律，确定了影响碳管纳米流体稳定性的最佳条件。旨在探讨提高纳米流体的稳定性，为后续纳米流体的制备提供理论依据及试验基础。

1 试验材料和方法

1.1 试验材料及仪器设备

材料：碳纳米管（Carbon Nanotubes，CNTs）纳米颗粒，黑色粉状，纯度≥99.9%，外径>50 nm，平均粒度30 nm，比表面积>60 m2/g，生产厂家为上海超威纳米科技有限公司。表面活性剂的详细参数和试验设备如表1。

表1 表面活性剂参数

仪器设备：高速剪切机（上海贝尔特B25）；超声分散仪（豫明仪器YM-1200Y）；马尔文激光粒度仪（马尔文公司）；紫外可见近红外分光光度计（精度±3%，日本岛津UV3600）；热常数分析仪（精度±3%，TPS2500S，Hot Disk AB公司）。

1.2 纳米流体的制备过程

本文采用两步法[13]合成碳管纳米流体，将碳管纳米粉末分散到蒸馏水中，然后通过高速剪切机将碳管纳米流体剪切10 min进一步分散。最后添加分散剂并利用超声分散仪对碳管纳米流体悬浮液进行了不同超声时间（10、60、110 min）的超声振动控制[14]，超声功率可调区间为10～1 200 W，考虑到功率太小超声时间较长，功率太大溶液温度过高，所以综合考虑超声功率采取800 W。振荡结束后取出试管，完成碳管纳米流体的制备。

1.3 纳米流体稳定性评价方法

目前对于纳米流体稳定性的评价方法有很多种，本文综合采用粒度法、Zeta电位法、吸光度法对不同分散剂进行了优选[15-17]。该方法能提高评定分散剂对纳米流体稳定性的准确性。

2 响应面试验设计及模型验证

响应面法（Response Surface Method，RSM），探究试验变量和指标的相关性，通过建立响应面图形并对其进行回归拟合[18]，可以分析每个影响因素及其相互作用的显著性，Box-Behnken模型作为RSM中的一个模型，适合多因素间交互作用的研究[19]。

2.1 试验设计和结果

根据分散剂优选的试验结果，选取DB-CNTs纳米流体的质量分数1、超声振荡时间2、放置时间3为自变量，以碳管纳米流体粒径(nm)为响应值。根据Box-Behnken模型，设计三因素三水平响应面分析，水平范围质量分数选取0.1%～0.5%，超声振荡时间选取10～110 min，放置时间选取0～10 d，因素与水平编码见表2。

表2 因素水平编码值表

根据表2各因素水平的编码值，设计了17组试验，其中14组为分析试验，5组为中心试验，用于试验误差的估计。采用Design-Expert软件分析，建立响应面模型，试验设计及结果见表3。

通过响应面试验数据进行二次多元回归拟合，得到粒径（）与碳管纳米流体质量分数（1）、超声振荡时间（2）、放置时间（3）二次回归方程式为粒径（）与碳管纳米流体质量分数（1）、超声振荡时间（2）、放置时间（3）二次回归方程式为

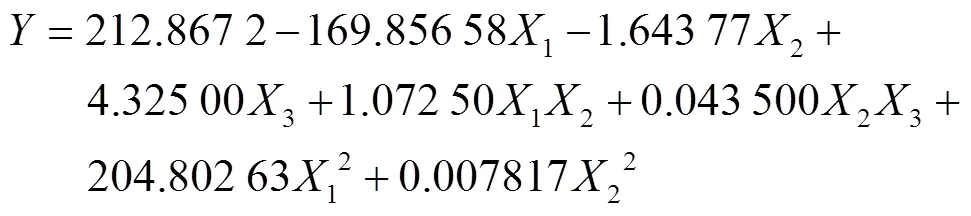
表3 响应面试验设计及结果

2.2 模型的充分性检验

图1所示的正态概率图进一步测试了所建立的模型，由图可知，所有检查参数的残差在正态概率图上大致呈直线，且没有遵循任何序列或趋势，这表明残差是正态且独立分布的，试验值合理且均匀[20]。

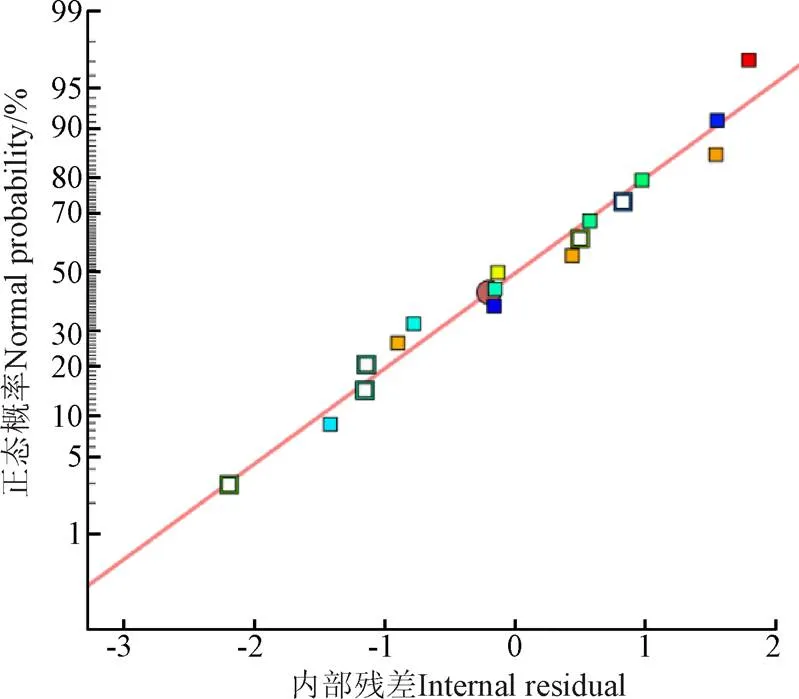
图1 内部残差的正态概率图

图2为离群点图被用来检查任何异常的数据点，如果数据点超过了红线允许的范围，本文取±3，那么这些数据点被认为是异常[10]。由图可知，该模型所有的点都在允许的范围内且分布散乱无规则，则表明模型是可靠的[21]。

图3将粒径的试验结果与模型预测的数据进行了比较，由图可知，实际值与预测值呈现正相关性，在斜率为1的直线两侧分布较为均匀，模型和试验数据之间有很好的一致性。因此使用统计方法得到的试验结果与预测结果之间有很好的相关性。

图2 离群点图

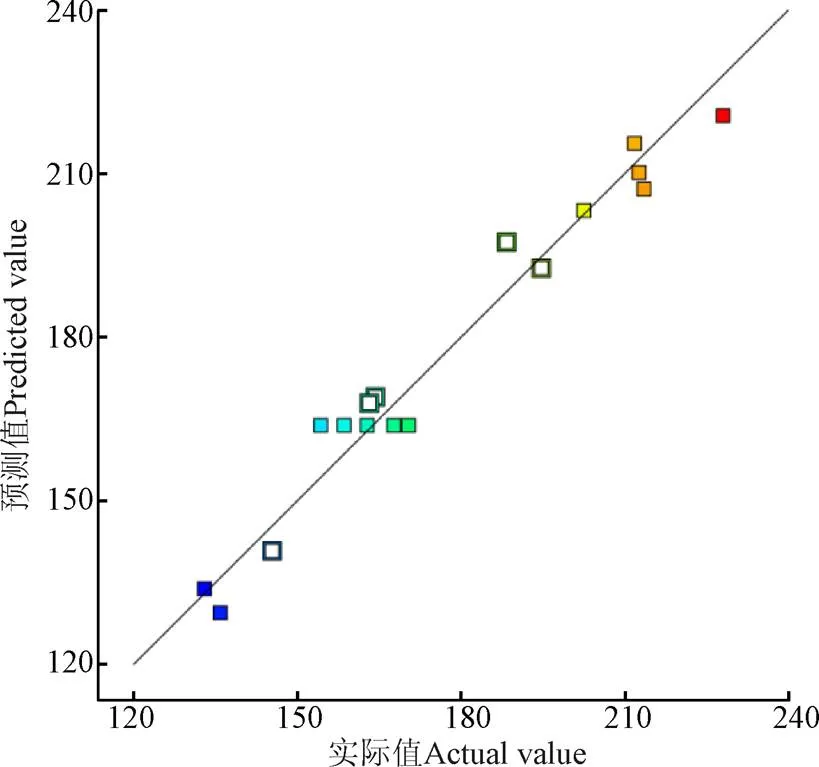
图3 预测值与实际值对比图

2.3 模型方差分析

为进一步确定1、2、3这3个因素对纳米流体粒径的影响程度，对回归模型给出的方差进行分析，结果见表4。概率值越小，相应变量的显著程度越高[22]。在本试验中，计算值为34.9，值小于0.000 1，说明所得模型具有很高的显著性。其校正系数2=0.964 4，模型拟合度好。此外失拟项值为1.46，值为0.366 5大于0.05，说明模型的有效性无统计学意义。因此，该回归方程的模型成立。

由上表4可知，在一次项中，放置时间3的影响最大（<0.000 1），达到极显著水平，超声时间2达到显著水平（=0.010 8<0.05）。在二次项中CNTs纳米流体质量分数12达到显著水平（=0.047 7<0.05），超声振荡时间22达到极显著水平（=0.000 4<0.01）。在交互项中，CNTs纳米流体质量分数和超声时间的交互项12达到显著水平（=0.017 1<0.05），超声时间和放置时间的交互项23达到显著水平（=0.016 0<0.05）。而13、32对碳管纳米流体粒径的影响均不显著（>0.05）。由碳管纳米流体粒径可知，3个影响因素（见表2）对纳米流体粒径的主次因素为：3>2>1。

表4 回归模型方差分析结果

注：<0.05，差异显著；<0.01，差异极显著。

Note:<0.05, the difference was significant;<0.01, the difference was significant.

3 结果与讨论

3.1 分散剂对纳米流体稳定性的影响及优选

3.1.1 粒径

图4显示了CNTs与分散剂在5种配比和5种放置时间下纳米流体平均粒径的试验结果。由图可知，DB-CNTs、CTAC-CNTs、Tx-100-CNTs和SDS-CNTs纳米流体随着分散比例的增加呈现先减小后增大的趋势，并分别在比例为1∶2，1∶2，1∶1，1∶2时粒径呈现最小值，这是因为此时纳米颗粒表面提供分散剂吸附的面积已经饱和，纳米流体分散稳定性较好，可以看出添加最佳添加量的分散剂可以提高纳米流体的稳定性[23-24]。

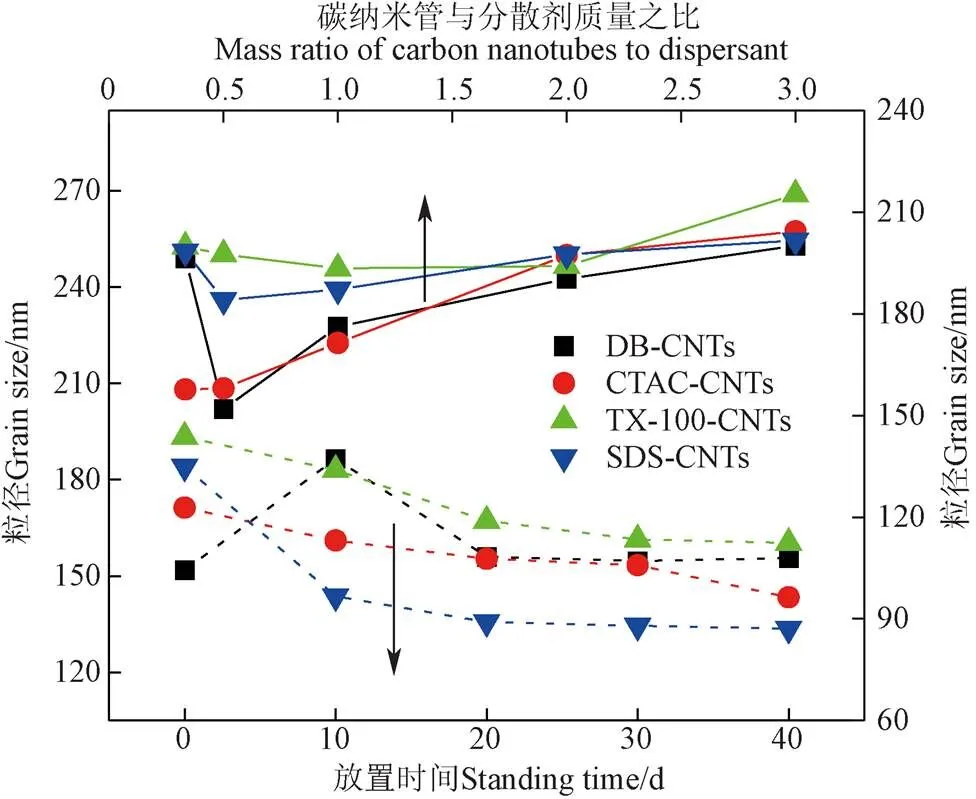
注：DB-CNTs等表示添加DB分散剂的碳管纳米流体，其中前缀表示添加的分散剂种类，后缀表示碳管纳米流体，下同。其中实线对应右纵坐标，虚线对应左纵坐标。

由图4可知，DB纳米流体放置10 d粒径发生较大的增大，可能是由于悬浮液中出现团聚现象，粒子体积增大，悬浮液中大体积的颗粒发生沉降现象，之后逐渐变小后趋于平缓，CTAC-CNTs、Tx-100-CNTs和SDS-CNTs纳米流体的粒径逐渐减小并趋于平缓。

3.1.2 Zeta电位

图5显示了不同分散剂含量及放置时间对CNTs纳米流体Zeta电位的影响。由图可知，随着表面活性剂比例的增加，含有SDS和CTAC的纳米流体产生更高的值，在比例为1:2时产生最大电位绝对值分别为40.4和28.4 mV，Zeta电位绝对值越大，说明此时纳米流体分散体系越稳定。含有DB纳米流体的Zeta值较小，整体在−20 mV附近波动。

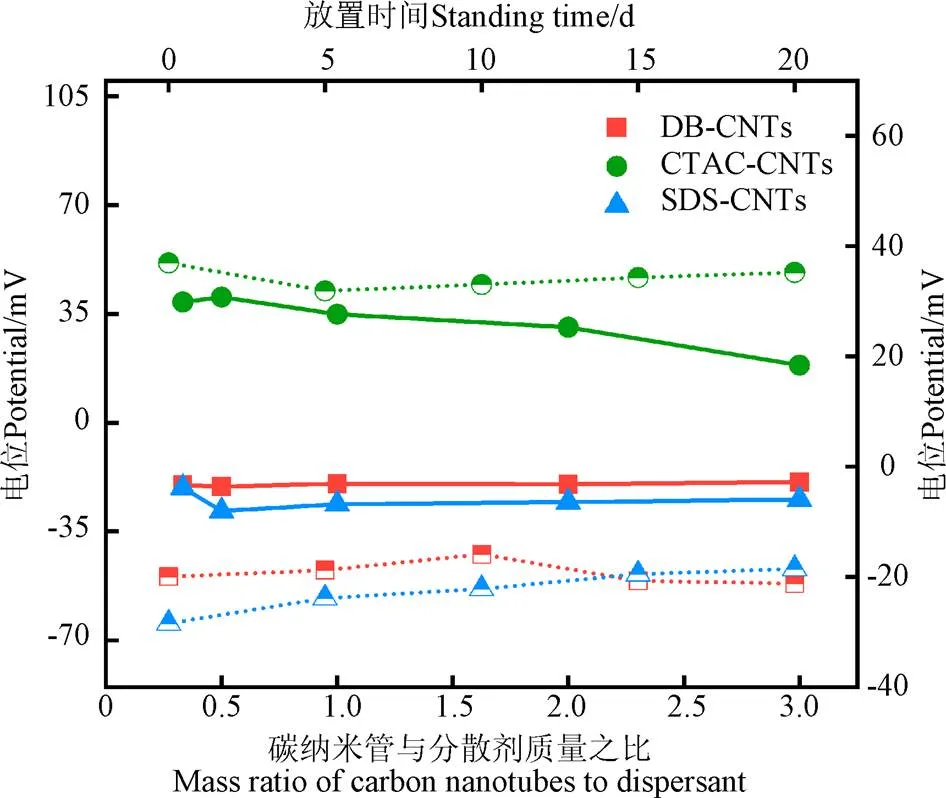
注：实线为分散剂比对应左纵坐标。虚线为放置时间对应右纵坐标。

由图5可知，DB-CNTs纳米流体的Zeta电位绝对值呈先减小后增大的趋势，在放置10 d取得最小电位绝对值15.6 mV。SDS-CNTs纳米流体电位负值一直呈现减小的趋势，说明稳定性不断下降。CTAC-CNTs纳米流体的Zeta电位变化趋势不明显。

3.1.3 吸光度

图6显示了分散在的水中添加不同比例分散剂的CNTs的UV-vis光谱。通过分光光度法[25]比较分散的CNTs在波长为301 nm的光谱。

图6 不同分散剂比例对吸光度的变化曲线（波长301 nm）

可以看到使用DB表面活性剂的纳米流体在比例为1∶2时具有最高的吸光度，接着是具有CTAC的纳米流体，然后是SDS的纳米流体，最后是Tx-100。这是由于两性表面活性剂DB对碳基纳米材料的吸附高于其它离子表面活性剂，两性表面活性剂分子的一头带负电荷和另一头带的阳离子电荷之间存在强静电相互作用[26]，使得纳米流体具有良好的分散性。

3.2 分散剂对导热性能的影响

图7显示了添加不同比例分散剂下碳管纳米流体的导热系数。由图可知，4种纳米流体随着分散比例的增加，导热系数均是先增大后减少的趋势，含有分散剂DB的碳管纳米流体具有最高的热导率。DB-CNTs，CTAC-CNTs，Tx-100-CNTs和SDS-CNTs 4种纳米流体的比例分别为1∶2，1∶1，1∶2，1∶2时导热系数呈现最大值，导热系数分别为0.687 9、0.682 3、0.675 1和0.674 7 W/(m·K)。

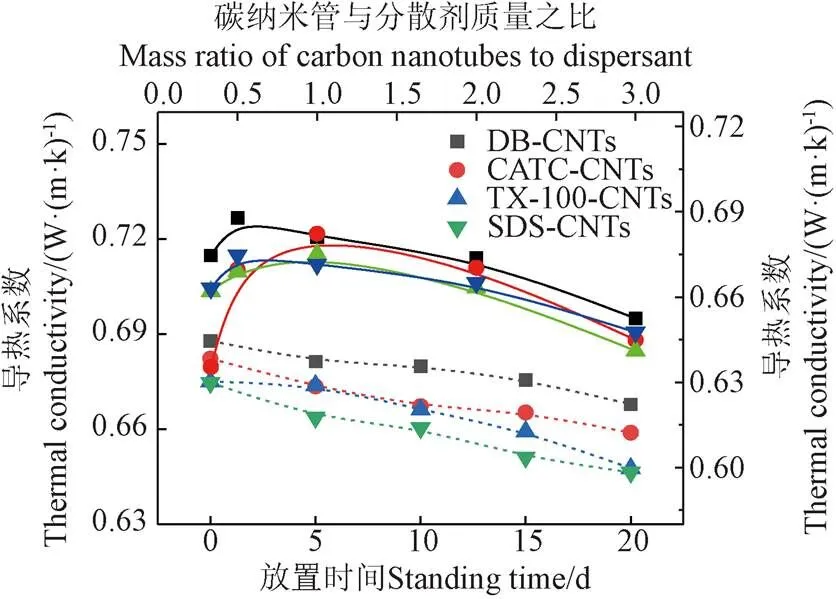
注：实线分散剂比对应右纵坐标。虚线放置时间对应左纵坐标。

由图7可知，DB-CNTs、CTAC-CNTs、Tx-100-CNTs、SDS-CNTs纳米流体导热系数随着放置时间得到了明显的变化，几乎呈线性下降，下降速率较快的是SDS-CNTs。

通过使用电位、粒径、吸光度和导热系数来评价分散剂-CNTs流体的整体性能，结果表明，与其他3种分散剂相比，含有DB分散剂的CNTs纳米流体，在碳管与分散剂比例为1∶2时的粒径最小、吸光度最高、导热系数最高，被认为是碳管纳米流体稳定的最佳比例。因此选用DB分散剂去研究CNTs纳米流体的性能。

3.3 碳管纳米流体稳定性的响应面分析

通过等高线图的形状可以得出各影响因素交互作用的大小，椭圆形代表着交互效应明显，而圆形则代表两者交互作用不明显[27]。而响应曲面变化的越快表示坡度越大，即对试验结果的影响更为显著。

3.3.1 质量分数与超声时间

图8为碳管纳米流体质量分数与超声时间两因素交互作用的等高线图。由图8可知，粒径随着质量分数和超声时间的增加均呈现先增大后减小的趋势，说明质量分数过大反而不利于碳管纳米流体的稳定性提高[28]，而超声时间过长，纳米粒子在超声比作用下剧烈运动，导致已经均匀分散的纳米颗粒剧烈碰撞凝聚使得体积变大，从而产生沉淀使得稳定性变差[29-30]。等高线图呈现椭圆形，说明两者的交互作用明显。

图8 质量分数与超声时间的等高线图

3.3.2 超声时间与放置时间

图9为超声振荡时间与放置时间两因素交互作用的等高线图。由图9可知，粒径随着超声振荡时间的增加呈现先减小后增大的趋势，随放置时间的增加而增加。粒径值在超声振荡时间为83.45 min左右较小，这是因为超声振荡使溶液的温度升高，加速了纳米颗粒的分子热运动，从而有利于纳米颗粒克服重力在溶液中稳定悬浮[31]。等高线不呈椭圆形，说明两者的交互作用不明显。

图9 超声时间与放置时间的等高线图

3.3.3 质量分数与放置时间

图10为质量分数与放置时间两因素交互作用的等高线图。由图10可知，粒径随着质量分数的增加呈先减小后增大的趋势，随放置时间的增加而增加。当碳管纳米流体质量分数过大时，碳纳米管间相互缠绕的几率变大，从而导致纳米流体整体粘度的增加，使得溶液稳定性变差。等高线不呈椭圆形状，说明两者的交互作用不明显。以上结论与方差分析一致，验证了与试验模型的拟合结果。

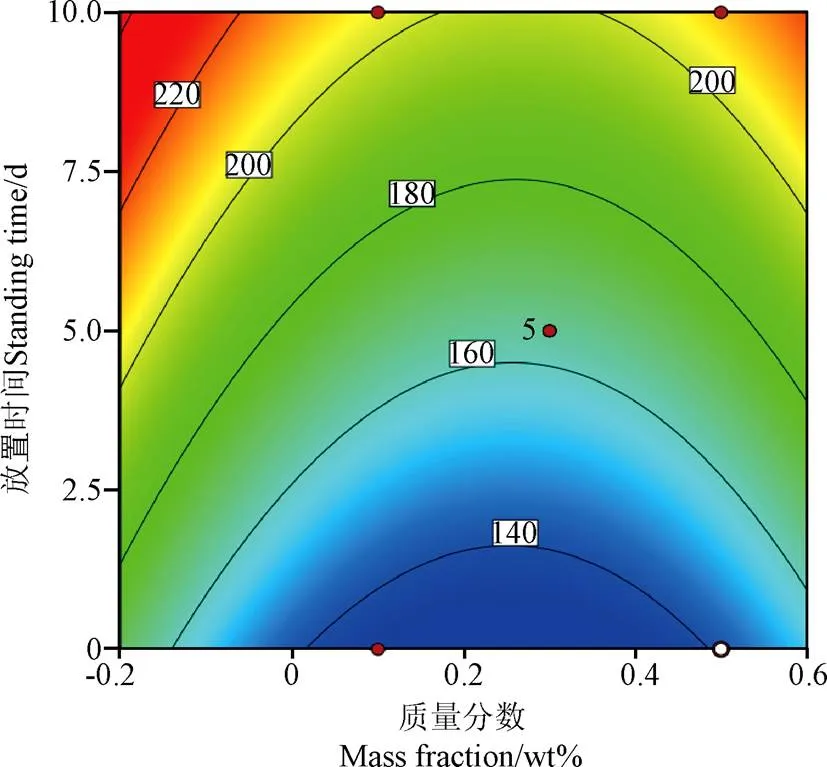
图10 质量分数与放置时间时间的等高线图

3.4 优化与验证

由响应面优化粒径所得最佳影响粒径的条件为DB-CNTs质量分数为0.27%，超声振荡时间为83.45 min，放置时间为8 h，粒径值为121.60 nm。采用最佳条件进行了3次验证试验，所得到的粒径分别为121.57、121.55、121.62 nm，所得平均值为121.58 nm，与理论值预测偏差0.016%，实际值与预测值接近，具有一定的参考价值。通过响应面试验分析可知，在后续的纳米流体制备中，尽量选好超声振荡时间和放置时间，以提高纳米流体的稳定性。

4 结 论

本文针对纳米流体稳定性问题，筛选出合适的分散剂，利用响应面模型与试验数据相结合的方法对纳米流体的稳定性进行了分析优化，主要结论如下：

1）通过试验对比分析了分散剂对碳管纳米流体稳定性和导热性能的影响。结果表明，与其他3种分散剂相比，含有DB分散剂的CNTs纳米流体，在碳管与分散剂比例为1:2时的粒径最小、吸光度最高、导热系数最高，因此选用DB分散剂去研究CNTs纳米流体的性能。

2）基于Box-Behnken试验设计方法，获得了能准确描述碳管纳米流体粒径值的响应面模型，同时获得响应值粒径（）与碳管纳米流体质量分数（1）、超声振荡时间（2）、放置时间（3）的二次多项回归方程。

3）通过显著性分析可知，对于碳管纳米流体粒径而言，3个因素对其影响的显著程度为：放置时间>超声振荡时间>CNTs纳米流体质量分数。其中CNTs纳米流体质量分数与超声振荡时间交互作用明显，超声振荡时间与放置时间的交互作用不明显，CNTs纳米流体质量分数与放置时间的交互作用不明显。

4）在试验的基础上，设计了三因素三水平响应面，得到最小粒径下各影响因素的条件：CNTs质量分数0.27%、超声振荡时间83.45 min、放置时间8 h，预测粒径值121.60 nm与实际平均值121.58 nm偏差0.016%。本文的研究为后续提高纳米流体稳定性提供理论依据和试验基础。

[1] Mehdi B, Saeed H. Electronics cooling with nanofluids: A critical review[J]. Energy Conversion and Management, 2018, 172: 438-456.

[2] 李龙，王江，翟玉玲，等. CuO-ZnO混合纳米流体导热系数影响分析[J]. 工业加热，2019，48(3)：38-40.

Li Long, Wang Jiang, Zhai Yuling, et al. Analysis ofthermal conductivity of CuO-ZnO nanofluids in ethyleneglycol/water mixture[J]. Industrial Heating, 2019, 48(3): 38-40. (in Chinese with English abstract)

[3] Taghizadeh A, Taghizadeh M, Azimi M, et al. Influence of cerium oxide nanoparticles on thermalconductivity of antifreeze[J]. Journal of Thermal Analysisand Calorimetry, 2020, 139(4): 225-236.

[4] 李栋，吴洋洋，刘昌宇，等. 分散剂对Al2O3-paraffin纳米流体吸收太阳能的影响[J]. 工程热物理学报，2019，40(7)：5.

Li Dong, Wu Yangyang, Liu Changyu, et al. Effect of surfactant on solar energy absorption of Al2O3-paraffin nanofluids[J]. Journal of engineering thermophysics, 2019, 40(7): 5. (in Chinese with English abstract)

[5] Xla C, Wc B, Cz B. The stability, viscosity and thermal conductivity of carbon nanotubes nanofluids with high particle concentration: A surface modification approach[J]. Powder Technology, 2020, 361: 957-967.

[6] Urmi W T, Rahman M M, Kadirgama K, et al. An overview on synthesis stability opportunities and challenges of nanofluids[J]. Materials Today: Proceedings, 2021, 41: 30-37.

[7] Iwaa B, Adma C, Am A, et al. An experimental study on stability and thermal conductivity of water/CNTs nanofluids using different surfactants: A comparison study[J]. Journal of Molecular Liquids, 304, 2020, 111025.

[8] Zareei M, Yoozbashizadeh H, Hosseini H. Investigating the effects of pH, surfactant and ionicstrength on the stability of alumina/water nanofluids using DLVO theory[J]. Journal of Thermal Analysis and Calorimetry, 2018, 135(2): 1185-1196.

[9] Esfe M H, Amiri M K, Bahiraei M. Optimizing thermophysical properties of nanofluids using response surface methodology and particle swarm optimization in a non-dominated sorting genetic algorithm[J]. Journal of the Taiwan Institute of Chemical Engineers, 2019, 103: 7-19.

[10] Danish M, Yahya S M, Saha B B, et al. Modelling and optimization of thermophysical properties of aqueous titania nanofluid using response surface methodology[J]. Journal of Thermal Analysis & Calorimetry, 2020, 139(5): 3051-3063.

[11] Abdulrahman A. Modeling and optimization of dynamic viscosity of copper nanoparticles dispersed in gear oil using response surface methodology[J]. Materials Today: Proceedings, 2021, 42: 771-775.

[12] Esfe M H, Firouzi M, Rostamian H, et al. Prediction and optimization of thermophysical properties of stabilized Al2O3/antifreeze nanofluids using response surface methodology[J]. Journal of Molecular Liquids, 2018, 261: 14-20.

[13] 孙恩博，陈今茂，熊春华，等. 纳米流体稳定性及其导热性能研究[J]. 热能动力工程，2021，36(5)：61-65.

Sun Enbo, Chen Jinmao, Xiong Chunhua, et al. Research on the stability and thermal comductivity of nanofluids[J]. Journal of Engineering for Thermal Energy and Power, 2021, 36(5): 61-65. (in Chinese with English abstract)

[14] Zhang H, Yan S, Wang T, et al. Enhanced heat transfer of carbon nanotube nanofluid microchannels applied on cooling gallium arsenide cell[J]. Journal of Thermal Science, 2020, 29(6): 1475-1486.

[15] Mahbubul I M, Elcioglu E B, Amalina M A, et al. Stability, thermophysical properties and performance assessment of alumina⁃water nanofluid with emphasis on ultrasonication and storage period[J]. Powder Technology, 2019, 345: 668-675.

[16] Aziz S, Khalid S, Khalid H. Influence of surfactant and volume fraction on the dispersion stability of TiO2/deionized water based nanofluids for heat transfer applications[J]. Materials Research Express, 2019, 6(1): 015031.

[17] Han Xiaofei, Lu Liwei, Yan Suying, et al. Stability, thermal conductivity and photothermal conversion performance of water-based ZnO nanofluids[J]. 热科学学报：英文版, 2021, 30(5):1581-1595.

[18] 王功亮，姜洋，李伟振，等. 基于响应面法的玉米秸秆成型工艺优化[J]. 农业工程学报，2016，32(13)：223-227.

Wang Gongliang, Jiang Yang, Li Weizhen, et al. Process optimization of corn stover compression molding experiments based on response surface method[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2016, 32(13): 223-227. (in Chinese with English abstract)

[19] 张续成，亓伟，徐艳，等. 响应面法对磁性碳基固体酸催化剂的制备工艺优化研究[J]. 太阳能学报，2018，39(9)：7.

Zhang Xucheng, Qi Wei, Xu Yan, et al. Optimization of preparation process of magnetic carbon based solid acid catalyst by Response Surface Methodology[J]. Journal of Solar Energy, 2018, 39(9): 7. (in Chinese with English abstract)

[20] Esfe M H. Investigation the effects of different nanoparticles on density and specific heat： Prediction using MLP artificial neural network and response surface methodology[J]. Colloids and Surfaces A-physicochemical and Engineering Aspects, 2022, 645.

[21] Esfe M H, Alidoust S, Asdershiri E M, et al. Comparative rheological study on hybrid nanofluids with the same structure of MWCNT (50%)-ZnO(50%)/SAE XWX to select the best performance of nano-lubricants using response surface modeling[J]. Colloids and Surfaces A-physicochemical and Engineering Aspects, 2022, 641.

[22] 李学琴，时君友，亓伟，等. 响应面法优化生物质基固体酸催化剂的制备[J]. 太阳能学报，2015，36(5)：5.

Li Xueqin, Shi Qunyou, Qi Wei, et al. Optimization of preparation of biomass based solid acid catalyst by response surface methodology[J]. Journal of Solar Energy, 2015, 36(5): 5. (in Chinese with English abstract)

[23] 夏国栋，李奥，马丹丹. 铂锡合金纳米流体导热系数与稳定性影响因素分析[J]. 北京工业大学学报，2021，47(9)：8.

Xia Guodong, Li Ao, Ma Dandan. Analysis of factors influencing the thermal conductivity and stability of PtSn nanofluids[J]. Joural of Beijing University of Technology, 2021, 47(9): 8. (in Chinese with English abstract)

[24] 丁洁，王平，张本国，等. 混合基纳米流体在汽车散热器中的稳定性及传热特性[J]. 科学技术与工程，2019，19(1)：196-206.

Ding Jie, Wang Ping, Zhang Benguo, et al. Stability and heat transfer characteristics in automotive radiators of mixed⁃based Nan fluids[J]. Science Technology and Engineering, 2019, 19(1): 196-206. (in Chinese with English abstract)

[25] 沈向阳，李国铭，陈嘉澍，等. Al2O3/丙二醇纳米流体的稳定性研究及热物性测量[J]. 低温与超导，2021，49(9)：7.

Shen Xiangyang, Li Guoming, Chen Jiashu, et al. Study on stability of Al2O3/propylene glycol nanofluids and its thermo physical property measurement[J]. Cryogenics Refrigeration, 2021, 49(9): 7. (in Chinese with English abstract)

[26] 李兴，汪昭玮，孙一峰. 分散剂对纳米流体影响的研究进展[J]. 材料导报，2015，29(23)：6.

Li Xing, Wang Zhaowei, Sun Yifeng. Research of surfactant on the influence of nanofluid. Material Guide, 2015, 29(23): 6. (in Chinese with English abstract)

[27] Guo X, Zou X, Sun M. Optimization of extractionprocess by response surface methodology and preliminary characterization of polysaccharides from Phellinus igniarius[J]. Carbohydrate Polymers, 2010, 80: 344-349.

[28] 郑丹，王哲，陈占秀，等. 电加热结构内纳米流体稳定性实验研究[J]. 工程热物理学报，2021，42(4)：6.

Zheng Dan, Wang Zhe, Chen Zhanxiu, et al. Experimental study on the stability of nanofluid in an electric heating structure[J]. Journal of Engineering Thermophysics, 2021, 42(4): 6. (in Chinese with English abstract)

[29] 钟桂健，翟玉玲，包桂蓉，等. 纳米流体稳定性和粘度的影响因素分析[J]. 材料科学与工程学报，2020，38(1)：8.

Zhong Guijian, Zhai Yuling, Bao Guirong, et al. Analysis of factors influencing stability and viscosity of nanofluids[J]. Journal of Materials Science Engineering, 2020, 38(1): 8. (in Chinese with English abstract)

[30] Afzal A, Khan S A, Ahamed S C. Role of ultrasonication duration and surfactant on characteristics of ZnO and CuO nanofluids[J]. Materials Research Express, 2019, 6(11): 158.

[31] 王宏宇，王助良，杜敏，等. 纳米流体的制备及稳定性分析[J]. 河南科技大学学报：自然科学版，2016，37(1)：5.

Wang Hongyu, Wang Zhuliang, Du Min, et al. Preparati on and stability of nanofluids[J]. Journal of Henan Un iversity of Science and Technology Natural Science, 2016, 37(1): 5. (in Chinese with English abstract)

Stability of carbon tube nanofluid based on response surface methodology

Yan Suying1,2, Zhang Tiange1, Yuan Xue1, Gao Hong1, Zhang Huiying1, Zhao Xiaoyan1

(1.,,010051,; 2.,010051,)

Nanofluids can be a new type of heat transfer medium with the high thermal conductivity. Particularly, the traditional heat transfer media cannot fully meet the growing needs of thermal management in the electronic devices in various fields. Among them, the stability is a key factor for the wide application of nanofluids. In order to obtain more stable nanofluids, the dispersants were first screened, and then the stability of the Carbon NanoTube (CNT) nanofluids with the selected dispersant was optimized using the Response Surface Method (RSM). CNT nanofluids were prepared by the two-step method. The comprehensive evaluation index was used to compare the effects of four surfactants on the stability and thermal conductivity of CNT nanofluids. The stability evaluation included the particle size potential and absorbance method. Taking the particle size as the evaluation index, three influencing factors were selected as the mass fraction, ultrasonic oscillation time, and placement of CNT nanofluids. A three-factor and three-level Box-Behnken test was designed to optimize the parameters. The RSM was used to investigate the effects of various factors on the interaction of nanofluid stability. The results show that compared with the rest dispersants, the CNTs nanofluids with the DB dispersant presented the smallest particle size, the highest absorbance, the highest thermal conductivity, and the best comprehensive performance, when the ratio of carbon tube to dispersant was 1:2. Therefore, the DB dispersant was selected to evaluate the performance of CNTs nanofluids. A RSM model was established to accurately describe the particle size of CNT nanofluids using the Box-Behnken experimental design. At the same time, the quadratic polynomial regression equation was obtained for the response value particle size () with the mass fraction of CNT nanofluids (1), ultrasonic oscillation time (2), and storage time (3). The quadratic polynomial mathematical model was established by the experimental data. There was the highly significant (<0.0001) with the correlation coefficient2=0.964 4, indicating an excellent fit between the predicted and the actual value. It infers that there was an excellent consistency between the model and the experimental data. An optimal combination of factors was achieved for the stability of CNT nanofluids using the quadratic regression model, where the mass fraction of CNT nanofluids was 0.27%, the ultrasonic time was 83.45 min, and the standing time was 8 h. In this case, the average particle size was 121.58 nm, and there was a 0.016% error with the predicted value of 120.60 nm. Therefore, this improved model was feasible to optimize the stability of CNT nanofluids. The actual value was close to the predicted value. There was a certain reference value for a theoretical and experimental basis, in order to improve the stability of nanofluids. Response surface test also revealed that the ultrasonic oscillation time and placement time can be selected to improve the stability in the subsequent preparation of nanofluids.

nanofluid; stability; thermal conductivity; dispersant; response surface methodology

10.11975/j.issn.1002-6819.2022.21.030

TK

A

1002-6819(2022)-21-0261-07

闫素英，张田歌，袁雪，等. 基于响应面法的碳管纳米流体稳定性[J]. 农业工程学报，2022，38(21)：261-267.doi：10.11975/j.issn.1002-6819.2022.21.030 http://www.tcsae.org

Yan Suying, Zhang Tiange, Yuan Xue, et al. Stability of carbon tube nanofluid based on response surface methodology[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2022, 38(21): 261-267. (in Chinese with English abstract) doi：10.11975/j.issn.1002-6819.2022.21.030 http://www.tcsae.org

2022-07-28

2022-09-03

国家自然科学基金资助项目（No.51766012）；内蒙古科技重大专项（No.2020ZD0017)

闫素英，博士，教授，研究方向为新能源中的工程热物理方面。Email：yansy@imut.edu.cn