A two-dimensional air streamer discharge model based on the improved Helmholtz equation at low temperature and sub-atmospheric pressure

Zhihang ZHAO (赵志航),Xinlao WEI (魏新劳),Shuang SONG (宋爽),Lin CUI (崔林) and Longfei ZHANG (张龙飞)

1 Key Laboratory of Engineering Dielectrics and Application of Ministry of Education,Harbin University of Science and Technology,Harbin 150080,People’s Republic of China

2 No.703 Research Institute of CSIC (China Shipbuilding Industry Corporation),Harbin 150078,People’s Republic of China

3 Yunnan Electric Test&Research Institute Group Co.,Ltd.,Kunming 650217,People’s Republic of China

Abstract

Keywords: efficient boundary condition,discharge current,propagation velocity,threshold value,low temperature and sub-atmospheric pressure

1.Introduction

Aircraft,airships,launch vehicles and other aircraft flying at high altitude will accumulate a large number of static charges due to a variety of electrostatic starting mechanisms.When the flying height is increasing and the air temperature and pressure are decreasing,electrostatic discharge can easily occur between the parts [1].The reliable performance and normal operation of electric power system components and sub-assemblies under operating conditions are essential to the operation and survival of aerospace equipment.It is difficult to carry out the test on the test platform at high altitude,with high costs,long cycles and limited testing time,and little data is obtained from the test,which is not convenient for systematic analysis.In [2] and [3],the discharge environment at high altitude was simulated,and the partial discharge and corona discharge were studied.Some other research on partial discharge involves hybrid electric propulsion(HEP)systems,which are applied to hybrid electric aircraft (HEA) in conceptual designs [4].

With the further development of China’s aviation industry,the requirements for aircraft structure have also been improved.Under the premise of satisfying the safety operation,the optimization of the air gap structure has become an urgent problem to be solved in aviation and high voltage engineering,and the breakdown characteristics of air are one of the key factors that affect the structure of the air gap.With the increase in altitude,air pressure and temperature decrease in proportion.Studies on air breakdown characteristics under such specific conditions are rare at home and abroad.Therefore,the study of air breakdown characteristics at low temperature and sub-atmospheric pressure has a certain significance in the promotion of the development of high voltage technology in the aviation field.

As a precursor of streamers,the electron avalanche has been studied by scholars as early as the beginning of the 20th century.Townsend proposed that the Townsend theory[5,6]laid the theoretical foundations of gas discharge.With the development of the study of gas discharge,it is difficult to explain some phenomena only using the Townsend theory(Rogowski scholars in 1928 are the earliest record of paper[7]),such as the fact that development speed is faster than impact ionization discharge,the discharge channel is not uniform but is a bright thin channel with branches,the breakdown voltage measured in the air at atmospheric pressure is independent of the cathode material,and so on.In the 1930s,Raether [8,9],and Loeb and Meek [10,11],respectively,put forward the streamer theory to supplement the deficiency of the Townsend theory.They have established simple models to predict the breakdown field of the gas discharge,but there is a certain gap between the results and the experimental measurements,and there was no further analysis of the basic streamer characteristics,such as streamer space charge and streamer development speed.Since then,scholars at home and abroad have carried out a lot of research on the theory of streamers,and it is of great significance to study the gas breakdown characteristics by studying the streamer theory.

Experiments are the foundation of theory and the most important criterion for testing theory.At present,the experimental research on the streamer theory includes the measurement of macroscopic parameters such as the corona starting voltage and discharge current,while the direct observation research on microscopic parameters,such as electron temperature and electron density,in the streamer is also improved compared with the previous research.Although streamer discharge has high resolution in time and space,Ivanov et al [12] still used a specially developed experimental setup to study the streak of the glow accompanying pre-breakdown and breakdown processes in the gap.Yatom et al [13] used different types of electrical,optical,x-ray,spectroscopy and laser diagnostics,and space-resolved and time-resolved plasma parameters were determined.Briels et al [14] found that there was a strong asymmetry in the propagation and development of positive and negative streamers at low voltage.With the improvement of computer speed and the development of numerical calculation methods,numerical simulation is still one of the effective means to study the characteristics of streamer propagation.At present,most of the streamer discharge processes are solved by a fluid model.The fluid model is formed by coupling the continuity equation of the particle with the Poisson equation for the potential,and photoionization is added to the continuity equation for electrons and positive ions as the source term.

In the early streamer simulation,photoionization was generally ignored or replaced by spatially uniform background ionization.Scholars were not satisfied with such equivalent substitution.To get closer to the reality,it has become common to use the integral model based on the absorption function of Zheleznyak et al [15] to solve the photoionization.Although the accuracy of the integral model is high,it requires a lot of time to solve the photoionization in each time step.To shorten the calculation time,Kulikovsky[16] proposed an approximation method,which greatly improved the efficiency of the calculation,but ignored the details of the discharge characteristics.Bourdon et al [17]converted the integral model into a set of Helmholtz differential equations,which not only greatly improved the calculation efficiency,but also retained the accuracy of the original integral model.

This paper adopts the mathematical equivalent method of three-group SP3proposed by Bourdon et al [17]: the sixexponential fit for the Helmholtz model,and we propose a more effective boundary condition to make it more accurate.The effectiveness of improved boundary conditions is verified by comparing the photoionization generated by Gaussian distribution ionizing radiation.Finally,the double-headed streamer propagation process at low temperature and subatmospheric pressure is simulated numerically using the fluid equation.Under the premise that the applied voltage exceeds the threshold value of the voltage [18],the discharge process is divided into two stages and the parameters such as streamer propagation velocity,discharge current,electron density,electric field and net charge are discussed.The transport parameters involved in the equation all refer to those shown in [19].

2.Simulation model

2.1.Fluid model for transient streamer propagation

An air streamer is a transient ionization wave,which travels very fast.At atmospheric pressure,the propagation velocity of the streamer is very fast,about 1% of the speed of light.The streamer also has a filamentary structure.These characteristics make it difficult to obtain microcosmic parameters of the streamer through only experimental research.Therefore,numerical simulation has gradually become one of the main tools for understanding the characteristics of the streamer.The vast majority of numerical studies follow the work of Davies et al [20],which uses a continuity equation to model the streamer process,called the hydrodynamic or fluid model.

The streamer discharge process has strong nonlinearity both in space and in time.In a very short propagation time,the convection and diffusion motions of ions are negligible.The simplest fluid model in this paper,including electron convection and the diffusion reaction equation,and the positive ion reaction equation coupled with the Poisson equation,is given by:

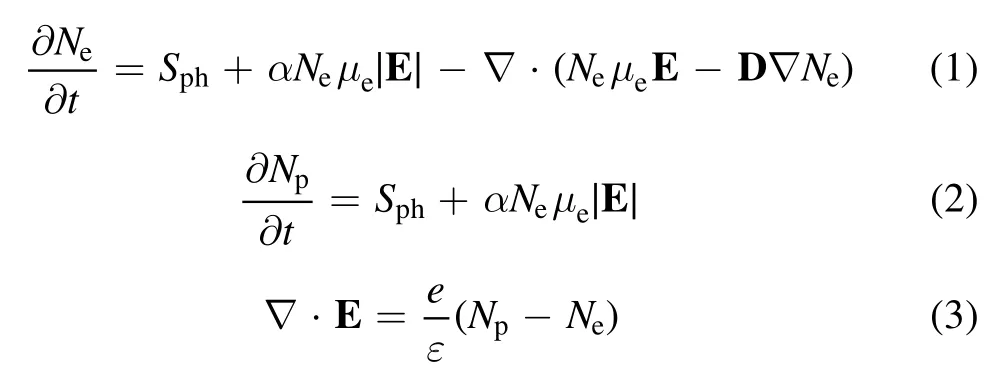
where Neand Npare,respectively,the electron density and positive ion density (cm-3),t is time (ns),μeis the electron mobility (cm2V-1s-1),D is the electron diffusion coefficient(cm2s-1),α is the collision ionization coefficient (cm-1),Sphis the photoionization rate (cm-3s-1),E is the electric field intensity (V/cm),ε is the dielectric constant of the gas and e is the elementary charge.

2.2.Photoionization model

2.2.1.Classical integral model.The classical integral model has been widely used by scholars such as Pasko et al[21]and Naidis [22].Considering two volumetric elements dV1and dV2separated by,the photoionization of UV photons emitted from dV1by oxygen molecules is [17].

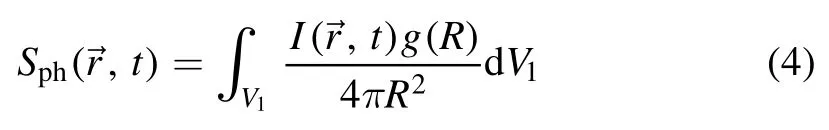
where ξ is the average photoionization efficiency in the interval 980–1025 Å,and pq/(p + pq)is a quenching multiplier(p is the gas pressure and pqis the quenching pressure of the singlet states of N2).The quenching pressure is assumed to be pq= 30 Torr[23],νuis the electron impact excitation frequency for level u and viis the ionization frequency.It can be found that νuand viare functions of a reduced electric field,andis the weak function of the reduced electric field E/N.In [16] the photoelectron production rate is expressed in terms of the ionization rate assumingwhich is a reasonable approximation.Here,g(R) in equation (4) is defined as

where χmin= 0.035 Torr-1cm-1,χmax= 2 Torr-1cm-1andpO2is the partial pressure of molecular oxygen (=150 Torr at atmospheric pressure).

The photoionization calculation needs to be carried out at each time step,and the traditional integral model is calculated in the three-dimensional space.Although the accuracy is very high,the calculation needs a lot of time.Kulikovsky [16]approximated that photoionization is uniform on the radial disc or cylindrical ring,and converts the complex triple integral into a simple one or two integrals.Although it saves calculation time,it ignores the details of the streamer current process,which leads to a decrease in the accuracy.When the streamer bifurcates,the hypothesis cannot be realized.

2.2.2.Helmholtz model.Luque et al [23] and Bourdon et al[17]expressed the absorption function as the form of a series sum,thus converting the integral model of photoionization into a set of Helmholtz equations.The photoionization expression given by [17] is

where

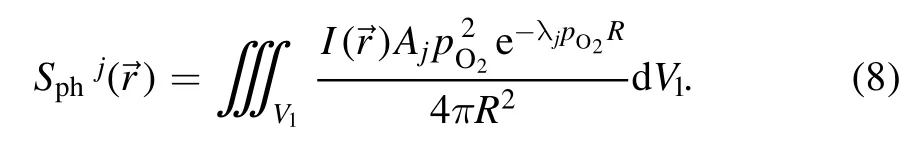
Then,it satisfies the Helmholtz equation.

Compared with the classical integral model,the Helmholtz equation can be divided into two forms:two-exponential fit and three-exponential fit.Bourdon et al [17] pointed out that the two-exponential fit was only applicable to the range of 1 ＜pO2R ＜ 60 Torr cm,while the three-exponential fit extended the applicable range to 1 ＜pO2R ＜ 150 Torr cm,which maintained good consistency with the classical integral model.

2.2.3.Three-group Eddington and SP3approximations.The photoionization source termin the work of Ségur et al[24]is calculated using direct numerical solutions of the first-(we refer to it as Eddington approximation in this paper) and the third-order (we also refer to it as SP3in this paper)Eddington approximations of the radiative transfer equation.Ségur et al [24] introduced a simple monochromatic approach.It is only limited to a high precision when the light absorption efficiency coefficient is large.To maintain a better consistency between the Eddington approximation and SP3and the classical integral model,Bourdon et al [17]adopted a method similar to a Gaussian type orthogonal [25]to improve the original model on the premise that only the isotropic part of the photoelectric dispersion term distribution function was considered.For the equation

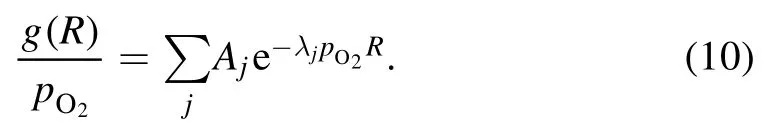
Bourdon et al[17]used the idea of the three-exponential fit to obtain the values of the parameters Ajand λjin equation(10),and then obtained a new approximation model;we also refer to it as the three-group method.By comparison,it is found that the application of the three-group method is further extended to 0.1 ＜pO2R ＜ 150 Torr cm,and accurate results can be obtained,even when the light absorption coefficient is relatively small.It is worth noting that the fitting results obtained using the three-group method are usually more accurate than the three-exponential fit for the Helmholtz model.

Table 1.Parameters of the six-exponential fit for the Helmholtz model.

In terms of structure,the Helmholtz equation is involved in both the three-group Eddington approximation and threegroup SP3equation.Bourdon et al [17] established the mathematical relationship of the three methods based on the structural commonality.The parameters in the three-exponential fit for the Helmholtz model were applied to the threegroup Eddington approximation and three-group SP3,and to obtain their equivalent form,its accuracy compared with the original algorithm is further improved.This paper uses the six-exponential fit for the Helmholtz model equivalent to the three-group SP3,where the parametersAj′ andλ′jare calculated based on Ajand λjin the three-exponential fit for the Helmholtz model.

2.3.Improved boundary conditions

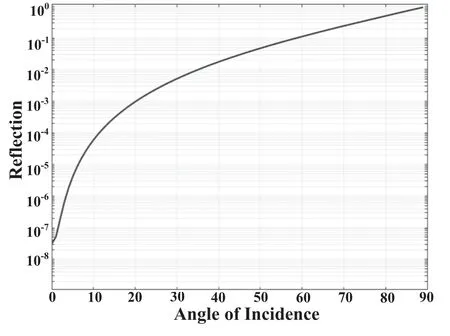
Figure 1.Reflection of a plane wave at the first-order SBC with respect to the angle of incidence.

In addition to using reasonable and efficient algorithms,effective boundary conditions are also one of the necessary conditions to ensure accurate results.Luque et al[23]adopted the zero boundary condition that is only effective when the boundary is far from the source,while Bourdon et al [17]considered the differential equation with the smallest absorption coefficient in the integral boundary,and the other differential equations are the zero boundary.This paper argues that the above two boundary conditions are not very accurate and lack a certain theoretical basis.According to the physical characteristics of the radiation,Cai et al [26]approximated that the boundary of the computational domain(except for the axis of symmetry) satisfies the far-field radiation boundary,that is,the Sommerfeld radiation boundary.

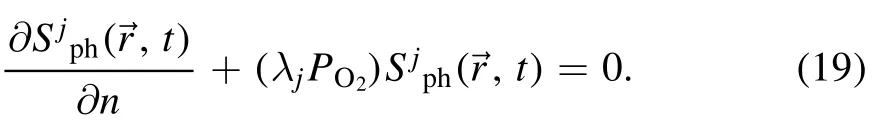
It is proved that the boundary condition is more effective and consistent with the results of the Zheleznyak et al [15]model.In this paper,the approximation of equation (19) is written as follows:

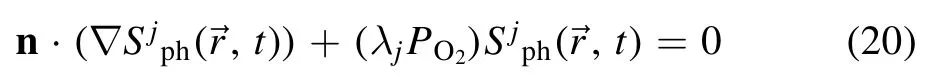
where n is the unit normal vector.This paper renames equation (20) as a first-order scattering boundary condition(SBC).This boundary condition is often used to solve electromagnetic wave problems.When electron density varies with time and space,the streamer can also be regarded as a transient ionization wave; therefore,this paper applies this boundary condition to the solution of the photoionization rate.Although the first-order SBC has been greatly improved in accuracy relative to the boundary conditions of Luque et al[23]and Bourdon et al[17],this boundary condition still has a very large limitation: there is no reflection only when the radiation is accurately incident on the boundary along the normal direction.All illegally reflected waves incident on the first-order SBC will be partially reflected.Figure 1 shows the reflection coefficients of plane waves at different incident angles on the first-order SBC.

From figure 1,we observe that as the incident wave approaches tangential incidence,the degree of reflection of the wave gradually increases until it is completely reflected.The angle of incidence is 60° and the degree of reflection is about 10%.Obviously,the first-order SBC is not the most effective boundary condition.To obtain more accurate boundary conditions,the second-order term (the second tangential derivative ofalong the boundary) is added into equation (20) in this paper.

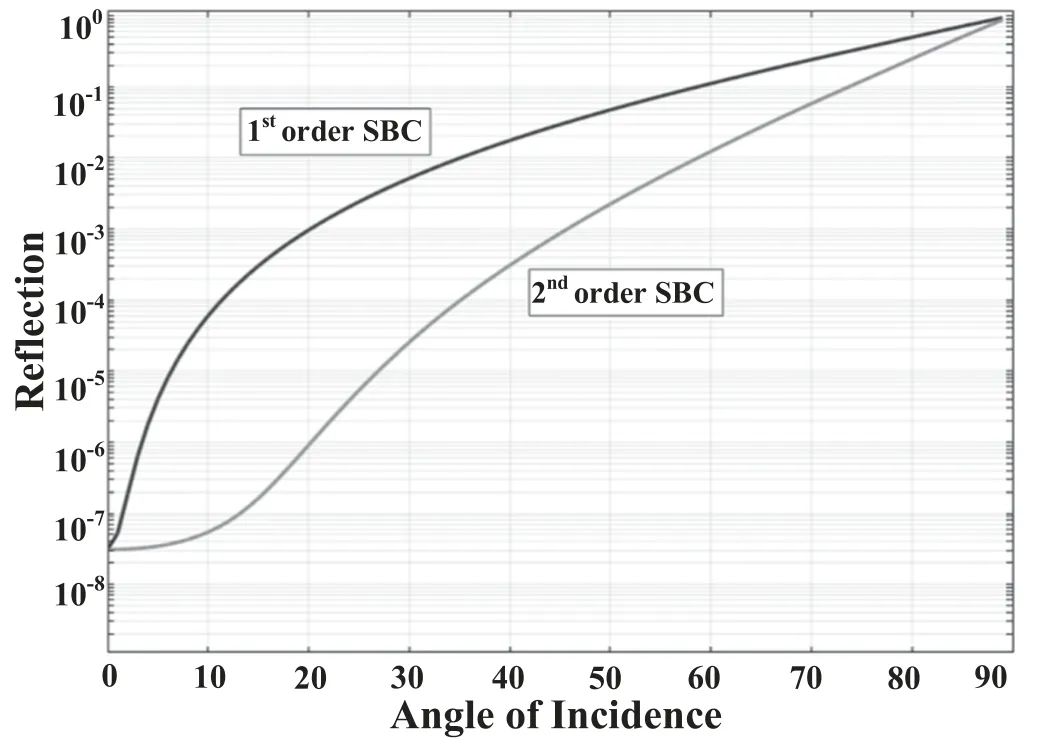
Figure 2.Reflection of a plane wave at the first-and second-order SBC with respect to the angle of incidence.

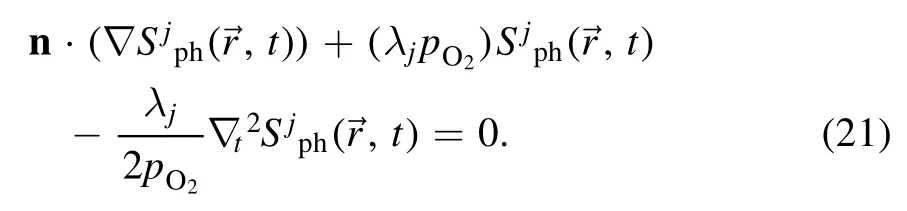
In figure 2,we compared the reflection coefficients of the first-order SBC and second-order SBC.

After comparison,it was found that the second-order SBC could only make the reflection degree reach 10% when the incident angle was about 75°,and the situation was obviously improved.Compared with the first-order SBC,the second-order SBC showed better consistency.In the following sections,the second-order SBC is combined with the sixexponential fit for the Helmholtz model to perform a detailed analysis of the double-headed streamer propagation process at low temperature and sub-atmospheric pressure.

3.Results and discussion

The model in this paper is a two-dimensional axisymmetric configuration,which is implemented using the COMSOL Multiphysics 5.4®package based on the finite element method (FEM).All numerical experiments are conducted using a workstation computer with two 2.9 GHz Intel(R)Xeon processors in 64-bit mode running in 256 GB randomaccess memory (RAM),all running on Windows 7.

3.1.Gaussian photoionization sources

In this section,the photoionization of the Gauss radiation source is calculated using different photoionization models.Assume that the length and radius of the two-dimensional axisymmetric region are Ldand Rd.The intensity of the radiation source is

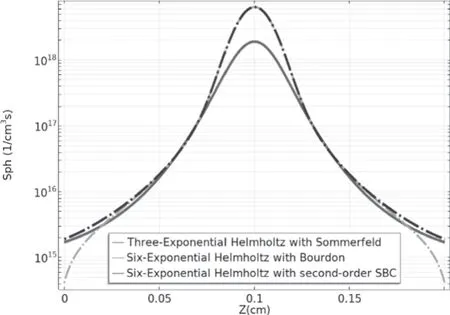
Figure 3.Axial calculation comparisons of different models.Solid line: the three-exponential Helmholtz with Sommerfeld model.Dotted line: the six-exponential Helmholtz with Bourdon model.Point dashed line: the six-exponential Helmholtz with the secondorder SBC.

Figure 4.Radial calculation comparisons of different models.

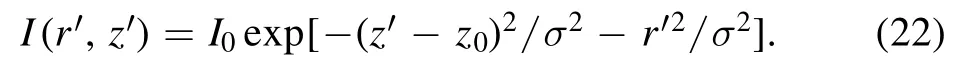
According to[26],it is known that I0= 2.3 ×1019cm-3s-1,where z0is the axial position of the radiation source,and σ is the parameter of the spatial width of the radiation source.

Figures 3 and 4 are the results calculated at σ = 0.01 cm,z0= 0.1 cm and Ld= Rd= 0.2 cm.This paper only compares the size of the streamer head in air with low temperature and sub-atmospheric pressure at σ = 0.01 cm.

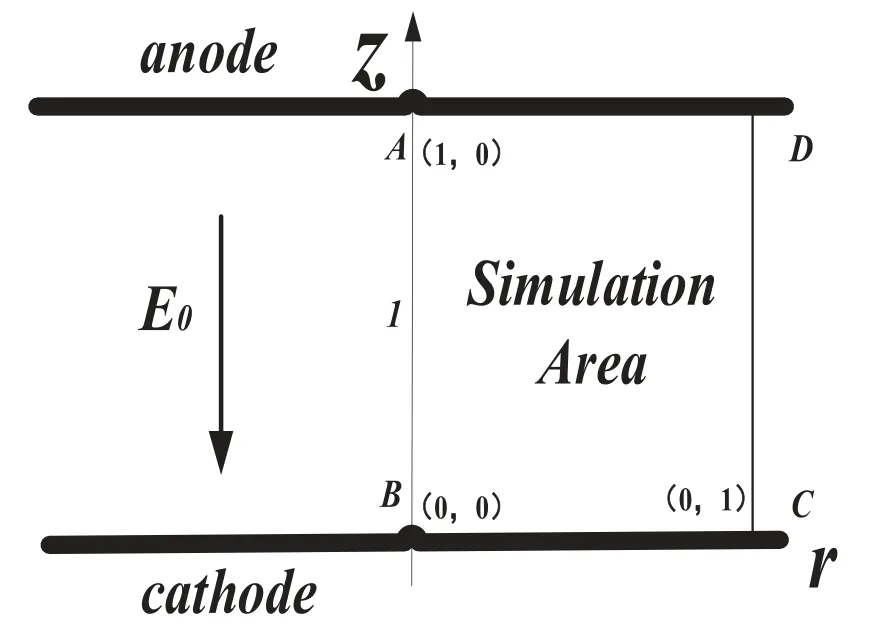
Figure 5.Configuration of the simulation domain.

Figure 3 is a comparison of the axial calculations of three different models.It has been pointed out in Bourdon et al[17]that the accuracy of the three-group SP3model is better than that of the three-exponential fit for the Helmholtz,and the sixexponential fit for the Helmholtz model has been further optimized on the basis of the three-group SP3model.Therefore,this paper uses the six-exponential Helmholtz with Bourdon model as the benchmark.Through comparison,the six-exponential Helmholtz with Bourdon model and six-exponential Helmholtz with the second-order SBC maintain good consistency in the simulation area,except for the boundary,and are superior to the three-exponential Helmholtz with Sommerfeld model.It proves again that the accuracy of the three-group SP3model is better than that of the three-exponential fit for the Helmholtz.At the boundary,the consistency of the six-exponential Helmholtz with the second-order SBC and three-exponential Helmholtz with Sommerfeld model is better than that of the six-exponential Helmholtz with Bourdon model,which also proves the irrationality of the boundary conditions in Bourdon et al [17].According to the above conclusions,the accuracy of the sixexponential Helmholtz with the second-order SBC is the best in the whole simulation area.

Figure 4 is the comparison of the radial calculation of the three different models,which is almost consistent with the conclusion obtained in figure 3.This paper will not be repeated here.It is worth noting that although the photoionization calculated by the three models is different,it has little influence on the simulation results.The main reason is that the number of electrons produced by electron collision ionization in the area near the radiation source is much higher than that produced by photoionization.

3.2.Double-headed streamers

This section only takes the low temperature and sub-atmospheric pressure environment of the aircraft in the 11 km troposphere as an example to simulate the propagation process of double-headed streamers under the DC voltage and a uniform electric field.The simulation area is shown in figure 5.

In figure 5,the electric field E0supplied by the plate to the calculated area is 52 kV cm-1(the voltage can be seen as constant in 3.5 ns),and in the calculation area,Ld=Rd= 1 cm.The threshold value of the electric field has important theoretical and practical significance for understanding the process of the gas discharge.The calculation formula of the electric field threshold is provided in[18],and the error is 3%.

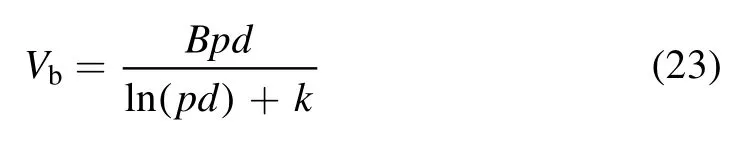
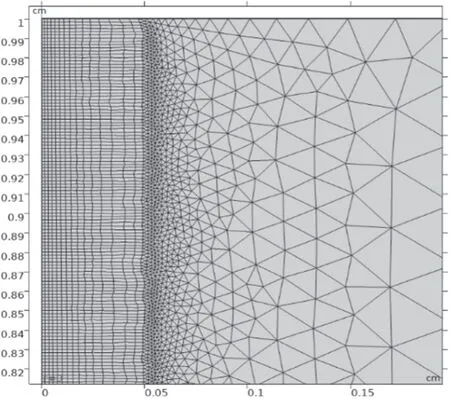
Figure 6.Mesh generation.

where

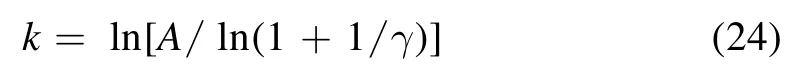
where p is the pressure,d is the gap spacing and γ is the secondary ionization coefficient,A is the saturation ionization in the gas at a particular E/p and B is related to the excitation and ionization energies.According to the parameter calculation in[18],the threshold value of the electric field under the simulation condition in this paper is 8.4 kV cm-1.Although the influence of temperature is not considered in the formula in [18],the actual electric field threshold is similar to the calculated result.In this paper,52 kV cm-1is selected as the electric field intensity,which is consistent with the value in[26]and provides the condition that the electric field exceeds the threshold value for the streamer discharge.

It can be seen from the calculation that the parameters in the simulation area are p = 170 Torr,T = 227 K,where T is the temperature and is substituted into the equation.

where kBis the Boltzmann constant,kB= 1.380 649 ×10-23J K-1,and calculation shows the neutral density N =7.23 × 1018cm-3.Therefore,the threshold of the reduced electric field E/N under the simulation condition is obtained as 116 Td (1 Td = 10-17Vcm2).All transport parameters(such as the electron collision ionization coefficient α and diffusion coefficient D) were referred to in the formula in[19].Most of the coefficients in the model are assumed to be functions of the local reduced electric field E/N.At the initial time(t = 0),the density distribution of electrons and positive ions is

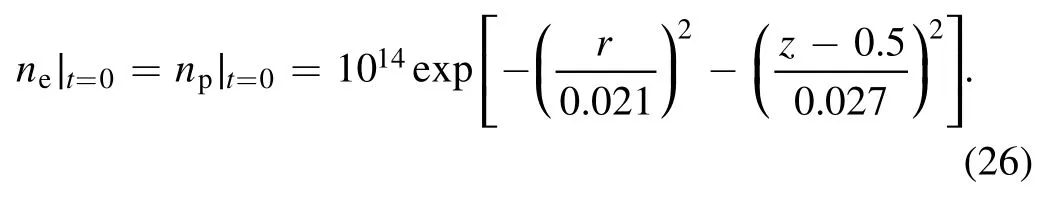
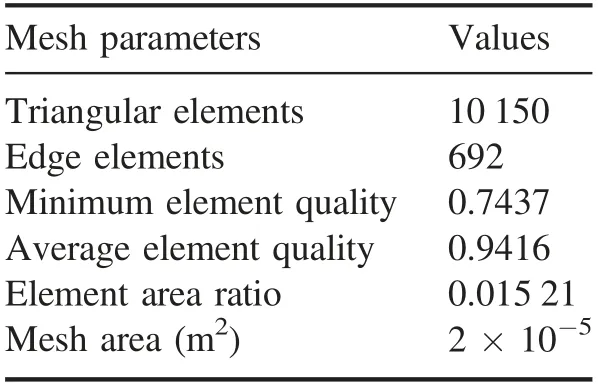
Table 2.Mesh statistics.

Streamer discharge occurs in the axial direction within the streamer radius.Therefore,a fine grid is shown in figure 6.

The grid parameters are shown in table 2.The quality of the mesh elements is an important consideration.According to table 2,the minimum unit mass is 0.7437 and the average unit mass is 0.9416.

The boundary conditions involved in this paper are shown in table 3.

To better study the characteristics of streamer generation and propagation,we divide the streamer discharge process into two stages:the initial stage of streamer development,and the accelerated stage of streamer development.

Firstly,based on the electron density distribution calculated by three different models,five different moments of t = 0 ns,t = 0.1 ns,t = 0.3 ns,t = 0.5 ns and t = 0.7 ns were selected for comparison.According to figure 7,whether in the initial stage or the accelerated stage,there is little difference in the electron density between the head and the internal electron density calculated based on different models.Such differences have little effect on the streamer propagation process.

Figure 8 shows the electric field distribution calculated by three different models.The same condition is selected at five different times: t = 0 ns,t = 0.1 ns,t = 0.3 ns,t = 0.5 ns and t = 0.7 ns.It can be seen that the electric field of the negative streamer is smaller than that of the positive streamer.This is because the polarity effect of the streamer dilutes the electron density of the negative streamer head,thus weakening the electric field of the streamer head.The difference in the electric field intensity between the doubleheaded streamers calculated based on different models is still very small and has no influence on the streamer propagation process.

In addition to the electric field intensity,the discharge current in the streamer process is also one of the important parameters reflecting the macroscopic performance.The discharge current is determined by the movement of charged particles between electrodes,mainly including the migration movement of electrons,positive ions and the diffusion movement of electrons.Because the diffusion velocity of ions is much lower than that of electrons,it can be neglected in the discharge process.The total current of the streamer discharge is composed of the conduction current and displacement current.In the initial stage of streamer discharge,the displacement current caused by the change in the electric field is dominant.When entering the stage of streamer acceleration,the proportion of the displacement current decreases,and the conduction current is dominant.Therefore,the discharge current in the process of streamer development can be expressed as:

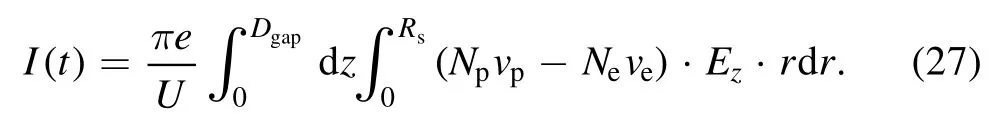
Equation (27) is basically consistent with the expression of the discharge current in [27],where Dgapis the gap distance(mm)and Rsis the radius of the streamer channel(mm).The definition method can refer to [28]: take the edge where the electron concentration is 10% of the central peak concentration as the streamer boundary,and the corresponding radius is the streamer radius.The size is about 0.1–0.5 mm.From the positive and negative streamer discharge currents given in figure 9,it can be seen that in the initial stage of streamer development,the streamer development is mainly maintained by the background electric field.Because the particle migration velocity is related to the electric field,the particle migration velocity is also constant under the constant background electric field; therefore,the streamer discharge current is constant and is maintained at about 10 mA.In the accelerated stage of streamer development,the electric field of the streamer head has been greatly enhanced and the impact ionization has an absolute advantage.The particle migration becomes very intense at high field strength,and the streamer discharge current shows an exponential growth law.The growth rate of the negative streamer discharge current is less than that of the positive streamer discharge current,which is consistent with the trend in[27].For the positive and negative streamer simulation in this paper,the discharge current is as follows:

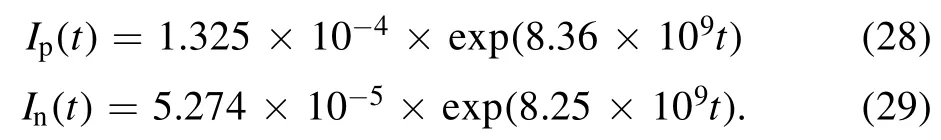
The photoionization rate in the streamer head region is closely related to the collision ionization rate,and their distribution in the development direction is approximately the same.Figure 10 shows the photoionization rate Sph(solid line) and collision ionization rate Si(dotted line) at the times of 0.1 ns and 0.2 ns.It is easy to see that in the initial stage of streamer development,the overall photoionization rate and collision ionization rate are not very different in the direction of streamer development.However,as the streamer continues to develop,the photoionization rate is reversed by the collision ionization rate of electrons.The maximum value of Siis about 1024cm-3s-1,which is two orders of magnitude larger than the maximum value of Sph(1022cm-3s-1).On the whole,Sphis negligible compared with Si,which is consistent with the conclusion mentioned in section 3.1 that the number of electrons produced by collision ionization is much higher than that produced by photoionization.

Figure 7 shows that the electron density of the streamer head remains almost unchanged,about 1014cm-3,which is consistent with the estimated 1014–1016cm-3in [28].In figures 11 and 12,t = 0 ns,t = 0.1 ns,t = 0.2 ns,t = 0.3 ns,t = 0.4 ns,t = 0.5 ns,t = 0.6 ns and t = 0.7 ns were selected to compare electron density and positive ion density at eight different moments.

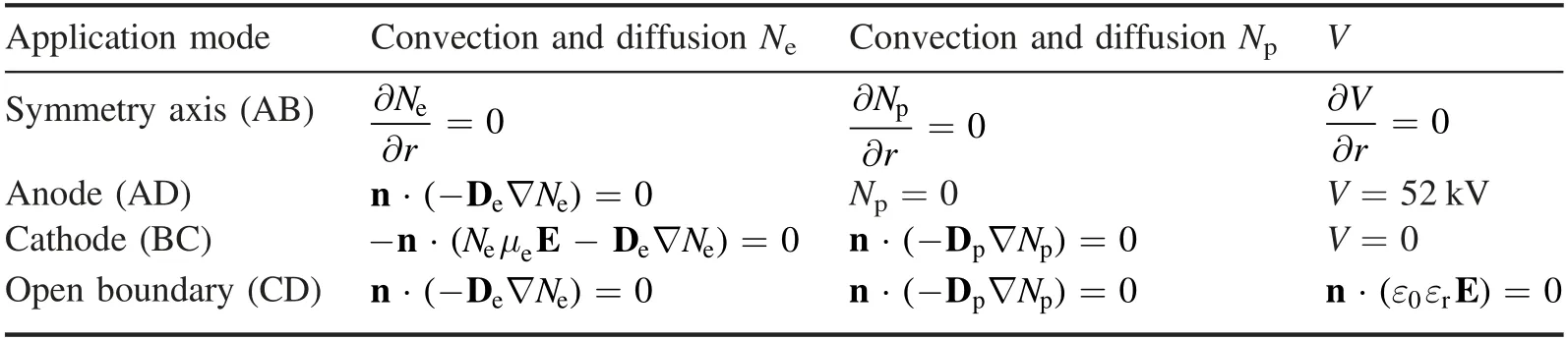
Table 3.Boundary conditions.

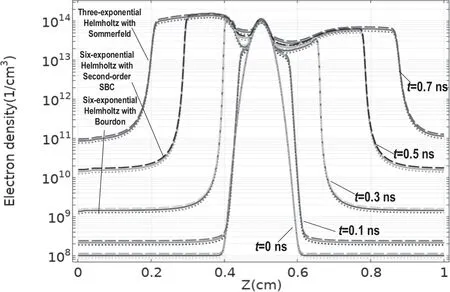
Figure 7.The calculated electron density based on different photoionization models.

Figure 8.The calculated electric field based on different photoionization models.

The above figures show that the spatial density distribution characteristics of electrons and positive ions are basically the same.This is because,in the space synthesis field,the electron collision ionization plays a major role,while the electron diffusion,attachment and composite effects are negligible compared with the collision ionization,and the electrons and positive ions appear in pairs in the collision ionization,thus the spatial density distribution characteristics of electrons and positive ions are basically the same.

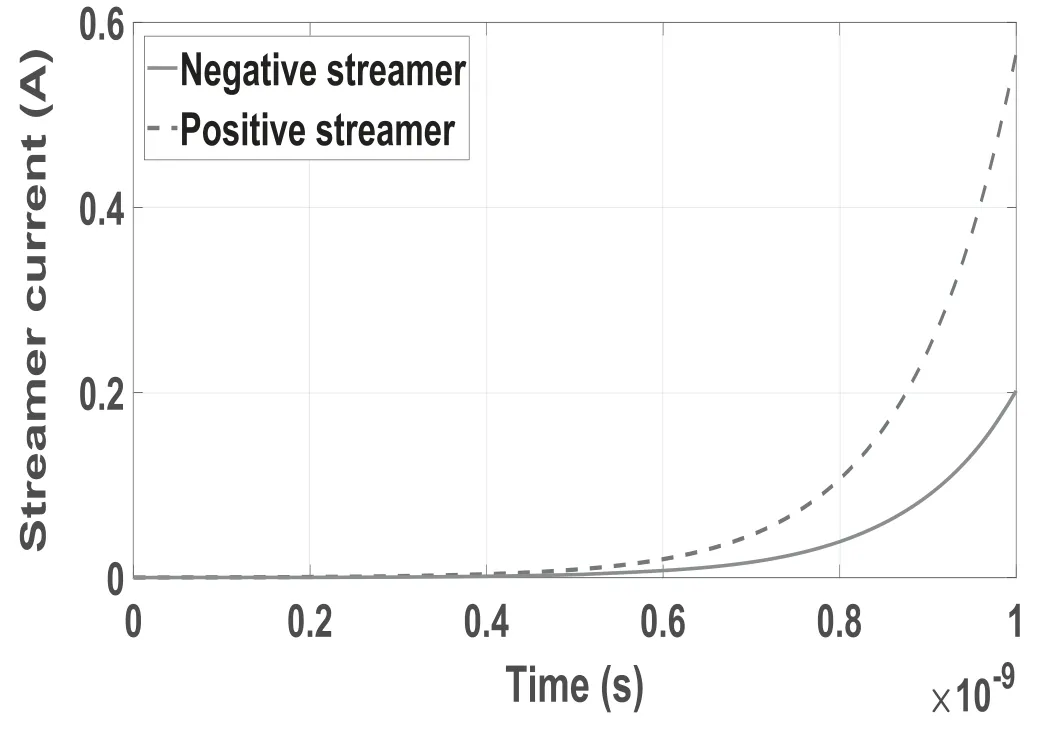
Figure 9.The streamer discharge current.

According to the distribution characteristics of electron density,it can be seen that the electron density of the positive streamer head is slightly higher than that of the negative streamer head.The reason is that the development direction of the positive streamer head is opposite to that of the electron,while the development direction of the negative streamer head is the same as that of the electron.During streamer propagation,the effect of space charge on the electric field distortion of the positive streamer head is greater,resulting in the head impact ionization and photoionization reaction of the positive streamer head being higher than that of the negative streamer head.The simulation results are consistent with those in [26].

The electric field is the main driving force of streamer development; therefore,the dynamic characteristics of streamer development are directly determined by the changing characteristics of the space electric field.In figures 13 and 14,t = 0 ns,t = 0.1 ns,t = 0.2 ns,t = 0.3 ns,t = 0.4 ns,t = 0.5 ns,t = 0.6 ns and t = 0.7 ns were selected to compare the electric field intensity and space charge density at eight different moments.

The internal electric field of the streamer channel is a self-consistent field.The electric field amplitude in the streamer channel mainly depends on the conductivity of the streamer and the current flowing through it.The streamer channel is a plasma channel with high concentration and high conductivity,thus the electric field in the streamer channel is relatively small.When the streamer develops to half of the gap,the field intensity in the streamer channel increases gradually.The main reason is that with the increase in the electric field in the streamer head,the high-speed electrons generated by the electron avalanche enter the streamer channel,resulting in ionization in the streamer channel,thus increasing the free charge density in the streamer channel.With the movement of electrons,the separation of electrons and positive ions in the center leads to the increase in the space net charge,thereby enhancing the electric field.The distribution characteristics of the net space charge in the streamer head also directly determine the enhancement of the field intensity in the streamer head.To better describe the propagation velocity of positive and negative streamers more conveniently,the definition of the head position of the streamer is given here.There are two methods to define the position of the streamer head: the space field strength maximum method,and the space net charge maximum method.They respectively define that the point with the largest space field strength and the point with the largest space net charge on the z-axis are the position of the streamer head.From the figure,we can see that the position of the streamer head determined by the two methods is basically consistent.However,due to the thickness of the space net charge layer,the position determined by the maximum space field strength method is slightly larger than that determined by the maximum space network charge method.Because the difference between them is not big,this paper chooses the method of the maximum space field strength to determine the position of the streamer head.

Figure 10.The photoionization rate and ionization rate at 0.1 ns(top)and 0.2 ns (bottom).

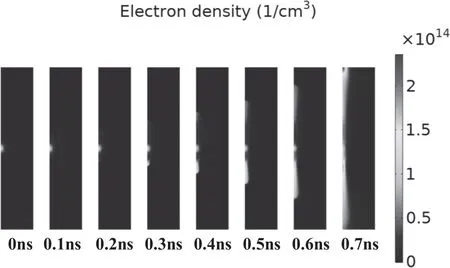
Figure 11.Electron density distribution.

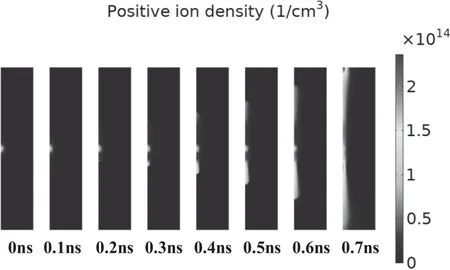
Figure 12.Positive ion density distribution.

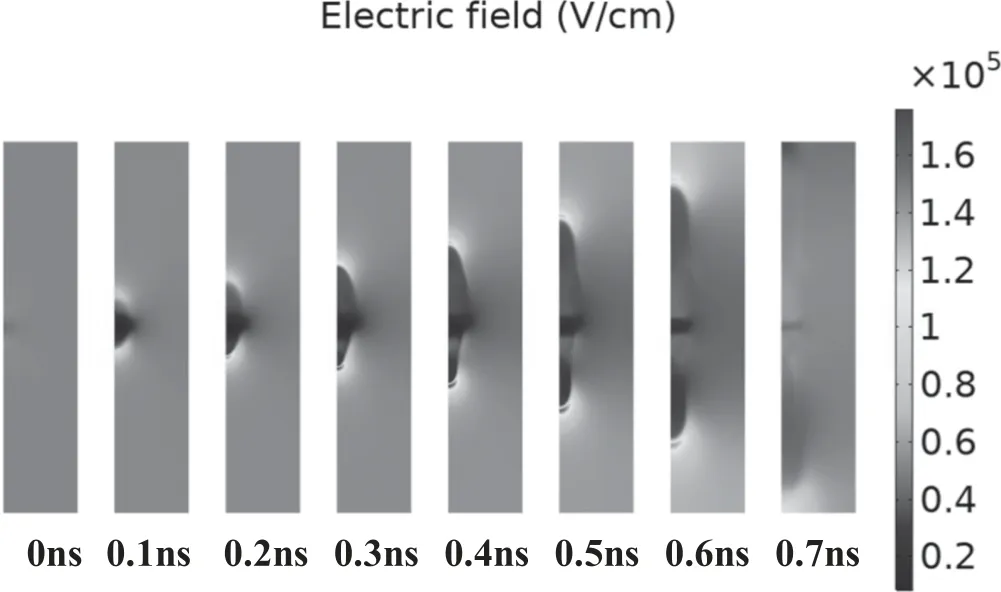
Figure 13.Electric field distribution.

Figure 14.Space charge density distribution.

Figure 15.The photoionization rate distribution.

Figure 16.Positive and negative streamer propagation velocity distribution.

In figure 15,eight different moments mentioned above are also selected to show the change process of the photoionization rate.It can be seen that the photoionization reaction is mainly concentrated in the head region of the streamer,and the photoionization rate in other regions is small.

The velocity of the streamer propagation is also an important parameter in the process of streamer development.In figure 16,t = 0.1 ns,t = 0.2 ns,t = 0.3 ns,t = 0.4 ns,t = 0.5 ns,t =0.6 ns and t = 0.7 ns were selected to show the differences in the propagation velocities of positive and negative streamers.

It can be seen that in the initial stage of streamer development,the propagation velocity of the negative streamer is faster than that of the positive streamer,mainly because the development direction of the negative streamer is consistent with that of electron migration.However,as the streamer approached the plate electrode gradually,when it reached about 0.5 ns,the velocity of the positive streamer caught up with that of the negative streamer and finally surpassed it.This is due to the higher electron density in the head of the positive streamer and the higher electric field generated by the space charge.It can be seen that the difference in electron density at the head of the streamer and the influence of the space charge electric field on the ionization rate are indeed the reasons for the different propagation velocities of the positive and negative streamers.

Although both temperature and pressure have effects on streamer discharge and propagation,the effect of temperature change on the streamer discharge process is less than that of the pressure.Therefore,this paper only discusses the characteristics of streamer discharge under different pressures.The influence of temperature on the parameters in the streamer discharge channel will be analyzed in detail in the following paper.

To facilitate comparison,the temperature was kept unchanged at 227 K,and the air pressure was evenly divided into five grades from 170 Torr to 760 Torr.According to the calculation of equations (23)–(25),it can be known that their respective threshold values of the reduced electric field are 116 Td,105 Td,98 Td,95 Td and 92.5 Td.Compared with the reduced electric field in the simulation conditions,they are,respectively,over 520%,267%,167%,110% and 73%.Five different conditions provide an environment that exceeds the electric field threshold for the development and propagation of streamers.Figures 17(a)–(e) show the distribution of the maximum electron density at different pressures at the breakdown time of the air gap.It can be seen that under the condition of constant temperature,the average electron density in the breakdown process increased with the decrease in pressure.The reason is that as the air pressure decreases,the average free path of the electron increases,and the kinetic energy of the electron under the action of the electric field increases,resulting in an increase in the probability of collision ionization,and thus more electrons are generated.Figure 17(f)is a data curve fitted by the CFTOOL in MATLAB R2018a.It can be seen that the maximum of the average electron density varies nonlinearly with the change in pressure.The number of electrons produced may depend on how much the electric field exceeds the threshold.The specific influence of the above factors on the microscopic parameters of the streamer discharge and its mechanism will be an important part of the following study.

The simulation results show that the propagation time of the double-headed streamer is only 0.7 ns at low temperature and sub-atmospheric pressure,which is much shorter than that at normal temperature and pressure.According to [29]

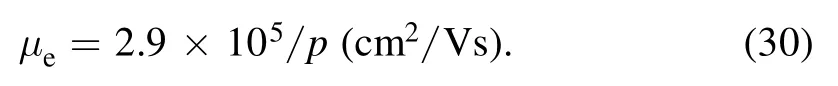
With the decrease in air pressure,the electron mobility increases gradually.Under the same applied electric field,the electron migration velocity at low temperature and subatmospheric pressure is obviously higher than that at normal temperature and pressure.As a result,the streamer propagation velocity is also faster than the normal temperature and pressure.In addition to the increase in the mean free path,the excited reaction between electrons and particles becomes more active under low temperature and sub-atmospheric pressure.Firstly,it increases the number of excited state particles.More excited groups release more photons when they transit back to the ground state,which enhances the photoionization process in the streamer head region.Secondly,the collision between excited groups is more likely to cause fractional ionization,which also leads to the increase in electron production.Finally,an active‘three-body recombination’ reaction at atmospheric pressure becomes less and less obvious as the pressure decreases,resulting in a decrease in electron consumption.In conclusion,the sum of electrons shows an increasing trend,which promotes the formation of streamer channels and shortens the time of streamer propagation.

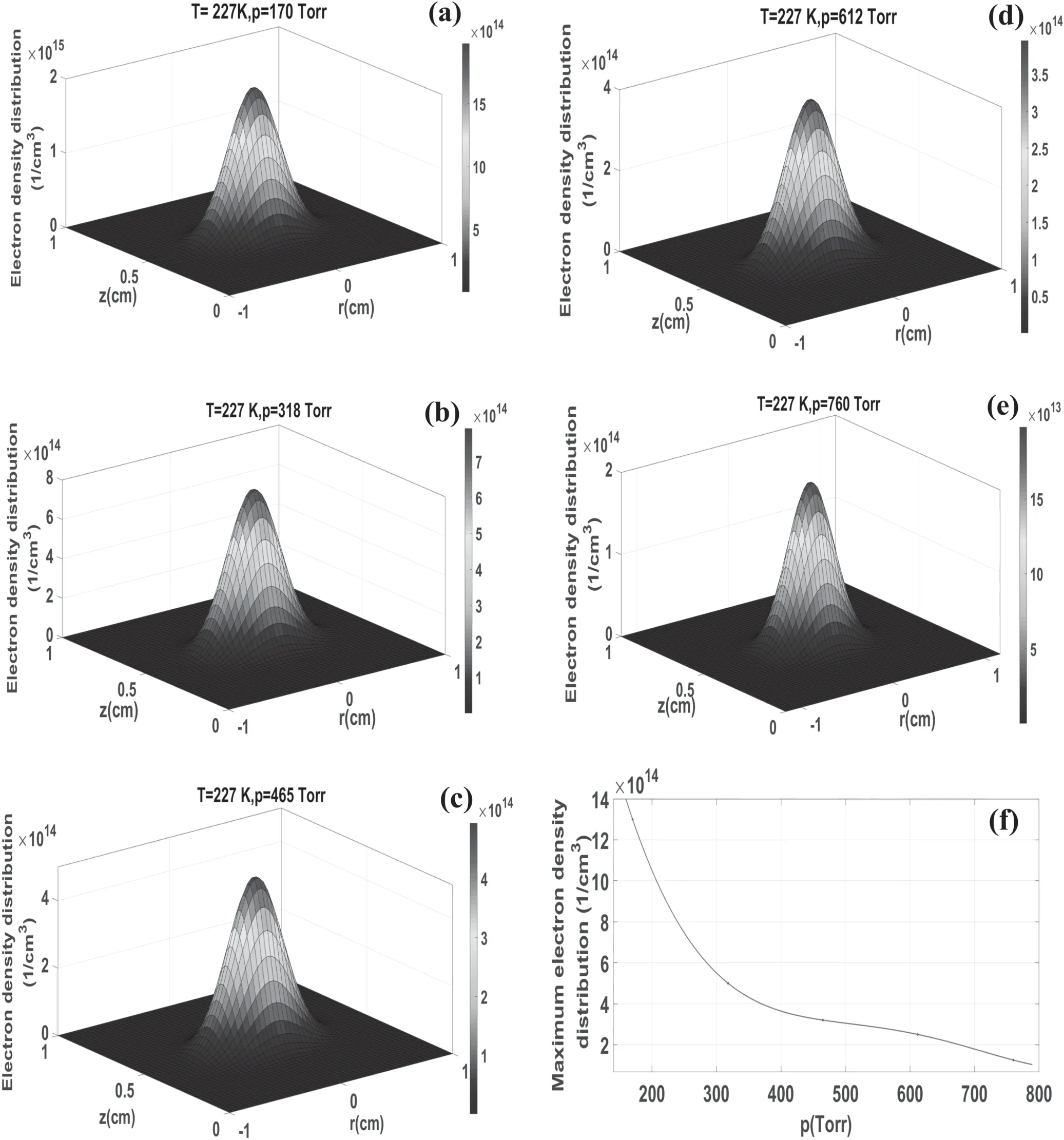
Figure 17.Electron density distribution at different pressures.(a) Electron density distribution at a temperature of 227 K and a pressure of 170 Torr.(b) Electron density distribution at a temperature of 227 K and a pressure of 318 Torr.(c) Electron density distribution at a temperature of 227 K and a pressure of 465 Torr.(d) Electron density distribution at a temperature of 227 K and a pressure of 612 Torr.(e) Electron density distribution at a temperature of 227 K and a pressure of 760 Torr.

4.Conclusions

This paper discusses several models for calculating the photoionization of plasma discharge in air,and improves the corresponding boundary conditions.Under the conditions of low temperature and sub-atmospheric pressure,the fluid equation is used to simulate the double-headed streamer propagation under DC voltage and a uniform electric field.The main conclusions are as follows:

(1) Using the six-exponential fit for the Helmholtz model to calculate the photoionization rate has the advantages of wide application range,high efficiency and good consistency with the classical integral model.The premise of using this method to replace the integral model is to have an accurate and efficient boundary condition.This paper proposes a more effective boundary condition,namely the second-order SBC.

(2) The simulation results show that under low temperature and sub-atmospheric pressure,the discharge current of the positive streamer is larger than that of the negative streamer,the photoionization rate of the streamer head is much lower than that of the collision ionization rate,the positive ion density and electron density of the positive streamer head are slightly larger than those of the negative streamer head,and the field strength of the positive and negative streamer head is enhanced due to the space charge effect.As well as the shielding effect of space charge,the internal field of the streamer is greatly weakened,and the above conclusions are consistent with those under atmospheric pressure.There is a great difference in the velocity of the streamer propagation.The main reason is that with the decrease in pressure,the mobility of electrons increases gradually,and the excited group also increases.The graded ionization reaction between the excited states is strengthened,which leads to the increase in the electronic output.The ‘three-body recombination’reaction becomes less obvious,resulting in less electron consumption.In conclusion,the sum of electrons is gradually increasing,which further promotes the formation of the streamer and shortens the time of the streamer propagation.

(3) Compared with the pressure,the change in temperature has less influence on the discharge process; therefore,this paper only discusses the characteristics of the discharge at different pressures.The results show that with the decrease in the pressure,the average electron density increased gradually in the process of breakdown; the main reason is that the increase in the mean free path leads to the increase in electron kinetic energy,and then it increases the probability of the occurrence of collision ionization,which produces more electrons.

Due to the limited time,this paper only studies the air breakdown characteristics under DC voltage and a uniform electric field.The research on the characteristics of a nonuniform electric field and different voltage sources (such as high repetition rate pulse power supply) will be reflected in the following paper.

Acknowledgments

This work is supported by the No.703 Research Institute of CSIC(China Shipbuilding Industry Corporation)and Yunnan Electric Test&Research Institute Group CO.,Ltd.The authors would like to thank Dr Lipeng LIU for helpful discussions.