Analysis of Drilling Riser in Re-entry Operation Under Complex Currents

-,-,

(1.Logistics Engineering College,Shanghai Maritime University,Shanghai 201306,China;2.School of Naval Architecture,Ocean and Civil Engineering,Shanghai Jiao Tong University,Shanghai 200240,China)

Abstract: In order to complete the operation accurately and safely, the dynamic characteristics of risers in re-entry, especially the riser deformation and the distance from lower-end to wellhead, should be studied precisely.Although the re-entry operation generally occurs when the destructive sea condition turns slightly better, there most likely exists a complex current due to the great operating water depth. However, the dynamics calculation for re-entry in previous research ignored the influence of currents. In this paper, a more accurate model is provided to describe the dynamic behavior of risers in re-entry operation considering three different currents (uniform, skewed, bilateral) that may occur in real sea-conditions.We adopted the Finite Difference Approximation (FDA)method to simplify the original partial differential equations into a linear equation set. According to the characteristics of the riser in re-entry, the special initial state and the boundary conditions are set up to make the calculation more efficient. The riser deformation (shape), horizontal distance from lower-end to wellhead and the velocity of lower-end were investigated under different currents with Newton iteration scheme. In addition, we compared the effect of different currents on the re-entry operation considering possible swings based on the velocity of lower-end.From the results,some interesting and meaningful information about the riser’s features and behaviors were obtained, which is quite useful for the riser’s control scheme constitution in its practical re-entry and also can offer reference for precise and safe operations.

Key words:riser;re-entry;complex currents;dynamics calculation;FDA;Newton iteration scheme

0 Introduction

In drilling operations, the drilling riser was a key component required to connect its bottom end to the blowout preventer at the seabed with both its top connected angle and its deformation controlled. The riser re-entry operation[1]means when the sea condition is getting worse or the complex current coming,the drilling risers will be pulled out of wellheads and moved to a safe location to avoid the destruction, while the sea condition gets better, the risers need to be moved and connected to the wellhead again. Fig.1 conceptually depicted the re-entry process of a drilling riser. The upper-end of riser is attached to the surface vessel and follows its motion, and the lower-end is completely free. Under the action of currents, the free lower-end would be positioned within the re-entry operation area above the wellhead located in the seabed though the movement of surface vessel,then the drilling riser installation begins. So,the horizontal distance from lower-end to wellhead directly affects the accuracy of re-entry operation.

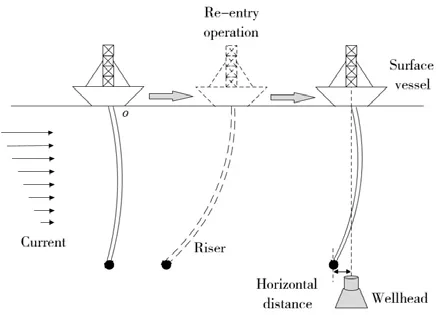
Fig.1 The re-entry process of a drilling riser

However, due to the free-hanging of lower-end, the re-entry operation of drilling riser is becoming increasingly difficult with increasing water depth and now it is one of the major operations regarding deep sea drilling because manual re-entry becomes difficult in such a water depth. In addition, influence factors, especially marine environment with non-uniform currents (shear current, stratified current), could also have significant effect on the riser in re-entry operation, hydro-dynamical drag forces and the flexibility of riser also make it more difficult for them to operate it correctly. In order to accomplish the operation correctly and safely, dynamics analysis of the riser in re-entry urgently needs to be studied to predict the riser deformation and locate the lower end.

For a long time,some studies are carried out for the free-hanging riser dynamic behaviors.Patel[2]presented a theoretical and experimental investigation of the lateral dynamics of freehanging marine risers, and the in-plane behavior had been investigated through a finite element analysis of the riser and its hydrodynamic loading.Hong[3]carried out numerical investigations on dynamics of flexible free-hanging riser with the equations of motion of riser derived based on Hamilton’s principle.Besides,Kuiper[4-5]studied the stability of a vertically suspended, fully submerged water-conveying pipe (water riser). However, for the re-entry of riser, as a particular and actual operation of free-hanging riser, there have been few study works being carried out. Suzuki[6-7]presented active control for deep-water riser’s re-entry operating systems based on previous research work about the riser,and carried out a basin experiment to investigate the coupled dynamic behaviors of the riser model.Ohtsubo[8-9]considered the entry/reentry control problem of the flexible marine riser applying LPV (Linear Parameter Varying)technique,and carried out the re-entry experiment in the towing tank to verify the results.Kajiwara[10]built and calculated the re-entry riser model considering a constant current on the upper half based on motion equation together with the mode shapes,and applied LQI(Linear Quadratic with Integral action)control methodology to obtain an appropriate re-entry controller.Recently,Wang and Xu[11]adopted specific boundary conditions to simplify the modeling of re-entry riser,and calculated the dynamics response of re-entry risers.

Although the re-entry operation generally occurs when the destructive sea condition turns slightly better,there most likely exists a complex current due to the great operating water depth in re-entry, so we cannot ignore the influence of currents. However, most of studies mentioned above did not consider current during analyzing the dynamics of re-entry riser, the hydrodynamic drag force was thought to be entirely caused by the movement of the riser, or the steady current was only considerd, it clearly does not accurately reflect the actual operation. In order to control the horizontal distance from lower-end to wellhead, studying precisely the dynamic characteristics of risers in re-entry considering actual currents needs to be carried out.

Hence, what is different from the conventional riser modeling in re-entry is that the current forces are added into the model in this work. The governing equations of re-entry riser were derived from the force equilibriums of external forces at node and an efficient time-domain analysis scheme was developed in this work based on finite difference approximation.The dynamic response of riser in re-entry was investigated considering uniform,skewed and bilateral currents. The riser deformation and the horizontal distance from lower-end to wellhead for re-entry were investigated under each current.Considering possible swings based on the velocity of lower-end, the advantages and disadvantages of different currents in re-entry operation were compared.

1 Modeling of riser

In the re-entry process, we assume that the riser is completely vertical in starting without velocity in any direction,with the upper-end hinged by a fixed connection to the surface vessel,and the riser is moved in horizontal direction,the vertical motion of riser caused by mother ship is very small, and the tension of riser is mainly caused by its self-weight. The aim of this process is to make the riser positioned accurately within the re-entry operation area above the wellhead located in the seabed.

Here,we treat the dynamic model of riser as a beam located in the vertical plane and subjected to the tension force, the gravities of the riser and the lateral force (current force), as shown in Fig.2(a). The global coordinate system is set with thex-axis parallel to the horizontal plane and thez-axis pointing to the sea floor. The local inertial coordinate system is set along the tangential direction(t)and the normal direction(n)of the riser.

Fig.2 Mechanical diagram of riser assumed(a)and schematic diagram of discrete model(b)

The analysis model is established in a local inertial coordinate system based on simple Euler-Bernoulli beam theory applying the discrete analysis scheme[12]. As shown in Fig.2(b),the riser can be divided intoNnumber of discrete rigid segments withN+1 nodes, and the length of each segment is ds(L/N),whereLis the total length of riser in the re-entry process.

Then, the differential control equations for a segment are established according to the dynamic force balance,and expressed in the tangential and normal directions,as shown in Eqs.(1-2).

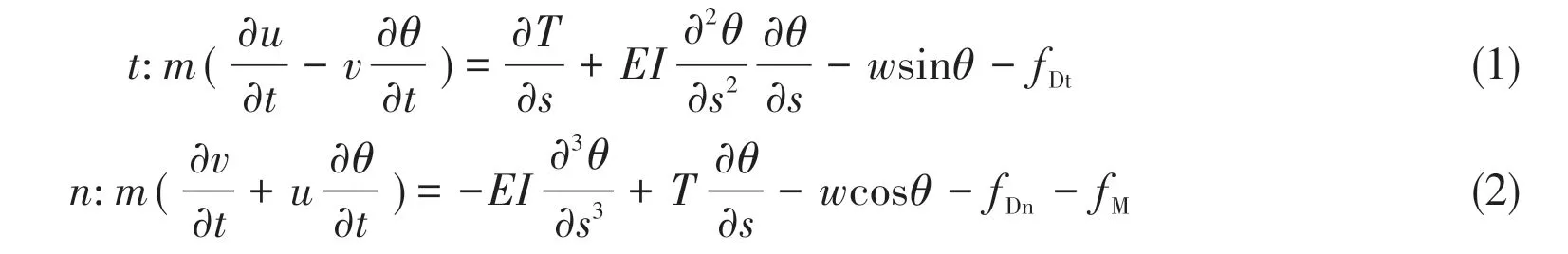
wheremis the mass of a segment,uandvare the tangential and normal velocities,respectively,Tis the dynamic tension force distribution in the tangential direction,Eis Young’s modulus,Iis the moment of inertia,wis the submerged weight of the segment,θis the angle of the node between the tangential direction and the vertical direction,fDtandfDnare the drag forces of currents in the tangential and normal directions respectively, andfMis the inertia force of currents in the normal direction.

In addition,Eq.(3)defines the tensions and shear forces.

We assume that the main external forces act on the nodes.fDtandfDnare the drag forces in the tangential and normal directions,respectively,andfMis the inertia force in the normal direction.The drag forces acting on riser is the result of motion of riser and current.

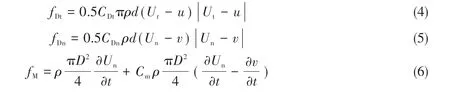
whereCDtis the drag coefficient in the tangential direction,CDnis the drag coefficient in the normal direction,ρis the density of sea water,dis the outer diameter of the riser,UtandUnare the current velocities in the tangential and normal directions respectively,andCmrefers to the inertial coefficient.

Here,u,v,UtandUnare variable in local coordinates, it is essential to simplify the representation of the results. In order to calculate expediently, their local inertial coordinates are transformed into global fixed coordinates.

whereVxandVzare velocities of node of riser in thex- andy-directions of the global coordinate system respectively, andUis the current velocity in thex-directions of the global coordinate system.

Introducing Eqs.(3-8)into Eqs.(1-2),we obtain the equation set for the dynamic motion of riser.

Due to the fixed connection of the upper-end with the surface vessel,the upper-end is determined by the motion of vessel.Here,we can assume the surface vessel only sails in thex-directions.

whereVx(1) andVz(1) represent the velocity of the first node of riser at any iteration time inxandz- directions, respectively,Pvis the velocity of surface vessel, and here it is relative to the velocity of the currents.

And,considering boundary conditions of lower-end,there is an equation as shown in Eq.(11).

whereM(n+ 1)represents the bending moment of then+1 node of riser.

2 Calculation method

To simplify the original partial differential equations to a linear equation set, a Finite Difference Approximation (FDA) method is adopted. The approximations of partial differentiations ofΘwith respect tolandtare written in Eq.(12). Then, introducing Eq.(12) into Eqs.(9-11),the linear equation set of 4N+1 nodes can be set up.

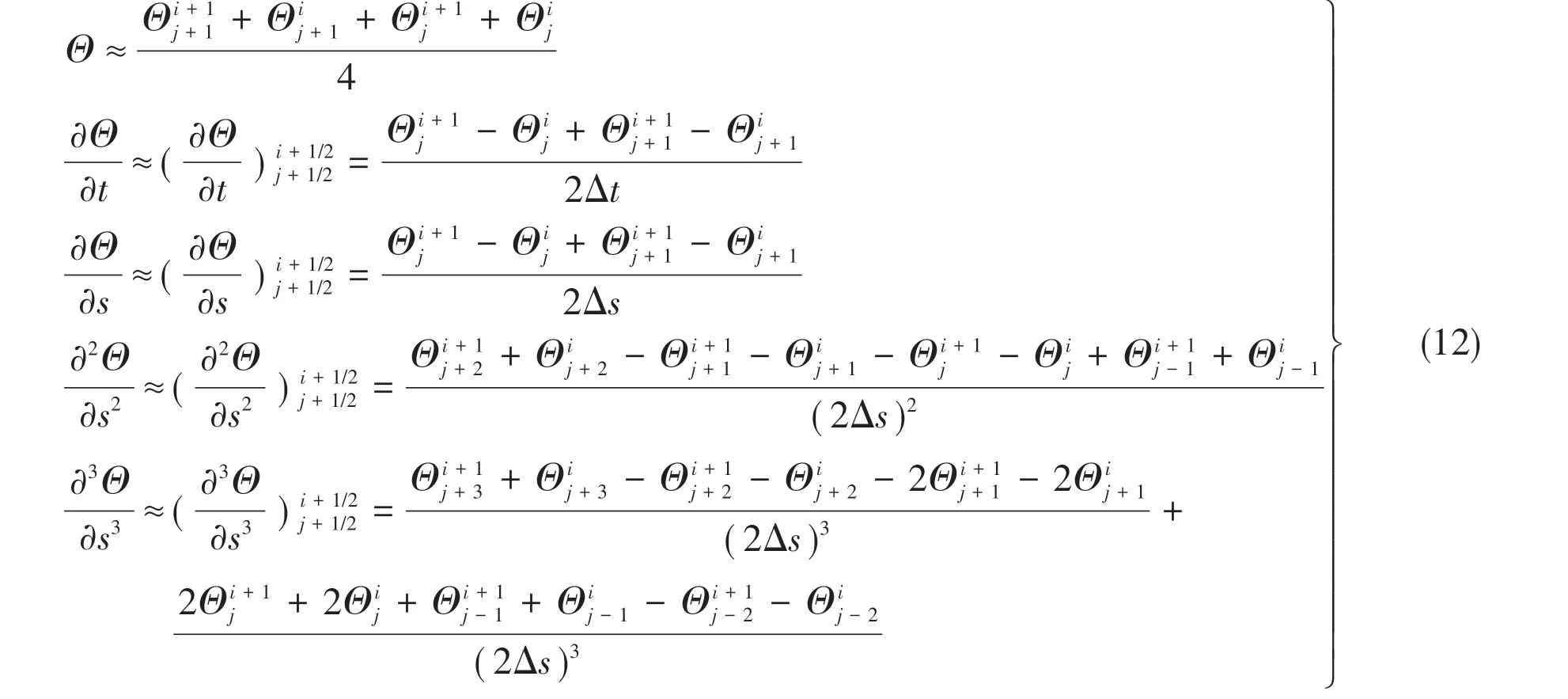
whenΘifor the iteration timetiis obtained,Θi+1for the next iteration timeti+1could be calculated using the Newton iteration algorithm.According to this theory,the initial state (i=1)needs to be set：riser is vertically located in the water without lateral displacement and vertical velocity,as followed in Eq.(13).The setting may cause a certain deviation in the initial condition,but in contrast with large calculation depth and duration,such a deviation can be neglected.

whereVx1andVz1are the velocity of each node at iteration time 1 inx-andz-directions respectively,θ1is the rotation angle of each node at iteration time 1,is the tension of nodej(j∈{1,2,3, …,N-1,N,N+1}) at iteration time 1,is the weight of segmentj(j∈{1, 2, 3, …,N-1,N})of riser at iteration time 1 andWeis the weight of equipment hanging at the lower-end of riser.

We firstly assumeΘ2=Θ1to calculateΘ2via the Newton iteration algorithm.Θidenotes an approximate value in thei-th iteration for the solution of the unknown variablesΘ, and the estimated value ofΘi+1for the initial guess is obtained withΘi：

The correction value ofΘi+1can be calculated usingΘiand the initial guess ofΘi+1：

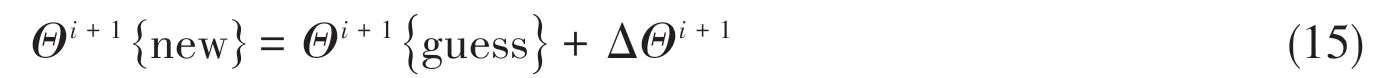
where ΔΘi+1is found by solving the linear equations.

And,Ji+1is the Jacobian matrix of equationsG,

whereG(Θi,Θi+1)=0

If the reference variableλ≤10-10, the calculation could continue to the next iteration time step(i+2).

Otherwise,the correction valueΘi+1(new)needs to beΘi+1(guess)to obtain a more accurate value via calculating Eqs.(14)-(17).

3 Simulation and results

In re-entry, the riser needs to be moved horizontally from the initial position to the target position. Now, the excellent simulation based FDA method can be done in re-entry for marine riser to predict the dynamics trajectory of riser.

3.1 An example

Taking an actual re-entry of riser as an example,a specific flexible marine riser is chosen as the simulation object, and the starting position of re-entry operation is about 135 meters from the target wellhead in horizontal direction.The riser is hinged vertically to the drilling vessel initially,and its features are shown in Tab.1

The environments are selected from the combined extreme (100 year return period) for loop currents and bottom currents, containing a combination of wind,waves and currents.Thedata provide indicative values for metocean parameters which can be suitable for conceptual studies. In re-entry analysis, the governing parameter is the current velocity profile. For this thesis, three distinctive profiles are considered： uniform, skewed and bilateral. The profiles are illustrated in Fig.3.

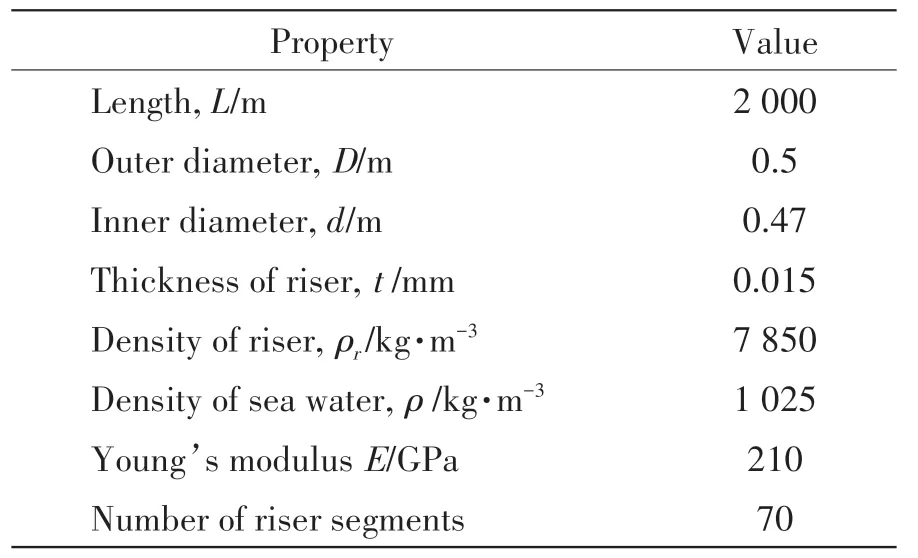
Tab.1 Properties of the marine riser

Fig.3 Current profiles used in the simulations(a：uniform;b：skewed;c：bilateral)

And these current profiles can be presented as Eqs.(19)-(21).

With the surface vessel traveling in forward direction with a prearranged velocity, the dynamic behaviors of marine riser are simulated. The vessel’s velocity (relative to the currents) and upperend’s velocity are changing as shown in Fig.4. In the initial 30 s,the vessel moves with 0.05 m/s2acceleration forward, and with the velocity from -0.5 to 1 m/s. And then, the vessel travels at a steady speed of 1 m/s for 60 s. Finally, the vessel slows down to -0.5 m/s with 0.05 m/s2deceleration for the last 30 s. Due to the relative velocityPv, the vessel remained static at 0 s and 120 s. On the side,because the upper-end’s velocity is the superposition of vessel’s velocity and current velocity, the changing trends of it are similar to those of vessel’s velocity. And the maximum velocity of upper-end runs up to 1.5 m/s in global coordinate system.

During the simulation time of 120 s, we mainly want to see the riser’s shape and the horizontal distance from lower-end to wellhead when the surface vessel travels with changing velocities.The riser is divided intoN=70 rigid segments with 71 nodes for calculations.The time intervals are chosen as Δt= 0.05 s. Although hydrodynamic coefficients are dependent on Reynolds number, Keulegan-Carpenter number and roughness, here, the values ofCDt,CDnandCmare taken as 0.01, 1.2, and 2, respectively, as suggested by Techet[13]for Morrison’s equations.Then,the numerical simulations of re-entry of riser under complex currents can be carried out.

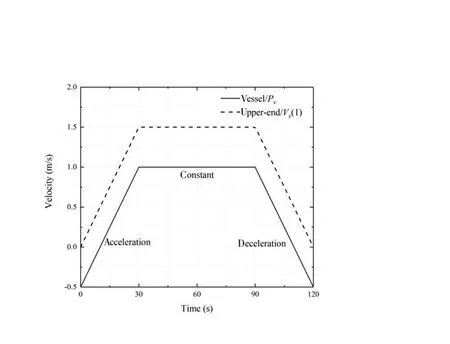
Fig.4 The time history of vessel’s and upper-end’s velocities

3.2 Riser deformation

In view of the re-entry operation of the free-hanging riser, the deformation of riser is of the utmost importance.With the upper-end movement,the smoother the riser shape,the smaller the bending deformation,not only to avoid the bending failure of the riser,but also to restore the riser as soon as possible to increase the efficiency of the re-entry process. There are three subfigures in Fig.5, respectively showing the simulation results of riser displacement over time under different currents of uniform, skewed, bilateral. There are 11 curves in each subfigure,and each curve presents the riser shape at a particular moment.The time interval between adjacent curves is determined by the movement of the surface vessel.In the first 30 s of the simulation time,the time interval is 10 s. In the ship’s uniform movement within 60 s, the time interval is 15 s.In the last 30 s,the time interval is 10 s.

From Fig.5, we can see that there is a large difference of deformation between the upperend and lower-end of the riser. The movement of lower-end was visibly lagging behind that of upper-end. The reason of motion lag may be that the length of the flexible riser was too long and the dynamic effects of the surface vessel’s movement on the lower-end were relatively small. With the increase of water depth, this lag is more obvious. In addition, we found that, in the uniform current, the deformation trend of riser is very similar in the first 90 s, following gradually change of this trend. This phenomenon also appears in the results of the skewed current and the bilateral current,which explains the deceleration movement of the vessel has a relatively large impact on the deformation of the riser.

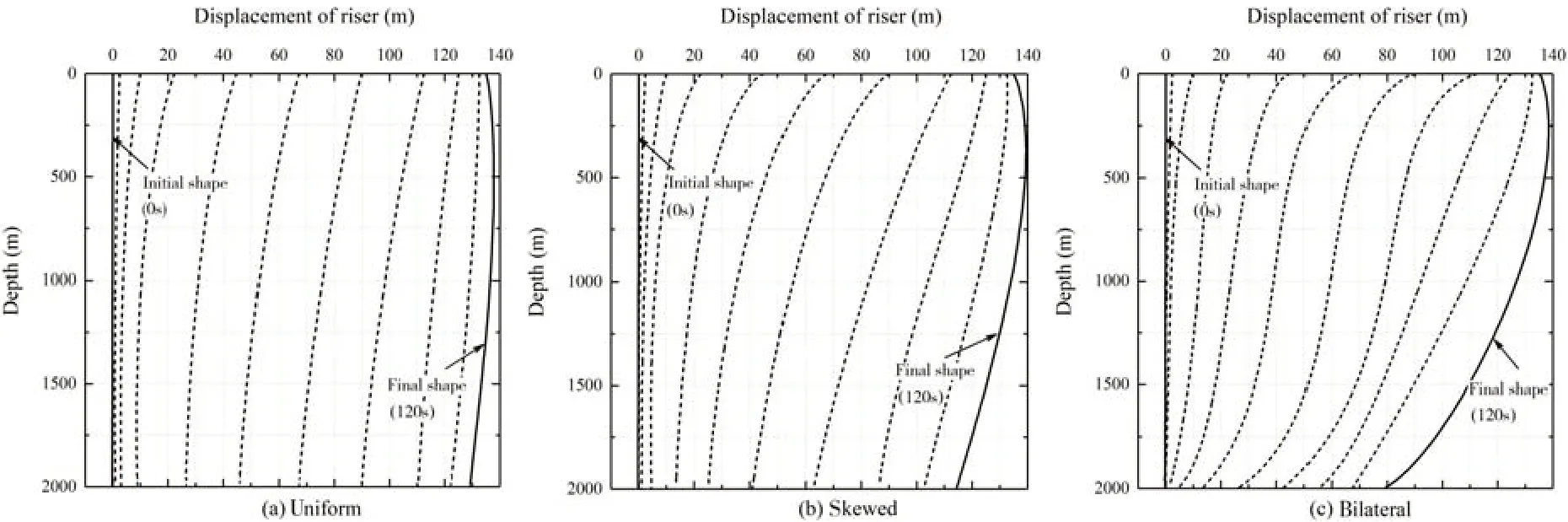
Fig.5 Riser shape over time due to three simulated current profiles

In uniform current (Fig.5a) and skewed (Fig.5b) current, the deformation trend of the riser is similar in the corresponding stage. In 0-90 s, with the increase of water depth, the left displacement of riser gets greater. And as time increases, the left displacement of the same position of the riser is also increasing. After 90 s, as the water depth increases, the riser offsets to the right first and then to the left.And as the time increases,the displacement of the same position of the riser is also increasing.In spite of these similarities,the deformation trend of the upper part of the riser is more pronounced and the bending degree is greater under the action of skewed current. However, in the bilateral current (Fig.5c), the deformation of the riser is more complicated. In the first 100 s, the shape of the riser presents‘S’. And in the first 80 s, as the time increases,‘S’shape gets more obvious. In 80 s-100 s, this trend is increasingly weakened.After 100 s,the‘S’shape of the riser completely fades away instead of a shape (offsets to the right first and then to the left) which is similar to that in the other two currents. Moreover,we found that,in the bilateral current,there is a greater displacement of the same position of riser than the other two currents, which is a result of the superposition of upper skewed current and lower skewed current.

3.3 Lower-end motion

In the re-entry operation of riser, the movement of the lower-end of the riser is more important,which directly affects the accuracy and efficiency of the re-entry process.When the upper-end of riser completes the displacement of 135 m within a specified time, the shorter the horizontal distance from the lower-end to the wellhead, the more favorable the re-entry operation of the riser. In this paper, the horizontal distance from the lower-end to the wellhead, the velocity and offset of the lower-end are analyzed and compared under three different currents respectively,as shown in Figs.6-8.

According to Figs.6 and 7,it can be seen that the movement of the lower-end is very similar under uniform and skewed currents. In the acceleration phase of surface vessel, the velocity of the lower-end has been slowly raised and the lower-end only slowly moves a little toward the wellhead (uniform： about 10 m, skewed： about 5 m), which results from the initial state of riser and the motion lag of the lower-end of riser.At the stage of uniform motion of vessel (30～90 s),the lower-end moves quickly and steadily towards the wellhead. However, the inflection point of enhancing velocity of lower-end in uniform current predates obviously the time (as shown in Fig.7), and this is mainly because the lower-end subjects to the same current force as the upper-end in uniform current so that the velocity of lower-end increases faster. In addition, we can see the difference of the inflection point of the two distance curves in Fig.6.During the deceleration phase of vessel (90～120 s), the lower-end continues to move toward the wellhead with a reduced velocity,which is caused by the motion deceleration of surface vessel.Moreover,due to the motion lag of the lower-end, the inflection point time of starting to reduce velocity is ＞90 s(uniform：about 100 s,skewed：about 110 s).

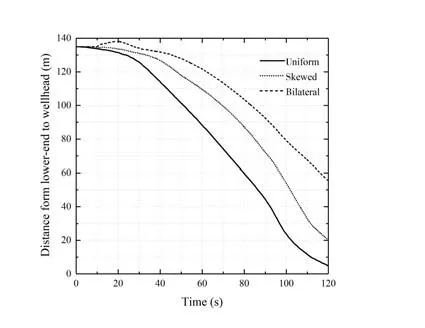
Fig.6 Distance changes

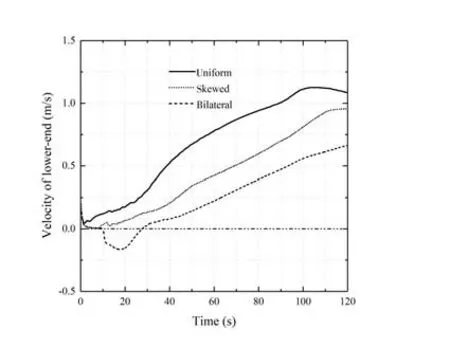
Fig.7 Velocity changes

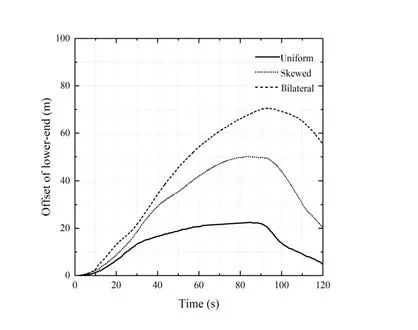
Fig.8 Offset Changes

We also found that the motion of the lower-end of riser is relatively complex due to the opposite effect in the upper and lower parts of the riser under bilateral currents.In the 30 s of accelerated motion, the lower-end moves away from the wellhead with the skewed current in the opposite direction although the upper-end moves toward the wellhead (as shown in Fig.6). The velocity of lower-end increases from 0 to 0.25 m/s,and then decreases to 0 m/s.At this point,it is just at uniform motion stage with the upper-end at a speed of 1.5 m/s.Since then,the lowerend begins to move toward the wellhead with a increasing speed.After 90 s,although the vessel has begun to slow down, there is still no significance for the lower-end to slow down under the effect of reverse current.

In addition, we can see some of the more important information. At the same time, the distances between the lower-end and the wellhead are very different under different currents. The distance in the uniform current is the smallest which is only about 6 m at 120 s, and it is the largest in the bilateral current, which reaches 55 m at 120 s. But the velocity of lower-end is the largest in the uniform current,which reaches 1.1 m/s at 120 s,and it will cause a relatively large swing at the lower-end when the vessel stops moving above the wellhead, which is very detrimental to the re-entry operation. With these two considerations combined, we believe that the skewed current is relatively suitable for the re-entry operation.

Finally, we study the offset of the lower-end to the upper-end of riser under the three currents, as shown in Fig.8. The bigger the offset, the bigger the bending degree of the riser, and the greater the bending stress on the riser,which is detrimental to the safety of the structure.By analyzing,we find a similar law for the offset of the lower-end under these currents.During the acceleration and uniform motion of the vessel, the offset at the lower-end increases with the time increased. At about 90 s, the vessel in deceleration phase, the offset curves present obvious inflection points. After that, the offset gradually reduces with the time increased. In addition, at the same time point, the offset of lower-end in bilateral current has always been relatively maximum, which reaches 70 m at 90 s. And at this time, the shape of riser is an obvious‘S’. In this case, it will cause not only relatively larger bending stress but also stronger shear stress in the middle of the riser, which is prone to cause the structure damage of riser. Therefore,we should try to avoid re-entry operation when there are bilateral currents.

4 Conclusions

Considering three different currents that may occur in real sea-conditions, an accurate model is provided to describe the dynamic behavior of risers in re-entry operation.The riser deformation (shape), horizontal distance from lower-end to wellhead and the velocity of lowerend were investigated under different currents (uniform, skewed and bilateral). Combining the horizontal distance of lower-end and the possible swings, we compared the effect of different currents on the re-entry operation, and some interesting and meaningful results were obtained that can offer references for precise and secure re-entry operation.

(1) The deceleration movement of the surface vessel has a relatively large influence on the riser deformation. After 90 s of motion deceleration stage of vessel, the shape of riser gradually changes.

(2)In uniform and skewed current,the deformation trend of the riser is similar in the corresponding stage. In 0-90 s, the left-displacement gets greater with the increase of water depth.After 90 s, the riser offsets to the right first and then to the left. However, in bilateral currents,the shape of riser shows a complicated‘S’.

(3) Although the distance from lower-end to wellhead is the smallest in the uniform current, the velocity of lower-end is the largest, which may cause a large swing at the lower-end when the vessel stops moving above the wellhead. Considering the two aspects, we believe that the skewed current is relatively suitable for the re-entry operation compared with the other two currents.

(4)The offset of lower-end to upper-end has always been relatively larger in bilateral currents,causing relatively larger bending stress and shear stress.The actual re-entry operation of riser should try to avoid the sea conditions with bilateral currents.