Study on the Perturbation Characteristics of Two-Channel Laser Propagation in Atmospheric Turbulence
2019-07-16XiaoDongYang杨晓东andMingGao高明
Xiao-Dong Yang(杨晓东)and Ming Gao(高明)
School of Optoelectronic Engineering,Xi’an Technological University,Xi’an 710021,China
AbstractIn order to study the influence factors of acquisition detection target information by lidar and understand the influence degree of each factor,the two-channel phase perturbation model and the two-channel eikonal variance model are derived in detail by using the geometrical optics method in this paper,and each factor is discussed in detail.The results show that the transmission distance is the main factor to a ffect the two-channel perturbation.With the increase of the transmission distance,the disturbing degree will gradually weaken.With the increase of transverse coordinates,the disturbing of two channels will also be weakened.In order to further weaken the disturbing degree,the feature dimension should be far larger than the wavelength,but far less than the transmission distance.
Key words:atmospheric turbulence,two-channel,phase perturbation,eikonal variance
1 Introduction
With the continuous development of detection technology,laser source has been effectively integrated into the detection device,provides the emitter with high intensity,good collimation and strong monochrome for the active detection method for active detection[1−3]and is widely used in military,transportation,agriculture,and life production.Without the influence of atmospheric turbulence,the target echo signal of the laser source[4−5]is invariable,and its value is determined by the transmitter’s emission shape and vacuum di ff raction effect,and it is easy to be observed.But in practical application,atmospheric turbulence is objective existence,and it will make the atmospheric properties change randomly and continuously,which will inevitably a ffect the signals received.Noise caused by atmospheric[6−8]will decrease the receiving performance of the system and interfere with the accuracy of signal acquisition.In remote sensing,the phenomenon of signal receiving perturbation[9]will be more obvious,so the corresponding theory must be used for in-depth study.If the emission and observation processes of optical distance are discussed separately,it is obvious that there will be a certain difference due to the real-time variation of turbulence.Light beams transfer twice in the same inhomogeneous medium,resulting in two-channel effect.[10−13]Two-channel effect should be studied in the same instantaneous medium parameters,which will effectively improve the detection accuracy,reduce the fl uctuation error of atmospheric turbulence,and provide a strong guarantee for accurate acquisition of target information.
In order to solve the problem of laser transmission and reception in medium with large-scale random inhomogeneity,statistical concepts need to be introduced,a simple and intuitive geometrical optical method(GOM)is adopted,[14−16]and large angle scatter and di ff raction phenomena are ignored.This method can analyze many problems which are difficult to be solved and described directly by other technologies,and some inference results even exceed the validity range with great advantages in the study of optical propagation characteristics.
2 Method
Starting with scalar monochrome light wave,the dielectric constant of transmission is derived from Helmholtz equation.

We can write plane waves as

where phase Φ =kφ,k as the beam, φ is an eikonal function.In turbulent atmosphere,a is expanded into reciprocal series of beams.[17]

In the extension of the above formula,item m is very complex and its value is very weak.Substitute Eq.(3)into Eq.(1)

It is called the eikonal equation.In a turbulent atmosphere,the dielectric constant can be expressed statisti-is the average part,is the fl uctuation part.Generally,the fl uctuations are much less than the average.The eikonal equation is similarly expressed as

Assume that the first term satisfies the eikonal equation without perturbation

Substituting Eqs.(5)and(6)into Eq.(4),the following linear equation will be obtained
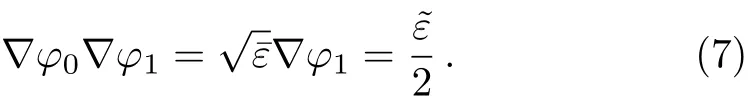
The phase perturbation a ffected by˜ϵ is obtained.
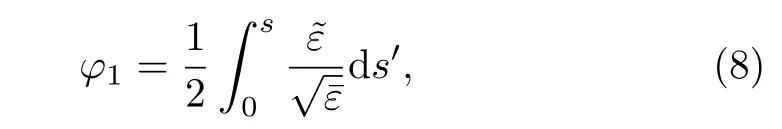
where,s is propagation path of ray.If the plane wave in the propagation process is1 of the statistical uniform medium,the covariance of the eikonal function is

where,rrr111=(ϱ1,z1),rrr222=(ϱ2,z2)is the radius vector of the observation point.Integrate the propagation path by Eq.(9),and

where,z is the propagation position, ϱ and ς are horizontal coordinates and vertical coordinates,respectively.The above equation can also be expressed in the form of spatial frequency spectrum

where,k1and k2are the normal and tangential components of wave numbers k.For ς integral and

In an isotropic homogeneous medium, Φε(kkk111,0)=Equation(12)is put into the polar coordinate system and the angle is integrated,and
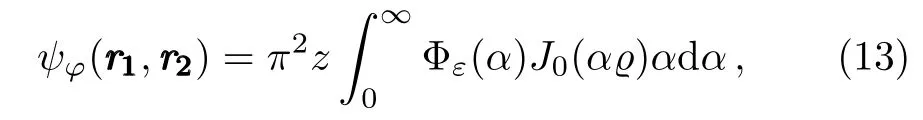
where,α is the angular coordinates of the polar coordinate system,J0(αϱ)is a zero order Bessel function.Eikonal variance σ2φwill vary with propagation distance z
It can be seen that the eikonal equation increases in direct proportion to the propagation distance.Moreover,equation function covarianceis the product of medium fl uctuation variance and effective integral correlation radius lef,and

where,Kε(ϱ,ς)is the correlation coefficient.Combining with Eq.(14)

The Gaussian distribution of the covariance of the dielectric constant is

Thus,the covariance of the Gaussian beam is
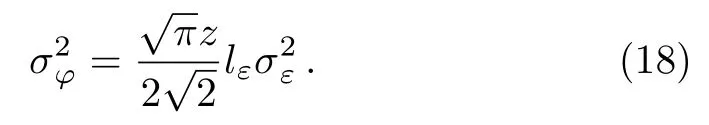
If the one-way distance that the ray passes through a random heterogeneous medium is L,ϱ can be regarded as angular coordinates.As shown in Fig.1,

Fig.1 Two-channel propagation diagram of ray through random inhomogeneous medium.
As can be seen from the fi gure,the target re fl ection point T is located at 0 point in the ordinate.When the launch point R and the observation point A are L ≫ ϱ,it can be regarded as the paraxial condition,and is symmetric about the z-axis propagation,then the covariance of dielectric constant obtained from z=L is

Combined with Eqs.(10)and(17),the covariance of the dielectric constant of the Gaussian beam is obtained as follows.


In the same way,the phase perturbation of the return path is obtained

Then two-channel phase perturbation is expressed by

In L ≫ lε,the two-channel statistical average eikonal equation of equation is If ϱ=0,the observation point coincides with the transmitting point,and


Substitute Eq.(20)into Eq.(24)to obtain the variance of Gaussian two-channel eikonal function

When the propagation distance is assumed to be a certain value,it can be observed that the phase perturbation degree of the two channels is a ffected by the transverse coordinates,as shown in Fig.2.The results show that when the transverse coordinate is smaller,the phase perturbation of the two-channel transmission increases rapidly and reaches a peak.As the transverse coordinates continue to increase,the phase perturbation begins to decrease smoothly and eventually tends to a statistical invariant.

Fig.2 The influence curve graph of horizontal coordinates of two-channel transmission on phase perturbation.
This shows that when the horizontal coordinate is large enough,the phase of the two-channel transmission presents a statistical stationary state,which can be expressed by a constant value.This will provide favorable conditions for space interference in atmospheric turbulence.
By taking three different values of transmission,respectively,it can be observed that the phase fl uctuation of two-channel transmission is a ffected by the transmission distance,as shown in Fig.3.
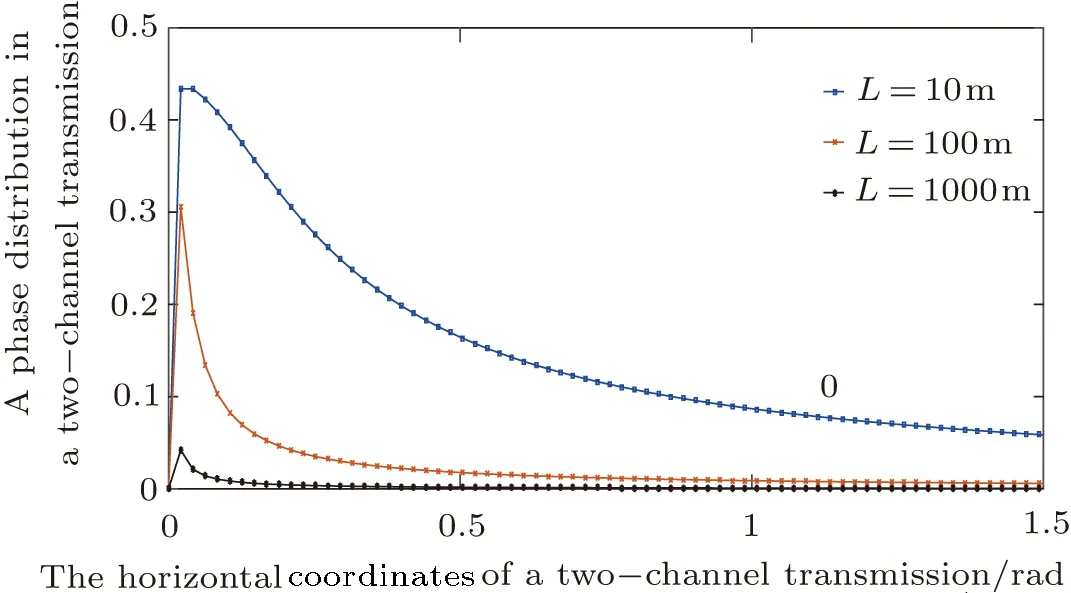
Fig.3 influence comparison diagram of different transmission distances on phase perturbation of two-channel transmission.
Thus,it can be seen that when the horizontal coordinates are the same,the smaller the transmission distance is,the larger the phase perturbation of the dual-channel transmission will be,and the opposite will be slower.Each transmission distance eventually tends to a relatively stable phase perturbation state.And on the whole,under the same conditions,if the transmission distance is small,the perturbation will be relatively obvious.When the transmission distance remains unchanged,the relationship between feature dimension and the eikonal variance of twochannel can be obtained,as shown in Fig.4.
As can be seen from the fi gure above,the feature dimension has an obvious variance increase trend near zero value,and finally,will tend to increase in direct proportion.Obviously,as the feature dimension increases contentiously,the variance of the two-channel eikonal will also continue to increase,so in the practical study of the twochannel,the feature dimension should be taken as an appropriate value.In single channel transmission,the feature dimension takes the value much larger than the wavelength value,which should also be taken here.However,it does not mean that the larger the value,the better.In the area of excessive strong fl uctuation(near zero),the value of feature dimension should be taken smaller as far as possible.
When the control feature dimension remains unchanged,the relationship between the laser transmission distance and the eikonal variance of the two-channel is studied,as shown in Fig.5.

Fig.5 The illustrative diagram of transmission distance effect on the eikonal variance of two-channel.

Fig.6 Illustrative diagram of the transmission distance and feature dimension comprehensive effect on the eikonal variance of two-channel.
As can be seen from the fi gure above,when the transmission distance is relatively short,the eikonal of the twochannel fl uctuates obviously,and the peak appears multiple times.However,as the transmission distance increases continuously,the variance will be gradually fl at,and the final region value will be very small.Therefore,in the study of laser close distance,the influence of atmospheric fl uctuation on the beam is relatively obvious.
In the actual transmission,the two-channel eikonal variance will be a ffected by both of the feature dimension and the transmission distance,as shown in Fig.6.
It can be seen that,although the feature dimension also has an impact on the two-channel eikonal variance,if the transmission distance is long enough,the value of the variance will trend to be stable and is very small.This indicates that the transmission distance has a more obvious influence on the two-channel variance on the two influence factors.Figure 7(a)shows the initial image acquired by the lidar.[18−19]When the target is acquired at different distances,different target contour extraction can be obtained.Figures 7(b),7(c),and 7(d)successively increase the detection distance.Obviously,the larger the visible distance is,the lower the influence on image extraction information will be.It shows that the statistical average value of air disturbance has a relatively small effect on long-distance,but relatively obvious effect on shortdistance.

Fig.7 Target Extraction of Lidar at different Distances.
3 Conclusion
In this paper,the influence of horizontal coordinates,feature dimension,and transmission distance on the twochannel laser transmission perturbation is studied.The results show that the larger the horizontal coordinate is,the smaller the overall influence fl uctuation is.The longer the transmission distance,the smaller the fl uctuation of the influence on the two-channel transmission.The selection of feature dimension should be much larger than the wave length but should be as small as possible after exceeding the strong fl uctuation region,so as to reduce the influence on the transmission fl uctuation degree.Based on overall consideration of the three factors,the transmission distance is the most important factor a ffecting the fl uctuation degree of two-channel transmission.Increasing the transmission distance is the most obvious way to reduce the fl uctuation of two-channel transmission.
In practical application,due to consider the absorption and scattering of beam energy by atmospheric,the greater the transmission distance is,the greater the loss of light energy is,and the worse the quality of the echo signal is obtained.A balance point should be found between the two.
In the future work,we will further study the problem of laser transmission,take into account the comprehensive factors of aerosol and atmospheric turbulence,and provide more reliable theoretical basis for dual-channel transmission and lidar detection.
猜你喜欢
——辨析聪明、精明、高明、英明
杂志排行
Communications in Theoretical Physics的其它文章
- Efficient Network Dismantling via Node Explosive Percolation∗
- Insights into the Discrepancy between Single Molecule Experiments∗
- effects of Inhibitory Signal on Criticality in Excitatory-Inhibitory Networks∗
- Controlling Airy-Bessel Light Bullets in an Optically Induced Potential∗
- Relativistic Self-Focusing of Hermite-cosine-Gaussian Laser Beam in Collisionless Plasma with Exponential Density Transition
- Dipole and Combo Optical Solitons in Birefringent Fibers in the Presence of Four-Wave Mixing
