Cavity enhanced measurement of trap frequency in an optical dipole trap∗

Peng-Fei Yang(杨鹏飞),Hai He(贺海)Zhi-Hui Wang(王志辉)Xing Han(韩星)Gang Li(李刚)2,†,Peng-Fei Zhang(张鹏飞)2,and Tian-Cai Zhang(张天才)2,‡

1State Key Laboratory of Quantum Optics and Quantum Optics Devices,Institute of Opto-Electronics,Shanxi University,Taiyuan 030006,China

2Collaborative Innovation Center of Extreme Optics,Shanxi University,Taiyuan 030006,China

1.Introduction

Optical dipole traps(ODT)have become practical tools in experimental realization of qubit manipulation between single photons and single atoms,which can be used to store and process the quantum information locally for quantum computation.[1]In a far-off-resonance optical trap(FORT),where the heating due to spontaneous scattering forces is strongly suppressed,[2]one can not only realize a long-time trapping of atoms but also manipulate the atomic internal state[3]with long coherent time,[4]and characterize the quantum state of a single atom[5]that is treated as a well-prepared quantum qubit.In particular,by using a tightly focused FORT,degenerate Raman sideband cooling of trapped atoms[6]and transfer of ultra-cold atoms[7–10]over macroscopic distances have been carried out.During the transfer,specific discrete transport durations are exhibited that are largely dependent on oscillation frequency of atomic ensembles in an ODT with no excitation of the vibration and no losses after transport.[10]To this end,researchers attempted to determine the trapping frequency in the experiment and clarified the dynamics of cold atoms in an ODT.The vibration mode of cold atoms inside ODT or lattices has been investigated[11–14]with an auxiliary probe beam applied to measure the fluorescence.However,this approach must induce some extra uncertainties,such as the spatial profile of the probe beam,and the intensity and the pointing fluctuations of the probe beam.[15]In addition,in some situations where a finite interspace exists,such as a cavity quantum electrodynamics(QED)system,imaging optics with a large numerical aperture to collect the fluorescence usually make the system complex.

A strongly coupled cavity QED system,even in the intermediate coupling regime,is very sensitive to atoms on the single-particle level.Such a system enables not only a succession of experiments of quantum information processing[16–18]and a nonlinear process[19]with single atoms,but also atomic sensing.[15,20–23]In a cavity QED system,the vacuum Rabi splitting is approximately pr√oportional to the square root of the effective average number(N)of atoms interacting with the cavity.[24,25]The number of coupling atoms alters the transmission of a weak resonant probe beam from the cavity.In this process,the atoms are barely excited.This provide us a way to directly measure the dynamic of atom loss or movement in the cavity without the atom fluorescence.In this Letter,we present a method based on a high- finesse optical cavity in a cavity-QED system to measure the trap frequency of cold atoms trapped in an ODT.In particular,this approach sheds fluorescence detection from the atom cloud and the imaging optics in traditional measurements.

2.Experimental setup

Figure 1 shows the scheme of our experimental system.The system consists of a vertically located high- finesse Fabry–Prot cavity with a length of 752µm and a TEM00mode with a waist of 40.7 µm(x–y plane),an ODT beam(y direction)perpendicular to the cavity axis(z direction),and a single photon counting module(SPCM).The characteristic cavity QED parameters are(g0,κ,γ)=2π × (4.7,6.6,2.6)MHz,where g0is the peak atom–cavity coupling coefficient for the133Cs 6S1/2|F=4,mF=4i↔6P3/2|F0=5,mF=5i transition,κ the cavity field decay rate and γ the atomic polarization decay rate.Cold atoms are initially captured by a magneto–optical trap(MOT)from the background vapor,the atom sample is located 7.4 mm beside the cavity mode.After a 5-ms polarization gradient cooling(PGC)phase,the cold atoms are loaded into the an ODT whose trap center is overlapped with the atoms.The ODT is formed by a tightly focused Gaussian laser beam at 1064 nm with a waist of 20µm and a power of 2 W,resulting in a potential depth of−0.74 mK and an average ac Stark shift of 16 MHz.Subsequently the loaded atoms are transported into the cavity mode with a displacement of 7.4 mm by an air-bearing translation stage(Aerotech ABL10150)operated at a maximum half-sine acceleration of 235.5 mm/s2and a maximum velocity of 20 mm/s.The transferring efficiency is approximately 83%at 200µK which can be enhanced over 90%after PGC phase.The cavity is stabilized via an auxiliary diode laser at 828 nm so that one of its longitudinal mode is resonant with the atomic transition F=4↔F0=5.A weak resonant beam with average 1 intracavity photon is adopted to probe the cavity.The transmission of the probe beam is detected by single-photon detector(SPCM in Fig.1).

Fig.1.Schematic diagram of the experimental setup.A MOT is located approximately 7.4 mm beside the cavity mode(vertical).Atoms can be conveyed into the cavity mode by an air-bearing translation stage where an ODT located.The waist of ODT is aligned with both MOT and cavity mode to ensure the successive of transport and measurement.We obtain the transmission spectra and vibration frequencies with a weak probe beam along the axis of the cavity and a single photon counting module(SPCM)applied.

3.Measurement of the oscillation frequency

The atomic sample is prepared in 6S1/2F=4 before the transportation.However,a second state preparing process after the transportation is necessary to pump atoms which decayed into the 6S1/2F=3 state during the transporting back to 6S1/2F=4 state.From the transmitting spectra,we can get the effective number of atoms which are strongly coupled to the cavity.Figure 2 shows the typical transmitting spectra with(red data points)and without(black data points)the atoms.A characteristic vacuum Rabi splitting of 94 MHz with a significant drop to almost zero at the resonance of the empty cavity indicates the effective atom number is 100±2 after the transportation.The green and blue curves are theoretical fittings.There is a detuning of−16±0.2 MHz between cavity and atom,resulting from the ac Stark shift of ODT as mentioned before,can be extracted from the spectra.

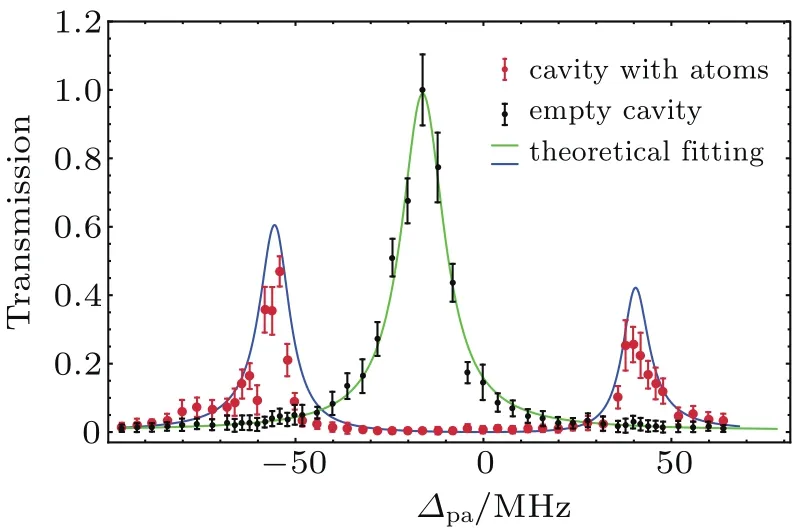
Fig.2.Transmission spectra of the cavity that show the vacuum Rabi splitting.The black dots(red dots)are the experimental data for empty cavity(atoms in cavity mode)and the green(blue)curve is the theoretical fitting.The cavity decay rate is κ =2π ×(6.6±0.2)MHz.There are approximately 100(±2)atoms transported inside the cavity with a Rabi splitting of 94 MHz.The asymmetry of splitting peaks attributes to a red detuning(−16±0.8 MHz)between cavity and atoms caused by ODT.Each point of the data is an average over 15 complete measurements obtained within 150µs.

The ODT can be approximately considered a harmonic potential.The oscillation frequencies of trapped atoms in both radial and axial directions are given[26]

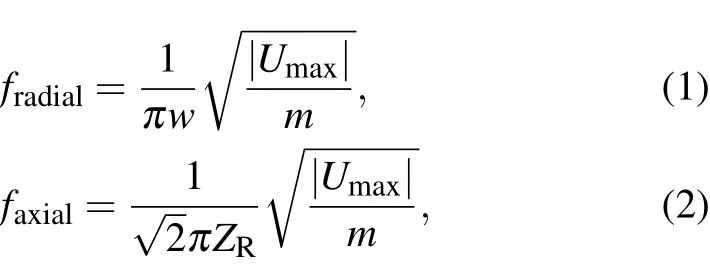
where w,ZR,and λ indicate the waist,Rayleigh length,and wavelength of the ODT beam,respectively,while|Umax|is the depth of the potential trap and m the mass of one Cs atom.If we take the beam quality factor(M2factor)into account,the Rayleigh connected with the waist by ZR= πw2/(M2λ).[27]In our experiment the trap light beam has a mode waist w=20µm and the beam quality factor M2=1.2,we can get the theoretical oscillation frequencies fradial=3.4 kHz and faxial=40.8 Hz.Regarding a resonant and parametric excitation scheme,[14,28,29]the depth modulation of the potential at trapping and double trapping frequencies results in atom heating and losses.[13,14,30]The transmission of the probe beam will change accordingly and then the trapping frequency could be determined by the transmitting spectra.

In our experiment the amplitude modulation is realized by an acousto-optic modulator(AOM)and the cavity is tuned and resonant to the transition of the trapped atoms,i.e.,the ac Stark shift due to the trap is considered.The observations of both radial and axial excitation are presented in Fig.3.The increase of transmission from cavity results from the losses of atoms in the ODT at the trapping and parametric excitation frequencies are observed.The red dots in Fig.3(a)indicate the normalized transmission spectra versus modulation frequency,and the blue curve is a Lorentzian fitting.The black dots correspond to the results without amplitude modulation.There are clearly two peaks at 2.76±0.01 kHz and 5.58±0.02 kHz corresponding to the direct trapping and parametric resonance frequencies[12]in the radial direction.

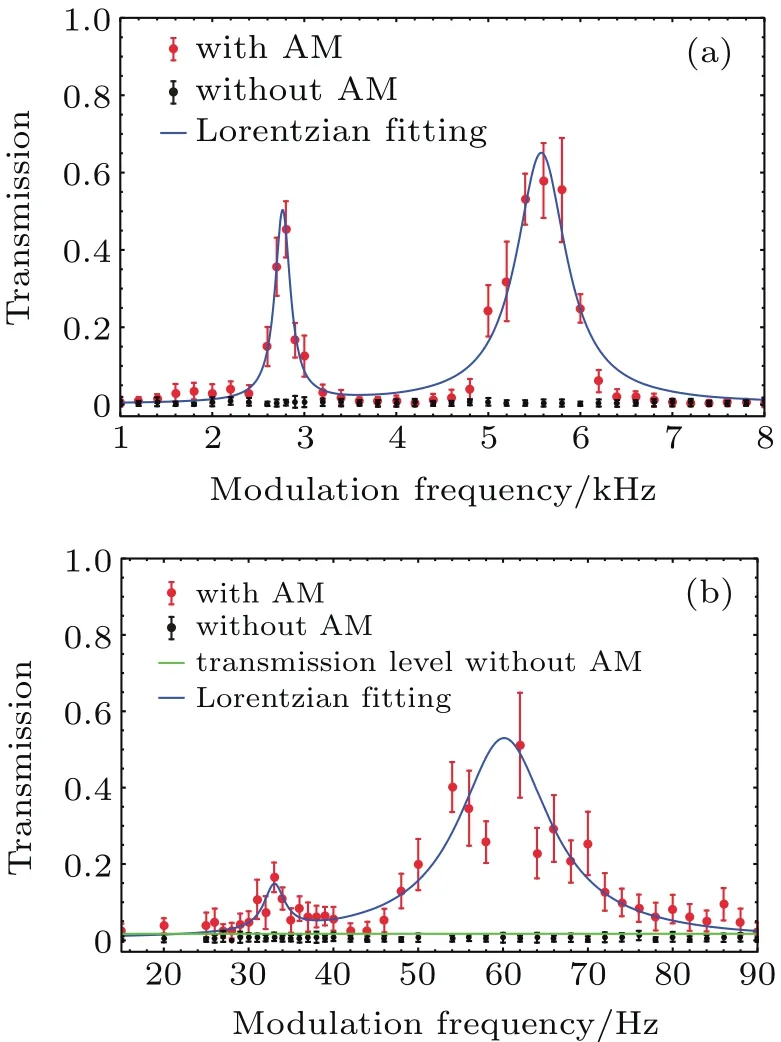
Fig.3.Transmission spectra of the cavity when AM of the ODT is applied.The red dots indicate transmission of the cavity(normalized)versus frequency of AM with a depth of 50%.The blue curve is a Lorentzian fitting.The black dots correspond to the results without AM.Panel(a)is for radial direction and panel(b)is the axial direction.Two peaks at 2.76±0.01 kHz and 5.58±0.02 kHz in panel(a)and 33.0±0.8 Hz and 60.1±0.6 Hz in panel(b)correspond to the trap frequency and its second harmonic in radial and axial directions,respectively.The green line in panel(b)shows the measured level of transmission without an AM.

Similarly,we obtain parametric heating spectra for axial atomic oscillation,as shown in Fig.3(b).The peaks at 33.0±0.8 Hz and 60.1±0.6 Hz correspond to the trap frequency and its second harmonic value in this direction.The confinement in the axial direction is weakened by a factor of πw/λ (see relations(1)and(2))of that of the radial direction,which results in a quite low trap frequency.In order to perform the parametric excitation,an AM is applied for 200 ms.The green line shows the measured level of transmission without an AM,which verifies that the transmission is dramatically altered by the AM rather than the loss of atoms in a static ODT within 200 ms.All data above are obtained under the circumstance that a fixed modulation depth of 50%is imposed.

The measured oscillation frequencies 2.76±0.01 kHz and 33.0±0.8 Hz are a little smaller than the theoretical expectations 3.4 kHz and 40.8 Hz.The discrepancy mainly results from the following facts.The presumption of a harmonic potential trap is not perfectly reasonable in our case,because the presumption of a harmonic potential trap is valid only under the condition when the atom temperature much lower than the trap depth.In our experiment the temperature of the atom is 40µK before the transfer,the temperature would be higher after the atom been transferred into the cavity,whereas the trap depth is 740µK.The oscillation frequency of hotter atoms is smaller than that of the atoms with lower temperature.Thus,the atomic vibrational state is more like to be excited with AM frequencies smaller than the harmonic one.[11]In Fig.3(b)the harmonic frequency measured is a little lower than the theoretical one.The reason comes from a power drift of ODT beam about 8%lower(in Fig.3(b))during the measurement.This can be avoided by stabilizing the power of ODT.

4.Intuitive observation of the oscillation frequency in axial direction

Benefiting from the fluorescence-free process,the atom number and state do not change in principle when interacting with cavity.The coupling strength g is position-dependent due to the cavity mode distribution,so the system provides a good way to measure the motion of the atom.The respond time of the cavity probe beam to the coupled atom is much faster than the conventional fluorescence-dependent detection method.The cavity QED system can also be used to continuous observe the atom movement in the cavity mode.Thus,the oscillation of the atom in the ODT in our experiment can be directly measured.The shape of cold atoms in our running wave ODT is known as a “cigar-shaped”cloud.A near-resonant intense pulse laser(x direction in Fig.1)orthogonal to both the axis of the cavity and direction of the ODT beam with a waist of 20 µm is shining to the center of the “cigar-shaped”cloud for 12.5 ms.A sufficient separation of the atom sample about 40µm appears between these two surviving atomic clouds.The waist of the near-resonant pulse laser is optimally overlapped with that of both the cavity mode and ODT beam,so the interspace on the same order as the waist of the cavity mode is initially located in the cavity mode,and these two separate parts remaining at both ends of the “cigar”will oscillate around the center of the trap after the near-resonant intense laser is switched off.The power of the ODT beam for this measurement is 2.2 W and the same waist as used above with a corresponding trap frequency and its second harmonic of 37.4 Hz and 74.8 Hz in the axial direction,respectively.As expected,a continuous oscillation feature shows up in the transmission spectrum(as shown in Fig.4)which reflects the two parts of the atom cloud oscillate in the ODT.A frequency of 73.5±0.6 Hz that corresponds to twice the trap frequency in the axial direction is extracted from the transmission spectrum.An exponential increase of the spectrum indicates a decay of atomic number in the ODT.The differences between the experimental result here and the calculation are mainly attributed to the following:(i)The ODT that we applied is not a perfect harmonic well,and(ii)as the intensity of the ODT beam is not stabilized,a slight slow drift in ODT power should be considered.

Fig.4.Transmission spectrum of the cavity when the cloud of atoms in the ODT is split into two parts.A near resonant intense pulse laser for 12.5 ms is used to split the atomic cloud into two parts with a separation of about 40µm.The red dots denote normalized transmission spectrum counted by a SPCM for 10µs for each point.The blue curve is a sinusoidal fitting of the data.A frequency of 73.5±0.6 Hz that corresponds to twice the trap frequency in the axial direction is extracted from the transmission spectrum.An exponential increase of the spectrum indicates a decay of atomic number.

Utilizing the proposed fluorescence-free detection method on the single atom level,we provided a method to determine the trap frequency of the cold atoms in an optical trap.The method avoids uncertainties in the usual measurement in which the fluorescence light is scattered by the atoms.Compared to these methods,the cavity-enhanced approach is extremely sensitive to atoms.One does not have to repeat the measurement many times,especially if the number of atoms in the ODT is small,even on the single-particle level.When the confinement is weak,or the trap frequency is low,such as several hertz,the traditional approach becomes ineffective owing to the tremendous loss of atoms during a long-playing AM procedure.[13]However,for a lower frequency in the axial direction,the advantage of the proposed system makes it easy to obtain the oscillation signal,as shown in Fig.4.These results enable us to optimize the transport of single atoms[31–33]without excitation of the vibration after transport.This makes it possible to monitor the vibration of a single atom in a single site of lattices located inside a cavity.In addition,the proposed method can be extended to record the interference signal from Bose–Einstein condensates(BEC),[34]in which the intensity of the condensate is modulated by the interference pattern,and the change of atomic vibration that heralds the formation of molecules in the preparation of Cs macrodimer molecules[35]can be detected.Moreover,this creates the possibility of determining the motion of a single atom with a stored quantum bit when keeping the atomic excitation quite low[36]with an average intracavity photon number less than 1.

5.Conclusion

In conclusion,we have presented a new cavity-enhanced method to measure the oscillation frequency of cold atoms in an ODT based on a strongly coupled cavity QED system.By modulating the intensity of the ODT beam and recording the transmission spectra of the cavity we get the oscillation frequencies of the trapped atoms with 2.76±0.01 kHz and 33.0±0.8 Hz,which correspond to the oscillation frequencies in the radial and axial directions of the ODT beam,respectively.We also provide a direct observation method for the motion of cold atoms in an ODT.By splitting the atom cloud,we get a transmission spectrum with an oscillation frequency of 73.5±0.6 Hz that corresponds to twice the trap frequency in the axial direction.Although it is difficult to eliminate the fluctuation of the average number of atoms for each transport,only the widths of the spectra are affected,while the frequencies of both resonant and parametric excitation are closely intensity dependent,and,in principle,the drift of which can be eliminated by using a servo technique.[37]Being compared to the conventional fluorescence detection,our approach avoids uncertainties associated with the illuminating light and auxiliary imaging optics.At the same time,this method enables us,simply and quickly,to obtain the most promising parameters for optimally transporting cold atoms and trapping atoms in cavity mode,[38]to determine the temperature of a single atom,[39]and to control the motion of cold atoms.

[1]Xia T,Lichtman M,Maller K,Carr A W,Piotrowicz M J,Isenhower L and Saffman M 2015 Phys.Rev.Lett.114 100503

[2]Miller J D,Cline R A and Heinzen D J 1993 Phys.Rev.A 47 R4567

[3]Wang Z H,Tian Y L,Yang C,Zhang P F,Li G and Zhang T C 2016 Phys.Rev.A 94 062124

[4]Yang J H,He X D,Guo R J,Xu P,Wang K P,Sheng C,Liu M,Wang J,Derevianko A and Zhan M S 2016 Phys.Rev.Lett.117 123201

[5]Tian Y L,Wang Z H,Zhang P F,Li G,Li J and Zhang T C 2018 Phys.Rev.A 97 013840

[6]Kaufman A M,Lester B J and Regal C A 2012 Phys.Rev.X 2 041014

[7]Kuhr S,Alt W,Schrader D,Dotsenko I,Miroshnychenko Y,Rosenfeld W,Khudaverdyan M,Gomer V,Rauschenbeutel A and Meschede D 2003 Phys.Rev.Lett.91 213002

[8]Thomas M,Stephan F,Christian L and Uwe S 2012 New J.Phys.14 073020

[9]Gustavson T L,Chikkatur A P,Leanhardt A E,Görlitz A,Gupta S,Pritchard D E and Ketterle W 2001 Phys.Rev.Lett.88 020401

[10]Couvert A,Kawalec T,Reinaudi G and Gu´ery-Odelin D 2008 Europhys.Lett.83 13001

[11]J´auregui R,Poli N,Roati G and Modugno G 2001 Phys.Rev.A 64 033403

[12]Alt W,Schrader D,Kuhr S,Müller M,Gomer V and Meschede D 2003 Phys.Rev.A 67 033403

[13]Poli N,Brecha R J,Roati G and Modugno G 2002 Phys.Rev.A 65 021401(R)

[14]Friebel S,Andrea C D,Walz J,Weitz M and H¨ansch T W 1998 Phys.Rev.A 57 R20

[15]Li W F,Du J J,Wen R J,Yang P F,Li G,Liang J J and Zhang T C 2014 Appl.Phys.Lett.104 113102

[16]NöllekeC,NeuznerA,ReisererA,HahnC,RempeGandRitterS2013 Phys.Rev.Lett.110 140403

[17]Specht H P,Nolleke C,Reiserer A,Uphoff M,Figueroa E,Ritter S and Rempe G 2011 Nature 473 190

[18]Ritter S,Nolleke C,Hahn C,Reiserer A,Neuzner A,Uphoff M,Mucke M,Figueroa E,Bochmann J and Rempe G 2012 Nature 484 195

[19]Ritter S,Nlleke C,Hahn C,Reiserer A,Neuzner A,Uphoff M,Mcke M,Figueroa E,Bochmann J and Rempe G 2010 Nature 465 755

[20]Du J J,Li W F,Wen R J,Li G,Zhang P F and Zhang T C 2013 Appl.Phys.Lett.103 129903

[21]Zhang P F,Guo Y Q,Li Z H,Zhang Y C,Zhang Y F,Du J J,Li G,Wang J M and Zhang T C 2011 Phys.Rev.A 83 031804

[22]Nußmann S,Hijlkema M,Weber B,Rohde F,Rempe G and Kuhn A 2005 Phys.Rev.Lett.95 173602

[23]Hood C J,Lynn T W,Doherty A C,Parkins A S and Kimble H J 2000 Science 287 1447

[24]Agarwal G S 1984 Phys.Rev.Lett.53 1732

[25]Rempe G,Thompson R J and Kimble H J 1994 Phys.Scr.1994 67

[26]Grimm R,Weidemüller M and Ovchinnikov Y B 2000 Advances in Atomic,Molecular,and Optical Physics(Academic Press)pp.95–170

[27]Sun H Y 1998 Opt.Eng.37 2906

[28]Gardiner C W,Ye J,Nagerl H C and Kimble H J 2000 Phys.Rev.A 61 045801

[29]Savard T A,O’Hara K M and Thomas J E 1997 Phys.Rev.A 56 R1095

[30]Roati G,Jastrzebski W,Simoni A,Modugno G and Inguscio M 2001 Phys.Rev.A 63 052709

[31]Schrader D,Kuhr S,Alt W,Müller M,Gomer V and Meschede D 2001 Appl.Phys.B 73 819

[32]Greiner M,Bloch I,H¨ansch T W and Esslinger T 2001 Phys.Rev.A 63 031401

[33]Wang J,He J,Yan S B,Geng T,Zhang T C and Wang J M 2008 Acta Sin.Quantum Opt.14 44(in Chinese)

[34]Andrews M R,Townsend C G,Miesner H J,Durfee D S,Kurn D M and Ketterle W 1997 Science 275 637

[35]Deiglmayr J,Saßmannshausen H,Pillet P and Merkt F 2014 Phys.Rev.Lett.113 193001

[36]Maunz P,Puppe T,Schuster I,Syassen N,Pinkse P W H and Rempe G 2004 Nature 428 50

[37]Blatt S,Mazurenko A,Parsons M F,Chiu C S,Huber F and Greiner M 2015 Phys.Rev.A 92 021402

[38]Wen R J,Du J J,Li W F,Li G and Zhang T C 2014 Acta Phys.Sin.63 244203(in Chinese)

[39]Tuchendler C,Lance A M,Browaeys A,Sortais Y R P and Grangier P 2008 Phys.Rev.A 78 033425