Mechanical response of continuously reinforced concrete pavement on foam concrete interlayer for two-way curved arch bridge

Chen Xiaobing Xu Libin Liu Han He Chusheng

(School of Transportation, Southeast University, Nanjing 210096, China)

Abstract：In order to research the mechanical response of continuously reinforced concrete pavement on foam concrete interlayer for a two-way curved arch bridge, the elliptical vehicle load is translated into the rectangular load based on the equivalence method. Then, a three-dimensional finite element model of the whole bridge is established. The reliability of the model is verified. Additionally, the mechanical response of continuously reinforced concrete pavement under vehicle loading is analyzed. Finally, the most unfavorable loading conditions of tensile stress, shear stress and vertical displacement are determined. The results show that the most unfavorable loading condition of tensile stress, which is at the bottom of continuously reinforced concrete pavement on the two-way curved arch bridge, is changed compared with that on homogeneous foundation. The most unfavorable loading condition of shear stress at the top is also changed. However, the most unfavorable loading condition of vertical displacement remains unchanged. The tensile stress at the bottom of about 1/4 span of the longitudinal joint, the shear stress at the top of intersection of transverse and longitudinal joint, together with the vertical displacement at the central part of longitudinal joint, are taken as design indices during the structural design of continuously reinforced concrete pavement on the two-way curved arch bridge. The results are helpful for the design of continuously reinforced concrete pavement on unequal-thickness base for the two-way curved arch bridge.

Keywords：two-way curved arch bridge; bridge deck pavement; unequal-thickness base; continuously reinforced concrete pavement; unfavorable loading condition; finite element method (FEM)

Continuously reinforced concrete pavement has advantages such as improved driving comfort, high carrying capacity, long service life, low maintenance cost and wide application range as a high-performance concrete pavement[1-2]. Compared with ordinary concrete pavement, the continuously reinforced concrete pavement prevents the deformation of concrete and distress caused by setting joints such as mud-pumping and faulted slabs[3-4]. Thus, it is widely applied in the road and deck pavement areas.

The continuously reinforced concrete pavement applied for homogeneous foundation has been continuously gaining attention in the civil engineering community[5-9]. Research results show that the most unfavorable loading condition of tensile stress, which is at the bottom of continuously reinforced concrete pavement on homogeneous foundation, is located at the central part of the longitudinal joint or transverse joint. For a composite pavement with continuously reinforced concrete pavement as the base, the most unfavorable loading condition of shear stress, which is at the top of continuously reinforced concrete pavement on homogeneous foundation, is symmetrically distributed at the central part of transverse joint.

However, there are often special bases in practical engineering, such as a two-way curved arch bridge foundation. Two-way curved arch bridges are widely used in the south of Yangtze River in China[10]. The thickness of its foam concrete fill is changed along the longitudinal direction, which forms the unequal-thickness base. Thus, it is necessary to analyze the mechanical response of continuously reinforced concrete pavement on the unequal-thickness foam concrete base for two-way curved arch bridges under vehicle loading. The most unfavorable loading condition should be determined as well.

In this study, the elliptical vehicle load is translated into the rectangular load based on the equivalence method[11]. Secondly, a three-dimensional whole-bridge finite element model of continuously reinforced concrete pavement on the two-way curved arch bridge is built. The reliability of the model is verified. Thirdly, the mechanical response of continuously reinforced concrete pavement under vehicle loading is analyzed. Finally, the most unfavorable loading conditions of tensile stress, shear stress and vertical displacement are determined. The results are helpful for the design of a continuously reinforced concrete pavement on the two-way curved arch bridge.

1 Simulation of Vehicle Load

As a vehicle drives along the road, the contact area between a wheel and pavement is approximately composed of one rectangular and two semicircles, as shown in Fig.1(a). The equivalence method is used to transfer the elliptical contact area in Fig.1(a) into the rectangular area in Fig.1(b)[11]. In the finite element model, the length of the load area is 23 cm, and the width is 16 cm +16 cm +16 cm, as shown in Fig.2. The single-axle double-wheel standard load with the grade of 100 kN is taken. The tire pressure is 0.7 MPa. Each double-wheel weighs 50 kN. Half the size of single-axle double-wheel standard load is applied in the contact area, when the combined effects of braking load and longitudinal slope are considered. The vertical load in the contact area is 0.7 MPa and the horizontal load is 0.35 MPa when the contact area is assumed to be constant.

(a)

(b)Fig.1 Transformation of the contact area

Fig.2 Load area in finite element model (unit: cm)

2 Static Model of Pavement

The stiffness of the main arch ring and abdominal arch ring of the two-way curved arch bridge are different. The thickness of the foam concrete fill is also different in the longitudinal direction. This special structure makes it difficult to solve the mechanical response of continuously reinforced concrete pavement, which is applied for the unequal-thickness foam concrete base for the two-way curved arch bridge. Thus, based on the numerical simulation method, ABAQUS software is used to establish a three-dimensional finite element model of the two-way curved arch bridge deck pavement. Two-span one-link continuously reinforced concrete pavement is adopted in this study. A symmetry principle is used to simplify two spans into one span.

2.1 Basic assumption

Considering the characteristics of the structure, the following assumptions are proposed for the FEM:

1) The bridge deck pavement system is an integrated system composed of homogeneous, continuous and isotropic elastic materials.

2) The steels in continuously reinforced concrete pavement are regularly arranged in straight line and completely bonded to the cement concrete.

3) The deflection of continuously reinforced concrete slab is far smaller than its thickness.

2.2 Structure and material parameters

According to the reinforcement and reconstruction project of Nanjing Yangtze River Bridge in 2017, the span of 34.9 m is adopted in the simulation. The width of roadway is 15 m with a sidewalk of 1.73 m at each side in the transverse direction. The total width in transverse direction is 18.46 m. The two-way curved arch bridge is symmetrical in transverse direction. Thus, the half width of 9.23 m is taken in transverse direction along the middle line of pavement. The vertical elevation of single span is shown in Fig.3. The material of spandrel fill is foam concrete. The 20 cm-thickness cast-in-place continuously reinforced concrete pavement is poured on the foam concrete base, and the vertical and horizontal steels are arranged at 7 cm from the top of the pavement. The longitudinal steel is rebar with 20 mm diameter and six of them are arranged for each linear meter. The transverse steel is rebar with 12 mm diameter and six of them are arranged for each linear meter. The material parameters are shown in Tab.1.

Tab.1Material parameters used in the finite element model

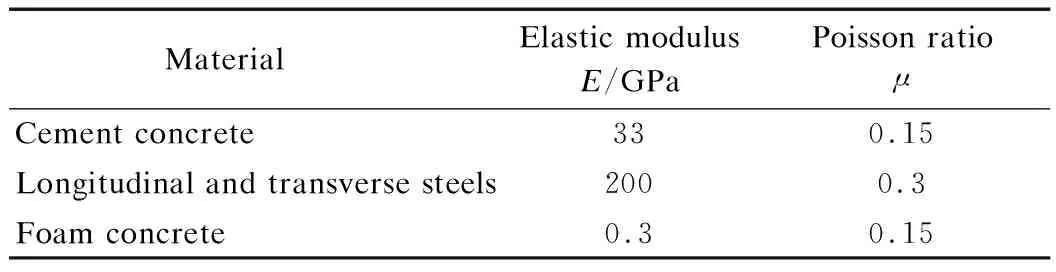
Material Elastic modulusE/GPaPoisson ratioμCement concrete330.15Longitudinal and transverse steels2000.3Foam concrete0.30.15

Fig.3 Vertical elevation of two-way curved arch bridge (unit: cm)

2.3 Mesh generation and unit selection

The continuously reinforced concrete pavement is meshed into 20-node hexahedron secondary reduced integral units, C3D20R[9]. The pavement layer is divided into six in simulation to improve accuracy. The steels are meshed into 2-node space linear beam units, B31[12]. The other bridge structures are meshed into 8-node linear hexahedron reduced integral units, C3D8R.

2.4 Boundary condition

Considering the characteristics of the structure, the following boundary conditions are proposed:

1) The arch springing and upright column at two longitudinal sides are fully fixed.

2) The horizontal displacement of the transverse symmetry of the model is fixed.

3) The continuously reinforced concrete pavement and foam concrete fill are completely fixed at the fixed end and hinged at the simply supported end.

3 Model Verification

According to the national standard of China[13], the stress of free concrete pavement on homogeneous foundation under the most unfavorable loading condition can be calculated as

(1)

whereσpsis the stress of free concrete pavement under the most unfavorable loading condition, MPa;Ecis the bending elasticity modulus of cement concrete, MPa;hcis the thickness of concrete pavement, m;Etis the equivalent resilient modulus of foundation under the concrete pavement, taking 375 MPa;υcis the Poisson ratio of cement concrete;Psis the designed axle load, taking 100 kN.

ABAQUS is used to establish finite element models to calculate the load stress of free concrete slabs which have different sizes, with the axle load at the central part of longitudinal joint. The size of each slab is shown in Fig.4. Taking slab 1 as an example, the load position is illustrated in Fig.5. Free concrete slabs are placed on homogeneous foundation and unequal-thickness foam concrete base for the two-way curved arch bridge, respectively, as shown in Fig.6 and Fig.7. Then,σpsof each slab in Eq.(1) is calculated by taking the material parameters in Tab.1. The results of the formula and two finite element models are compared in Tab.2 and Fig.8. Since the two finite element models are both symmetrical in the longitudinal direction, only the results of six slabs on one side in the longitudinal direction are compared.

Fig.4 Size of different slabs (unit: cm)

Fig.5 Load position of slab 1 (unit: cm)

(a)

(b)Fig.6 Pavement on homogeneous foundation. (a) Finite element model;(b) Load stress of slab 1

(a)

(b)Fig.7 Pavement on two-way curved arch bridge foundation. (a) Finite element model; (b) Load stress of slab 1

SlabnumberSize/(cm×cm)σps/MPaσh/MPaΔσ1/%σa/MPaΔσ2/%1650×3751.6521.6450.41.7304.72730×3751.6521.6430.51.7103.53730×3751.6521.6540.11.6261.66650×5481.6521.6450.41.7304.77730×5481.6521.6430.51.7103.58730×5481.6521.6540.11.6261.6

Notes:σhis the result of homogeneous foundation model, MPa;σais the result of arch bridge foundation model, MPa; Δσ1is the error betweenσhandσps, Δσ1=|σps-σh|/σps,%; Δσ2is the error betweenσaandσps, Δσ2=|σps-σa|/σps,%.

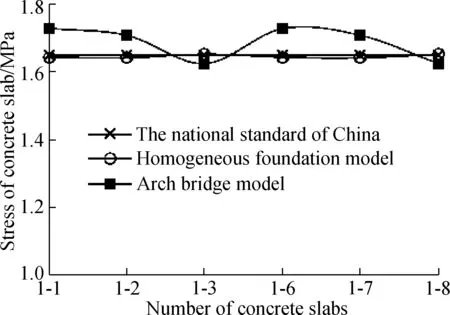
Fig.8 Comparison of load stress

It can be seen from Tab.2 and Fig.8 that the load stresses of free concrete pavement on the homogeneous foundation, which are simulated by ABAQUS and calculated by the formula of national standard of China, are basically consistent. Thus, the finite element model used in this study is reliable[14]. On this basis, the free concrete pavement on the unequal-thickness foam concrete base is changed into continuously reinforced concrete pavement, to analyze the most unfavorable loading condition of continuously reinforced concrete pavement on the two-way curved arch bridge.

4 The Most Unfavorable Loading Condition

The composite pavement structure is adopted in the reinforcement and reconstruction project. Since there is no asphalt layer in the sidewalk, an asphalt surface layer with a thickness of 9 cm, length of 34.9 m and width of 7.5 m is added to the model. The asphalt pavement is hinged on both sides in the longitudinal direction and meshed into C3D20R. The upper layer of the asphalt pavement is 4 cm-thickness SMA-13, whose elastic modulus is 1 400 MPa and Poisson ratio is 0.25. The bottom layer of the asphalt pavement is 5 cm-thickness SMA-10, whose elastic modulus is 1 200 MPa and Poisson ratio is 0.25. Each layer of the asphalt pavement is divided into two in simulation. The mesh generation of the composite pavement model is shown in Fig.9.

(a)

(b)

4.1 Selection of load position

Given that the vehicle load only acts on the roadway, the effective width of the continuously reinforced concrete pavement is taken as 750 cm. Every 1 m, a load position from the center of slab to the central part of longitudinal joint in transverse direction, as well as every 4 m a load position from the central part of longitudinal joint to the corner of slab in longitudinal direction, is taken as the load positions in simulation, as shown in Fig.10.

(a)

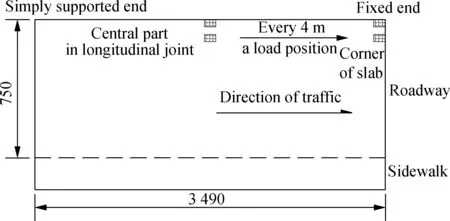
(b)Fig.10 Load position. (a) In transverse direction; (b) In longitudinal direction (unit:cm)

4.2 Mechanical response of continuously reinforced concrete pavement

The mechanical response of the continuously reinforced concrete pavement with different load positions are shown in Fig.11 to Fig.14.

4.3 Unfavorable loading condition of tensile stress

The most unfavorable loading condition of the maximum longitudinal tensile stress, which is at the bottom of the continuously reinforced concrete pavement, is 8 m to the central part of longitudinal joint, namely about 1/4 span in longitudinal joint. The value of the maximum longitudinal tensile stress is 1.580 MPa, as shown in Fig.15(a). The most unfavorable loading condition of the maximum transverse tensile stress, which is at the bottom of continuously reinforced concrete pavement, is also about 1/4 span in longitudinal joint. The value of the maximum transverse tensile stress is 1.079 MPa, as shown in Fig.15(b). However, those two of homogene-ous foundations are at the central part of longitudinal joint. Thus, the most unfavorable loading condition of tensile stress, which is at the bottom of the continuously reinforced concrete pavement on unequal-thickness foam concrete base for the two-way curved arch bridge, is changed.

Fig.11 Relationship of the maximum longitudinal tensile stress at the bottom and load position

Fig.12 Relationship of the maximum transverse tensile stress at the bottom and load position

Fig.13 Relationship of the maximum shear stress at the top and load position

Fig.14 Relationship of the maximum vertical displacement and load position

(a)

(b)Fig.15 Tensile stress of continuously reinforced concrete pavement. (a) In transverse direction; (b) In longitudinal direction

4.4 Unfavorable loading condition of shear stress

The most unfavorable loading condition of the maximum shear stress, which is at the top of the continuously reinforced concrete pavement, is at the corner of the slab, namely the intersection of the transverse and longitudinal joints. The value of the maximum shear stress is 0.819 MPa, as shown in Fig.16, while that of the homogeneous foundation is symmetrically distributed at the center of the transverse joint. Thus, the most unfavorable loading condition of shear stress, which is at the top of the continuously reinforced concrete pavement on the unequal-thickness foam concrete base for the two-way curved arch bridge, is changed.

Fig.16 Shear stress of continuously reinforced concrete pavement

4.5 Unfavorable loading condition of vertical displacement

The most unfavorable loading condition of the maximum vertical displacement of the continuously reinforced concrete pavement is the central part of the longitudinal joint. The value of the maximum vertical displacement is 0.155 mm, as shown in Fig.17, while that of the homogeneous foundation is also the central part of the longitudinal joint. Thus, the most unfavorable loading condition of vertical displacement of continuously reinforced concrete pavement, which is applied for the unequal-thickness foam concrete base for the two-way curved arch bridge, remains unchanged.

Fig.17 Vertical displacement of continuously reinforced concrete pavement

5 Conclusions

1) The most unfavorable loading condition of the maximum tensile stress, which is at the bottom of the continuously reinforced concrete pavement on the unequal-thickness foam concrete base for the two-way curved arch bridge, is about 1/4 span in the longitudinal joint. It is different from that on the homogeneous foundation.

2) The most unfavorable loading condition of the maximum shear stress, which is on the top of continuously reinforced concrete pavement on the unequal-thickness foam concrete base for the two-way curved arch bridge, is at the intersection of the transverse and longitudinal joints. It is different from that on the homogeneous foundation.

3) The most unfavorable loading condition of the maximum vertical displacement of the continuously reinforced concrete pavement, which is applied to the unequal-thickness foam concrete base for the two-way curved arch bridge, is the central part of the longitudinal joint. It is the same as that on the homogeneous foundation.

4) During the structural design of the continuously reinforced concrete pavement on the unequal-thickness foam concrete base for the two-way curved arch bridge, the tensile stress at about 1/4 span in the longitudinal joint, the shear stress at the intersection of the transverse and longitudinal joints, and the vertical displacement at the central part of the longitudinal joint should all be taken as design indices.