Non-Markovian Master Equation for Distant Resonators Embedded in a One-Dimensional Waveguide∗

Xin-Yu Chen(陈歆宇),Wen-Zhao Zhang(张闻钊),and Chong Li(李崇),†

1School of Physics,Dalian University of Technology,Dalian 116024,China

2Beijing Computational Science Research Center,Beijing 100193,China

AbstractWe develop a master equation approach to describe the dynamics of distant resonators coupled through a one-dimensional waveguide.Our method takes into account the back-actions of the reservoirs,and enables us to calculate the exact dynamics of the complete system at all times.We show that such system can cause nonexponential and long-lived photon decay due to the existence of a relaxation effect.The physical origin of non-Markovianity in our model system is the finite propagation speed resulting in time delays in communication between the nodes,and strong decay rate of the emitters into the waveguide.When the distance satis fies the standing wave condition,we find that when the time delay is increased,the dark modes formation is no longer perfect,and the average photon number of dark mode decreases in steady time limit.

Key words:non-Markovian master equation,quantum network,waveguide QED

1 Introduction

Quantum network is the backbone of distributed quantum computing architecture and quantum communication.[1−2]It comprises ofa set ofnodes exchanging quantum information via connecting quantum channels. Quantum networks include both local quantum networks connecting small-scale quantum computers,[3−5]or wide-area quantum networks between distant nodes.[6]The former mainly focuses on the local interaction between qubits,input-output formalism for few-photon transport[7−8]and the local quantum information process such as single-photon router,[9−11]quantum rectifier,[12−14]and quantum gates.[15−17]Due to high propagation speed and small system scale,these works are mostly described by the Born-Markov(BM)approximation based on weak-coupling perturbation theory and the neglect of time delays in interactions.[18]Many local quantum network setups have been experimentally demonstrated in recent years.[19−20]

At the same time that the technology evolves,recent experiments begin to investigate the regime of a non-negligible delay time because of interest in scalable quantum networks.[21−24]Unlike local quantum network,a wide-area quantum network usually contains many distant nodes to implement long-distance quantum information tasks such as quantum state transfer between remote nodes.[1,25−26]The finite propagation speed of these excitations naturally introduces time delays in the dynamics,which can invalidate the Markov approximation.In order to analyze the non-Markovian effect of quantum network exactly,several analytical and numerical methods have been developed,where the Born-Markov approximation is not involved.This includes the numerical Green function approach,[27]the time-dependent Schrödinger equation approach,[28]the full quantum electrodynamical approach,[29]the path integral approach,[30]the extended master equation method,[31]and so on.However,an exact master equation describing the non-Markovian dynamics of a quantum network is rare.So,further development of theoretical methods is required.

In this paper,we study the non-Markovian dynamics of distant resonators coupled through a one-dimensional waveguide.The dissipation dynamics of the resonators alone are obtained by integrating out the degrees of freedom of reservoirs.As a result,the back-action between the waveguide and the resonators can be fully taken into account.We then derive the exact master equation nonperturbatively.The transient and steady state dynamics of the system can be obtained directly from the master equation.In the short time delay limit,we find that the exact solution given by our master equation is in good agreement with the Markovian solution in previous work,which justifies the validity of the Markov approximation.However,when the distance between the nodes is raised,the deviation between the exact solution and the Markovian solution can be extremely large.Under the standing wave condition,similar to the Markovian case,we find that the non-localized mode is uncoupled from the waveg-uide and become so-called dark mode of the system in the steady state limit.However,we notice that the average photon number in steady time limit decreases with the increase of time delay.Finally,by comparing with the exact numerical solution,we find that our master equation can accurately describe the non-Markovian dynamics of the system.

2 The Model

As illustrated in Fig.1,our model comprises an infinite one-dimensional waveguide lying along the x axis,containing Ncnon-interacting resonators(cavities)placed at x=xn.The waveguide have a continuous spectrum of photons in modes propagating to the left and right.Under the dipole and rotating wave approximation,the Hamiltonian of such a system(cavities plus waveguide)takes the form H(t)=Hsys(t)+Hw+Hint,with

Fig.1 One-dimensional waveguide coupled to an array of cavities placed at xn.Cavities decay by emitting photons into the right-and left-moving modes of waveguide.

3 The Exact Master Equation for Dissipative Photonic Dynamics

To investigate photon dynamics of distant cavities embedded in a one-dimensional waveguide,we shall concentrate on the reduced density matrix for the quantum emitter by tracing out the free degree of waveguide,ρ(t)=Trwρtot(t).We suppose that the waveguide is initially in the vacuum state and the initial density matrix is factorized into a direct product of the system and the waveguide state.[33]i.e.,ρtot(t0)= ρ(t0)⊗ ρw(t0).Using the Feynman-Vernon in fluence functional approach,[34−35]we can integrate out completely the waveguide degree of freedom in the coherent representation[36]and obtain the following exact master equation,

This master equation determines completely the quantum coherence dynamics of the driven resonators system.[37−41]

is the effective Hamiltonian of driving resonators with the renormalized cavity frequencies(t)and the renormalized driving fieldThe second non-unitary term describes the dissipation non-Markovian dynamics with time-dependent dissipation coefficient γmn(t)due to the interaction between the resonators and waveguide.All these time-dependent coefficients can be determined nonperturbatively and exactly through the relations[39−40]

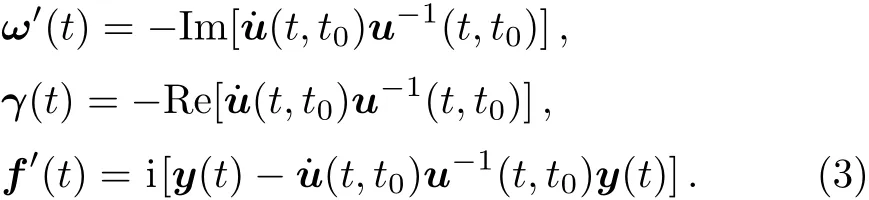
Here the Nc×Ncmatrix function u(t,t0)is the cavity photon field propagating Green’s function describing the photon field relaxation,and y(t)is 1×Ncmatrix.These time-dependent functions obey the equations of motion,

subjected to the boundary conditions u(t0,t0)=I and y(t0)=0 with t≥t0,where we have defined the Nc×Ncmatrix ω =diag(ω1,ω2,...).The time non-local integral kernel in above equation is the so-called dissipation kernels and represents non-Markovian memory effects on the system due to its coupling to waveguide.It can be expressed aswhere σR=+and σL= − for the right-and left-moving modes,respectively.After u(t,t0)determined by Eq.(4),we can easily find that

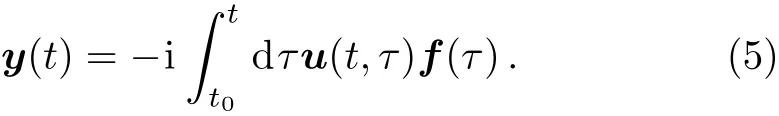
The real time non-equilibrium photon dynamics of the systems is fully described by the master equation(2).

A quantum network consists in general of many nodes,which are connected by the waveguide to implement longdistance quantum communication via emission and absorption of photons.The finite propagation speed of photons naturally introduces time delays in the dynamics,which can invalidate the Markov approximation.To study Non-Markovian effects of retardation, first,we consider a minimal model of a pair of driven resonators coupled to the waveguide.For simplicity,we assume ω1= ω2= ω and γ1= γ2= γ.The large distance between two cavities is d=|x1−x2|,such that the delay time td=d/vgbetween them is comparable to their lifetime,even greater than their lifetime.Using Eq.(4),after performing the integrations over t,we obtain delay differential equation for u(t)with associated time delay td(setting t0=0 for simplicity):

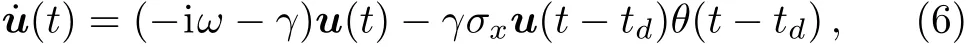
where θ(t)is the Heaviside step function.The first term on the right-hand side describes standard spontaneous emission with rate γ.The second term represents that the retardation interaction caused by another atom can occur at times t≥td.

We note that Eq.(6)is a set of coupled differential equations.In order to decouple the Eq.(6),we introduce two non-localized bosonic modes,which are linearly related to the field modes of two cavities.In the asymmetric/symmetric basis,Eq.(6)split into two independent delay differential equations for˜u(t)=

subjected to the boundary conditions˜u(0)=I with t≥0,where H is Hadamard matrix.Using contour integral techniques[42−43]and solving Eq.(7)iteratively by partitioning the time axis into time interval td,we can obtain the analytical solution of the propagating Green’s function

where

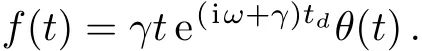
From Eq.(8),each term of the summation describes the contribution of multiple re flection and reabsorption of propagating photons from cavity 1 to cavity 2 and vice versa.

4 Non-Markovian Dynamics of System

In this section,we study dissipation photon dynamics of distant resonators coupled to the waveguide beyond the Markovian regime.As discussed in the Introduction,Born-Markov approximation is valid when propagation time of the photon can be ignored,i.e.γtd≪1.In this regime,Eq.(8)can be approximated as

Using Eq.(3),we can find that all the coefficients in Eq.(3)are constants,

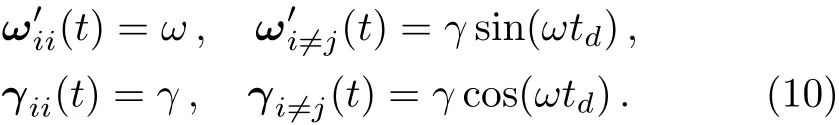
The exact master equation(2)agrees with the Markovian master equation[44−45]where the decoherence rates are time independent.This gives the standard Lindblad form for the Markovian dynamics.

The photon dynamics of distant cavities,⟩,are plotted in Fig.2. In this section,we set the system with the same initial statefor simplicity.From Fig.2(a),we find that the dissipation dynamics of the cavity photon modes are standard spontaneous emission and the solution given by the exact master equation(2)is in good agreement with the Markovian solution[44−45]in the regime γtd≪ 1.Figure 2(b)shows that the corresponding decay rates γiiare constant and positive,which leads to a Markovian dynamics.This means that the interaction between two resonators can be considered to be instantaneous and the reservoir has no memory effect on the evolution of the system.

To study the regions of beyond the Markovian regime,we can increase the separation between the emitters.When the time delay tdis comparable to the characteristic spontaneous emission time 1/γ,retardation effects play a significant role in the system dynamics and the Markovian master equation cannot describe system dynamics any more.Dissipation rate is not constant.The system dynamics depend on the time delay,and thus the time evolution of the system must be described by the exact master equation(2).

Figure 2(c)shows the deviation between the exact solution and the Markovian solution becomes relatively obvious in the regime γtd≃ 1.The corresponding damping rates γijare given in Fig.2(d).We can observe two clearly distinct regions.At times t

Fig.2(a),(c)Time evolution of the average cavity photon number with the BM solution(dashed line)and the exact solution(solid line),and(b),(d)the corresponding decay rate γijfor(a),(b) γt=0.1 and(c),(d) γt=1.Values of other parameters are γ/ω =0.01 and ωl=0.

In the following,we discuss retardation effects in the long-time limit.From Eq.(3),we find that the decay of the symmetric(asymmetric)mode depends strongly on the distance between two emitters.In particular,when the distance satis fies the standing wave condition,i.e.ωtd=nπ (n is even number),the decay of the asymmetric mode into the waveguide modes is completely suppressed.Because of the interaction between two emitters,the asymmetric mode is uncoupled to the waveguide and become so-called dark mode.When the distance satis fies the other standing wave conditions ωtd=nπ (n is odd number),we note that the symmetric mode would replace the asymmetric one and be uncoupled to the reservoir.However,according to the above results,when the separation between the emitters is increased,Markov approximation is no longer valid and the retardation effect has to be taken into account.With the help of Eq.(2),the average photon number of two non-localized photon modes evolve as

Using the final value theorem,the average photon number of the non-localized modes in the long-time limit is given by

where F±(s)is the Laplace transform of

From Eq.(12),the dynamics of the two non-localized modes are similar,because there is only one π phase difference between them.Without loss of generality,here we only study the separation is resonant for the asymmetric mode.In Fig.3,we show the evolution of the average photon number of the asymmetric mode for different separation between two emitters.The corresponding decay rate κ(t)is given in Fig.3(d).From Eq.(12),we note that the average photon number mainly depends on the time delay γtd.The dynamics of the average photon number of asymmetric mode reach a stable value in the long-time limit.This is caused by a destructive interference between the different paths that the photon emitted by the two resonators.However,the dark mode of the system is no longer perfect as in the Markovian case,and the average photon number in steady time limit decreases with the increase of time delay.At time t

Fig.3(a)-(c)Time evolution of the average photon number of non-localized modes with the BM solution(dashed line)and the exact solution(solid line),and(d)the corresponding decay rate of asymmetric mode γ− for(a)td=2π/ω,(b)td=20π/ω,and(c)td=200π/ω.Values of other parameters are γ/ω =0.01 and ωl=0.

Fig.4 Comparison between the results of exact MPSs evolution method(dashed line)and our master equation method(solid line)with different coupling strengths and different driving field frequencies.

To give the regime of validity of our master equation method in this paper,we introduce matrix product states(MPSs)techniques,[46−47]which are originally developed in a condensed-matter context to enable an efficient description of one-dimensional many-body system.The state of the total system(cavities plus waveguide)is described as an MPSs and evolved according to the Schrödinger equation.[48]This allows one to simulate the non-Markovian dynamics of the system for large retardation time td.Figure 4 shows that the evolution of the photon number as computed by our master equation method used in this paper and the numerically exact MPSs evolution method with different coupling strengths and different driving field frequencies.By contrast,we find that whatever the case is,the analytic solution derived from our master equation is perfectly consistent with the exact numerical solution obtained by the MPSs evolution method.This shows that our master equation can exact describe the dynamics of the system,because we take full consideration of the reaction of the environment.

5 Conclusions

We have studied dissipation dynamics of distant resonators coupled through a one-dimensional waveguide.Making use of the Feynman-Vernon in fluence functional theory,we have derived an exact non-Markovian master equation for the distant resonators by treating the waveguide as environment.The back-reactions between waveguide and resonators are fully accounted.The photonic dynamics of the system can be obtained directly from the master equation.We have shown that the exact solution obtained from our master equation is in good agreement with the Markovian solution in previous work in the short time delay limit.Our results show that non-Markovian effects start to appear due to the increase of delay time.The deviations can be extremely large,and the Markov approximation is invalid in the long time delay regime.Similar to the Markovian situation,when the separation between the nodes is resonant for the symmetric(asymmetric)mode,the symmetric(asymmetric)mode is uncoupled to the waveguide and become so-called dark mode in the steady state limit.This is caused by a destructive interference between the different paths that the photon emitted by the two resonators.However,we have noticed that the average photon number in steady time limit decreases with the increase of time delay.Finally,we have verified the validity of our non-Markovian master equation by comparing the analytic solution derived from our master equation with the exact numerical solution obtained by the MPSs evolution method.