时间约束下帆板挠性振动抑制的预测与反演控制
2018-01-04谭天乐


摘要: 针对航天器挠性帆板的振动抑制问题开展研究,基于模态空间中状态方程形式的动力学模型,在离散时间系统中求解太阳帆板振动受控下模态坐标随时间变化的规律;对振动模态坐标的状态转移进行预测,利用模型预测方法外推预测挠性各振动模态坐标状态的预计偏差;采用广义逆方法,反演得到抑制挠性振动所需的控制序列; 通过逐步逼近的方法,设计了在给定的振动抑制时间约束下,太阳帆板挠性振动抑制的模型预测与反演控制律;分析了该方法在应用中的一些特点;仿真结果表明,所提出的方法能够实现指定时间约束下航天器太阳帆板的快速振动抑制。
关键词: 太阳帆板; 振动抑制; 模型预测与反演控制; 挠性; 时间约束
中图分类号: V414.3+3; TB535 文献标志码:A 文章编号1004-4523(2018)05-0744-08
DOI:10.16385/j.cnki.issn.1004-4523.2018.05.003
引 言
挠性结构在空间飞行器上普遍存在, 如太阳帆板、空间机械臂或天线等。这些结构通常尺寸大,质量轻,刚度相对较小,结构振动模态频率低且密集、模态阻尼小,在伸展、转动过程中容易受到各种内、外部激振,且振动常会持续较长时间,直接影响航天器本体姿态的高精度控制。为抑制挠性结构的振动,国内外先后有学者提出了输入成形法[1-2]、线性二次型最优控制[3-4]、分力合成法[5]、正位置/速度反馈[6-8]、鲁棒控制[9]、自适应控制[10-11]、滑模变结构控制[12-13]、最优控制[14-15]、模糊/神经网络控制[16-17]、预测控制[18-21]等控制方法。其中预测控制采用模型预测、反馈校正和滚动优化,通过二次规划方法求解关于控制偏差及控制量的加权目标函数以得到控制输出,对于振动抑制过程通常没有时间约束。系统控制周期的选择对控制过程和结果有较大影响。上述方法中,在控制姿态的同时考虑抑制挠性振动的集中控制方法需要较快的采样测控速度和较宽的控制系统带宽,控制系统阶数高,系统设计难以兼顾,常规的姿态敏感器、控制器、执行机构等难以满足要求,工程实现困难。
随着频带宽、响应速度快、体积小、质量轻、埋入粘贴方便的压电材料的出现,在连接结构上减小航天器刚体部分与挠性附件的耦合影响,对航天器姿态控制和振动抑制分别进行设计,采用不同的测量敏感器、控制器和执行机构,形成相对独立的控制回路,进行分散控制是目前航天器挠性附件振动抑制的一种有效途径。
在模态空间[22-24]中进行控制器设计是挠性振动主动控制的一种常用方法。模态空间法采用模型降阶将无限自由度的振动控制转化为模态空间内有限个振动模态的控制,虽然可能存在不受控模态所引起的“溢出”问题,但在考虑足够多的、能量较为集中的几个主要模态,通过模态截断准则对振幅小、能量少的高阶模态进行截断后,可以降低控制系统设计复杂程度,减小控制器阶数,较好地抑制挠性结构振动所导致的姿态干扰,因此基于模态空间的控制方法在工程上得到了较为广泛的应用。
本文在状态方程形式的航天器帆板挠性振动动力学模型基础上,对模态坐标的状态转移过程进行求解,获得模态坐标状态的演变规律,外推预测挠性振动各个模态坐标状态的预计偏差,反演抑制挠性振动所需的控制序列;根据振动抑制的时间约束,控制模态坐标逐步逼近期望状态,设计了在时间约束条件下,帆板挠性振动抑制的模型预测与反演控制律,讨论了该方法在应用中的一些特点,通过仿真验证了控制方法的有效性。
1 太阳帆板模态空间模型
航天器上的太阳帆板通常由铝蜂窝两侧覆盖碳纤维/环氧复合材料薄板组成,四周安装有铝框。太阳帆板及安装于其上的应变片、压电陶瓷片共同构成太阳帆板智能结构和主动振动控制回路。应变片测量帆板的弯曲位移,压电片激励和控制弯曲振动。
模态坐标状态的振幅表征了振动的能量。比较图1和2,易知随着时间由0→3 s,需要阻尼和抵消的能量是逐渐增加的,故挠性抑制过程中压电作动器输入电压随时间逐渐增大。
对于有更多模态需要进行振动抑制的动力学系统,以及敏感器、控制器与执行机构能够满足控制要求的航天器姿态、帆板一体化集中控制系统,MPIC-TC方法同樣是适用的。此外,在不同的系统控制周期下,帆板挠性振动模态均能在3 s内较为精确地达到零位移状态。
5 结 论
本文所提出的方法遵循挠性振动模态坐标状态受控转移的规律,可以较为精确地实现指定时间下帆板挠性的振动抑制。在每个采样控制周期中,根据剩余的模态坐标状态转移时间控制器参数是时变自适应的,与初始状态和期望状态无关。后续可以在多输入、控制输出受限和能量最小等约束及目标下,基于预测模型反演控制的一般解,对控制方法和参数进行进一步优化。
参考文献:
[1] 姚雨晗, 周 军, 刘莹莹. 基于输入成型法的空间站变构型过程挠性振动抑制策略[J]. 宇航学报, 2013, 34(5):665—670.
Yao Yuhan, Zhou Jun, Liu Yingying. Research on flexible vibration suppression strategy for variable configuration space station based on input shaping technique[J]. Journal of Astronautics, 2013, 34(5):665—670.
[2] Deng H, Sun J D, Huang S D. Vibration suppression of the flexible manipulator using optimal input shaper and linear quadratic regulator [C]. 12th International Conference on Ubiquitous Robots and Ambient Intelligence, Goyangcity, Korea, 2015:255—260.
[13] 肖 岩,叶 东, 孙兆伟. 面向刚柔耦合卫星的有限时间输出反馈姿态控制[J]. 宇航学报, 2017, 38(5):516—525.
Xiao Yan, Ye Dong, Sun Zhaowei. Finite-time output feedback attitude control for rigid-flexible coupling satellites [J]. Journal of Astronautics, 2017, 38(5): 516—525.
[14] 王晓明, 周文雅, 寻广彬, 等. 带有振动抑制的压电结构动态形状主动控制[J]. 宇航学报, 2017, 38(2):185—191.
Wang Xiaoming, Zhou Wenya, Xun Guangbin, et al. Dynamic shape control of piezoelectric structures with vibration suppression [J]. Journal of Astronautics, 2017, 38(2):185—191.
[15] Yu Y, Han F, Leng X Z, et al. Design and implementation of a wireless optimal controlscheme for active structural vibration control [C].Fifth International Conference on Instrumentation and Measurement, Computer, Communication and Control, Qinhuangdao, China, 2015:901—906.
[16] Chen Y, Dong C Y. A fuzzy-neural network sliding mode control for flexible spacecraft[C]. 2nd International Conference on Information Engineering and Computer Science, Wuhan, China, 2010, 5677732.
[17] Qiu Z C, Zhang X T, Ye C D. Vibration suppression of a flexible piezoelectric beam using bp neural network controller [J]. Acta Mechanica Solida Sinica, 2012, 25(4):417—428.
[18] Lin C Y, Huang Y H. Enhancing vibration suppression in a periodically excited flexible beam by using a repetitive model predictive control strategy[J]. Journal of Vibration and Control, 2016, 22(16) 3518—3531.
[19] Takács G, Batista G, Gulan M, et al.Embedded explicit model predictive vibration control [J]. Mechatronics, 2016, 36 :54—62.
[20] Yuan Y, Zhang H T,Wu Y, et al. Bayesian learning-based model predictive vibration control for thin-walled workpiece machining processes [J]. IEEE/ASME Transactions on Mechatronics, 2017, 22(1):509—520.
[21] 胡俊峰, 張宪民, 徐贵阳. 基于模型预测控制的高速柔性并联机构振动控制[J]. 振动与冲击, 2014, 33(1):24—32.
Hu Junfeng, Zhang Xianmin, Xu Guiyang. Vibration control of a high-speed flexible parallel mechanism based on model predictive control [J]. Journal of Vibration and Shock, 2014, 33(1):24—32.
[22] Canfield R A, Meirovitch L. Integrated structural design and vibration suppression using independent modal-space control[J]. AIAA Journal, 1994, 32(10): 3404—3413.
[23] 陈学前, 汪小华, 阮剑华,等. 两种独立模态空间控制法在柔性结构振动主动控制中的应用研究[J]. 中国科学技术大学学报, 2001, 31(6):700—706.
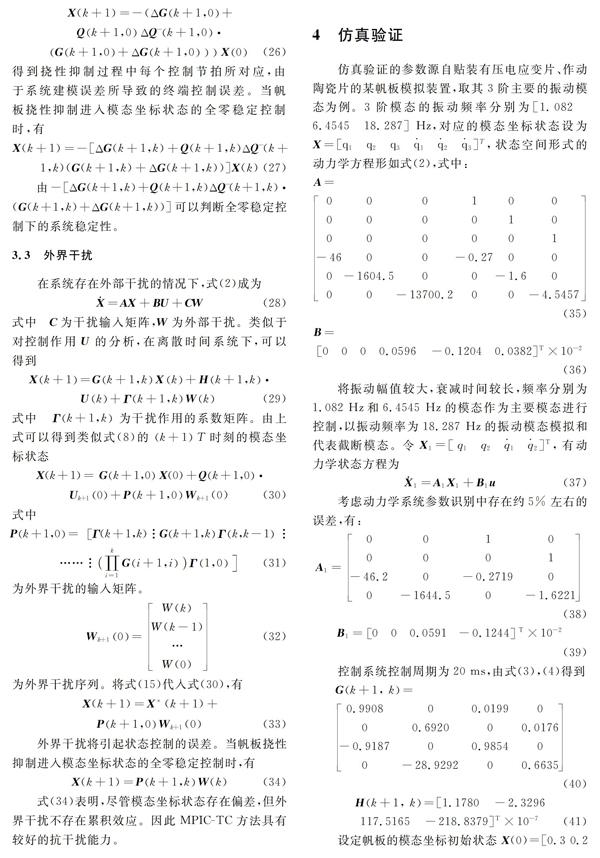
Chen Xueqian, Wang Xiaohua, RUAN Jianhua, et al. Study on two independent modal space control methods in the vibration active control of flexible structure[J]. Journal of University of Science and Technology of China, 2001, 31(6):700—706.
[24] 司洪伟, 李东旭. 大型挠性结构基于独立模态空间的模糊PID控制[J]. 中国空间科学技术, 2005, 25(3):59—65.
Si Hongwei, Li Dongxu. Fuzzy PID vibration control of large flexible structures based on IMSC[J]. Chinese Space Science and Technology, 2005, 25(3):59—65.
[25] 靳永强, 刘向东, 王 伟, 等. 基于模态观测器的挠性航天器姿态控制[J]. 宇航学报, 2008, 29(3):844—848.
Jin Yongqiang, Liu Xiangdong, Wang Wei, et al. Sliding mode attitude control for flexible spacecraft based on modal observer[J]. Journal of Astronautics, 2008, 29(3):844—848.
[26] 王松桂, 杨振海. 广义逆矩阵及其应用[M]. 北京:北京工业大学出版社, 1996:1—3.
[27] 席裕庚. 预测控制[M].第2版.北京: 国防工业出版社, 2013:24—28.
[28] 通雁辉. 挠性航天器的姿态控制及振动抑制研究[D]. 沈阳: 东北大学, 2009.
Abstract: To study vibration suppression of spacecraft flexible solar arrays, based on state transition model of dynamical system in modal space, the evolution law of mode coordinates of solar arrays is solved in discrete time systems. Deviation of mode coordinates is predicted by model prediction method. Generalized inverse method is used to obtain the control sequence needed for vibration suppression. A model predictive and inversive control law for flexible vibration suppression of solar arrays under given time is designed by the method of gradual approximation. Some characteristics of the method in application are discussed. Simulation results show that the proposed method can achieve fast vibration suppression of spacecraft solar panels under specified time constraints.
Key words: solar arrays; vibration suppression; model predictive and inversive control; flexible; timing constraint
作者簡介: 谭天乐(1973-),男,博士,研究员。电话:(021)24183453, 18616017107;E-mail:18616017107@163.com
