Effects of pore structure and distribution on strength of porous Cu-Sn-Ti alumina composites
2017-12-22BioZHAOTinyuYUWenfengDINGXinyingLI
Bio ZHAO,Tinyu YU,Wenfeng DING,*,Xinying LI
aCollege of Mechanical and Electrical Engineering,Nanjing University of Aeronautics and Astronautics,Nanjing 210016,China
bDepartment of Aerospace Engineering,Iowa State University,Ames,IA 50010,USA
Effects of pore structure and distribution on strength of porous Cu-Sn-Ti alumina composites
Biao ZHAOa,Tianyu YUb,Wenfeng DINGa,*,Xianying LIa
aCollege of Mechanical and Electrical Engineering,Nanjing University of Aeronautics and Astronautics,Nanjing 210016,China
bDepartment of Aerospace Engineering,Iowa State University,Ames,IA 50010,USA
Finite element analysis(FEA); Metal-matrix composites(MMCs); Microstructural analysis; Pore structure; Strength
Porous Cu-Sn-Ti alumina composites were fabricated by sintering Cu-Sn-Ti alloy powders,graphite particles,and alumina hollow particles agent.The effects of the pore structure and distribution on the composites strength were evaluated.Different pore distributions were modeled by using finite element analysis to investigate the tensile strength of the composites.Furthermore,a fractal analysis-based box-covering algorithm was used on the Cu-Sn-Ti alumina composites topology graphs to better investigate the pore structure and distribution.Results obtained show that different sizes and concentrations of alumina hollow particles could result in different porosities from 20%to 50%.A larger pore size and a higher pore concentration reduce the strength,but provide more space for chip formation as a bonding material of a grinding wheel.The body-centered pore structure of the composites shows the highest stress under a tension load.The original composites topology graphs have been transformed to ordered distributed pore graphs based on the total pore area conservation.The information dimension magnitude difference between the original topology graphs and the ordered distributed circulars graphs is found to be linear with the Cu-Sn-Ti alumina composites strength.A larger difference renders a lower flexural strength,which indicates that uniform ordered distributed pores could bene fit the composites strength.
1.Introduction
In recent years,Cu-Sn-Ti alloys have been applied widely as bond materials for brazing diamond and Cubic Boron Nitride(CBN)superabrasives onto the surfaces of metallic grinding tools for their high wear resistance and outstanding cutting performance.1–5Furthermore,Shiue et al.6used different hard and blocky particles(TiC,W,Mo,WC,and SiC)to improve the wear resistance of the Cu-Sn-Ti brazing layer,among which TiC particles show an excellent performance over others during the wear test of diamond grinding wheels.Hsieh and Lin7evaluated the wetting behavior of Cu-Sn-Ti on graphite.They found that increasing Ti at 10at%Sn could promote the formation of CuSn3Ti5and SnTi3intermetallic compounds,while increasing Sn at 10at%Ti could promote the formation of Sn3Ti5and Sn3Ti2intermetallic compounds.A high temperature(i.e.,1000°C)was used to induce wetting of Cu-Sn-Ti alloys on graphite.The Cu-Sn-Ti alloys bond microstructure,residual stress,and shear strength of a diamond grinding wheel have been investigated by Buhl et al..8They found that a brazing temperature of 880°C showed an optimal in shear strength(average 320 MPa from a shearing test)and compressive residual stress 350 MPa.Li et al.9found that Ti and Sn atoms semicoherently nucleated and grew on the TiC layer in the form of an interwoven fine lacey structure,with a Cu-based matrix filling the hollow space of the structure.This yields a stronger interfacial interlocking con figuration.Furthermore,Lin et al.10investigated the Cu-Sn-Ti alloys wetting behavior on alumina(Al2O3)surfaces during brazing.It was found that a 9wt%Ti brazed at 900°C showed the best wetting ability to alumina,and a lower residual thermal stress was expected compared to that of Ticusil®alloys.Cu-Sn-Ti alloys have also been used to braze polycrystalline CBN grains onto steel wheels under different temperatures.11,12TiN,TiB2,TiB,and TiAl3have been found to form within the alloys-CBN grain interface,and a good bonding strength has been obtained.
Porous alloys-composites are of great interest as a potential engineering material due to their light weights and high strengths,13–16particularly in grinding wheel bond.They show the superiority in large chip storage space,high wear resistance,and good self-dressing to grind Ti-and Ni-based alloys.Ti-Al micro/nano-sized porous alloys have been developed through the diffusion-rate discrepancy-based Kirkendall effect by He et al..17The Ti-Al porous structure contained pore sizes ranging from 2 to 50 μm with an open porosity from 40%to 60%.They also found that a stable protecting alumina layer over the entire pores surface was formed and the developed Ti-Al porous alloys could be used in aggressive corrosive and rugged environments.Jiang et al.18fabricated porous Ti-Al alloys through a reactive synthesis of Ti and Al powders.They found that the content of Al dominated the formation of pores for different phases(α2-Ti3Al, γ-Ti3Al,and Ti3Al).Krüger and Mortensen19evaluated the interface and mechanical properties of Cu-8wt%Al-Al2O3on Ti contents.It was found that a 0.2wt%Ti showed the best mechanical properties for the interface between the Cu alloy and Al2O3particles.Ti3(Cu,Al)3O has been found to form with an increase of Ti at 2wt%.
Recently,alumina hollow particles have been used as a pore-forming agent to develop porous Cu-Sn-Ti alumina composites for brazing.20,21It was found that the bond maintained a high strength attributing to the formation of stable protecting layers between Cu-Sn-Ti composites and CBN grains,as well as Cu-Sn-Ti composites and alumina hollow particles.A good grinding performance has been observed.Chen et al.22used 0.5 mm size alumina hollow particles to fabricate a Cu-Sn-Ti alloys-bonded CBN grinding wheel with a bending strength of 60 MPa and 30%porosity.The grinding forces,speci fic grinding energy,and temperatures of the porous bonded CBN wheels were found lower than those of vitrified bonded CBN wheels.Ma et al.23investigated experimentally the effect of alumina hollow particles on the microstructures and mechanical strengths of porous Cu-Sn-Ti alumina composites.It was found that,by altering the alumina hollow particles size and distribution,the pore size and distribution could be controlled during the fabrication process.A balance between mechanical strength and high porosity has been achieved experimentally.
It is noted that,although important progress has been made,a quantitative analysis of the effect of the pore structure and distribution on the flexural strength of Cu-Sn-Ti alumina composites has barely been carried out,which has a limitation on the improvement of this new type of porous grinding wheels bonding.On the other hand,owing to the development of a fractal analysis-based box-covering algorithm in analyzing planar networks,the classical information dimension is proposed to characterize the fractal dimension of the entire area,and quantitative analysis results could be obtained.24–27Ferna´n dez-Martı´nez and Sa´nchez-Granero28studied the relationship and mathematical properties of the Hausdorff dimension from both continuous and discrete views to analyze the fractal dimension.Li et al.29proposed an efficient box-coveringbased approach to estimate the fractal dimension analysis accuracy.It was found that the improved boxing-covering method showed more efficient in characterizing natural images and could be more useful in image categorization.Wei et al.30provided a new information dimension method of complex networks to study fractal and self-similarity properties of planar networks.The pore structure and distribution are complex and have been further analyzed with the fractal analysis-based box-covering algorithm in the current study.
In this paper,the effect of the pore structure and distribution on the flexural strength of porous Cu-Sn-Ti alumina composites has been investigated.Alumina hollow particles with different sizes and concentrations have been used.The fractal analysis-based box-covering algorithm method has been used on metallographic images to better describe the porosity formation mechanics,dimension,and distribution.Furthermore,the in fluence of the pore size and concentration on the flexural strength has been discussed.The pore distribution uniformity has been quantitatively analyzed with the fractal analysisbased box-covering algorithm.Finally,a quantitative relationship between pore characteristics and the composites strength has been determined.
2.Experimental procedures
2.1.Cu-Sn-Ti alumina porous composites sintering
The materials used for production of composites are(A)Cu-Sn-Ti alloy powder((Cu-18wt%Sn-10wt%Ti)),(B)graphite particles,and(C)alumina hollow particles(closed pore former).In Fig.1(a),the microstructure of the Cu-Sn-Ti alloy powder before sintering has been analyzed by using a Scanning Electron Microscope(SEM,Hitachi S-3400).It shows both spherical and irregular particles,for example,A and B respectively.Both points are analyzed by using Energy-Dispersive X-ray Spectrometry(EDS).The results are displayed in Fig.1(b)and(c)respectively,which show that the compositions in the spherical particles are Cu and Sn with the same extent(Cu-20wt%Sn alloy)and the irregular ones are Ti particles.

Fig.1 Microstructure and EDS of Cu-Sn-Ti alloy powders.
In addition,10wt%graphite particles have been added to strengthen composites and avoid a collapse during a hightemperature sintering.Alumina hollow particles(Fig.2)with a diameter of 0.25–0.60 mm are used as closed pore former for the formation of porous Cu-Sn-Ti alumina composites.The microstructure of the fractured alumina hollow particles(0.25–0.30 mm)wall is shown in Fig.3(a).The EDS results show that the wall composition is Al2O3with high peak values of Al and O elements,as shown in Fig.3(b).A wall thickness of 22 μm renders a 70vol%porosity in the alumina hollow particles.Meanwhile,an increase in the wall thickness with the diameter of particles has been observed from SEM images,32 μm for 0.35–0.45 mm particles and 45 μm for 0.50–0.60 mm particles,respectively.Since the wall is very thin and during the sintering process,the alumina can also diffuse into the composites matrix,thus the pore size of the composites is assumed the same as that of the alumina hollow particles.The primary content and physical properties of the alumina hollow particles are shown in Table 1.
In this study,the sizes of Cu-Sn-Ti alloy powders and graphite particles are both less than 50 μm.Porous Cu-Sn-Ti alumina composites are fabricated by cold pressing with Cu-18wt%Sn-10wt%Ti alloy powders,graphite particles,and alumina hollow particles,followed by a sintering process in a VAF-20 vacuum furnace at a temperature of 880°C for 30 min.The heating and cooling rates are controlled at 10°C/min with a pressure of about 10-2Pa.
Compared to the closed pores produced by alumina hollow particles during the sintering process,the micropores created by the flowing and contraction of the Cu-Sn alloy are quite small and has been neglected in the current study.
The porosity induced by alumina hollow particles can be written as follows:

where

in which Vbis the composites bulk volume,and Vpand mpare the total volume and weight of the alumina hollow particles additive,respectively.The average density of alumina hollow particles ρphas been measured by using a pycnometer as 1.72,1.58,and 1.43 g/cm3for 0.25–0.30 mm,0.35–0.45 mm,and 0.50–0.60 mm,respectively.The porosity of the Cu-Sn-Ti alumina composites η can be determined by the weight and density of the alumina hollow particles.From this,substitute Eqs.(2)to(1)and obtain

Different sizes and concentrations of alumina hollow particles have been used for the formation of porous Cu-Sn-Ti alumina composites.Table 2 shows the formed composites with different alumina hollow particles and different porosities.A maximum 50%porosity has been created with 0.25–0.30 mm alumina hollow particles.

Fig.2 Different sizes of alumina hollow particles used.

Fig.3 Microstructure and EDS of alumina hollow particles.

Table 1 Primary content and physical properties of alumina hollow particles.

Table 2 Samples with different alumina hollow particles sizes and concentrations.
2.2.Flexural strength test
The strength of sintered composites can be described by a three-point bending test,an assay method for the transverse rupture strength of sintered metal composites.31This strength is closely associated with the level of porosity and inhomogeneity of composites.
The flexural strength of different samples σflsis estimated by the following equation:

where F is the critical load for fracture;L is the loading effective length,25 mm used in the current experiment;b is the width of specimens;h is the thickness of specimens.
It can be observed from the load-displacement curves(Fig.4)that during the test,a larger pore size and a higher pore concentration will induce a lower critical load,and the minimum load is about 0.4 kN.Here,the loading rate is fixed at 1 mm/min.
3.Results
3.1.Microstructure and characterization of Cu-Sn-Ti alumina porous composites

Fig.4 Load-displacement curves during the 3-point bending test for sample Nos.1–6.
Fig.5(a)shows the microstructure SEM image of the composites.The schematic microstructure of porous alloys is shown in Fig.5(b),and the large lamellar graphite can be observed with small alumina hollow particles bonded by the Cu-Sn-Ti alloy.Meanwhile,the pores tend to form at the interface between the large lamellar graphite and the binding alloy,as displayed in Fig.5(c).As seen from Fig.5(d),the concentrations of the Ti element on both sides of the graphite particle are high,which has been tested using EDS with a line scan at the bonding interface.This shows that the addition of graphite particles facilitates the formation of pores in the composites by(A)providing cracks/pores generation along the graphite particles boundary and/or(B)accelerating the diffusion of alumina into the composites matrix.
The topological map of the composites has been achieved by using a KH-7700 optical microscope after a polishing process.As shown in Fig.6(a),if no alumina hollow particles have been used,only small pores are formed from the powder sintering process.The binarization image converted from the original image is shown in Fig.6(b)for further analysis.The pore size distribution is obtained by analyzing the white pixels in Fig.6(b),as shown in Fig.7.It indicates that the fraction of micro-pores(d=0–50 μm)is more than 80%,and less than 1%of the pores are over 100 μm size.Thus,compared to alumina hollow particles-induced large pores,the sintering process-induced pores could be neglected in the current study.
Fig.8 shows the original images and their corresponding binarization images for different sizes of alumina hollow particles Samples 2,5 and 6.It can be observed that the pore size increases with an increase of the alumina hollow particles size and the same composites porosity of 20%.This shows that the pore size is controlled by the alumina hollow particles size during the sintering process,and the porous composites structure is controllable.Fig.9 shows the original images and their corresponding binarization images for different porosity Samples 2,3 and 4 of the same alumina hollow particles size.It shows that for the same size of the alumina hollow particles,the pore size increases with an increase of the pore concentration since the coalescence happens among the pores during the sintering process.The pore size distributions of Figs.8 and 9 are plotted in Fig.10(a)and(b),respectively.If the concentration of porous alloys is fixed at 20%,the pore size distribution is shown in Fig.10(a).It reveals that the peak value and majority of the pore size are consistent with the size of alumina hollow particles.As seen from Fig.10(b),if the pore size is fixed at 0.25–0.30 mm,the peak value and majority of the pore size are the same.The results show the consistency between the pore size distribution and the size of alumina hollow particles.Consequently,the pore size and distribution of the composites can be controlled by selecting an appropriate size and concentration of alumina hollow particles.
The relationships between the flexural strength and the pore size and porosity are shown in Fig.11,which shows that when the porosity increases from 0 to 50 vol%,the flexuralstrength ofporousCu-Sn-Tialumina composites decreases.The larger the size of alumina hollow particles is,the lower the flexural strength exhibits.When the porosity reaches 50%,the strength of porous composites drops by 40%to about 60 MPa compared to that of alumina hollow particles-free composites.

Fig.5 Surface morphology of porous Cu-Sn-Ti alloy alumina composites.

Fig.6 Sample 1 topology with micropores from the sintering process.

Fig.7 Pore size distribution for Sample 1 without alumina hollow particles.
3.2.Finite element analysis of different pore distributions on the porous composites strength
Finite element analysis has been used in this section to investigate the relationship between the pore distribution and the maximum stress within the structure under a tension load,which also provides a guidance to better understand the porous composites performance in addition to the bending tests.Sketches of porous composites with different pore distributions are shown in Fig.12.In all three cases,the left side of an element is fixed with 4 corner pores;in Fig.12(a),there are four edge pores in the middle of a distance p from the left;in Fig.12(b),there are two face-centered pores of a distance p from the left;in Fig.12(c),there is a body-centered pore.
The porosity of each element model is the same and has the following form:

Fig.8 Topologies of Samples 2,5 and 6.

Fig.9 Topologies of Samples 2,3 and 4.

Fig.10 Pore size distributions for different samples.

Fig.11 Effects of porosity and pore size on flexural strength of porous Cu-Sn-Ti alumina composites.

where p is the horizontal distance of the adjacent pore,1 mm used in this simulation.r is the radius of pores.
An isotropic material model and a steady state condition are used in an ABAQUS 6.10 environment.A pore size of 500 μm is chosen and a tension load is applied on the right sides of the models.An elasticity module E=700 GPa and Poisson’s ratio υ=0.3 have been used.Fig.13 shows the simulation result of Mises stress,and the corresponding maximum Mises stress σmaxis shown in Fig.14.
The results show that the body-centered pore model has the highest stress since this model has the most nonuniformity and induces the most stress concentration.Compared to the other two models,the edge pores model has a 5%–10%stress relief and is the most uniform con figuration.The results obtained reveal that a uniform pore distribution will attribute to the porous composites strength.
3.3.Fractal analysis-based box-covering algorithm for information-dimension analysis
From Section 3.1,it is known that the porosity and flexural strength of the porous composites are closely associated.Moreover,from Section 3.2,the FEA results show that the nonuniformity of the pores has a signi ficant in fluence on the porous composites strength.To further investigate the pore uniformity and distribution effect in a bulk composites scale,a fractal dimension analysis method has been used.In this study,information dimension is applied to evaluate the pore distribution uniformity of the porous Cu-Sn-Ti alumina composites with different sizes and concentrations of alumina hollow particles.This probability method applies the boxcovering algorithm to scan the whole binarization image by various sizes of squares.
The ration number of the white squares is given as

where N is the square size(in pixel).ni(N)is the number of white pixels in each covering square.Qi(N)is the total number of pixels in the covering square.The total information of a certain covering square N can be provided as

where MNis the number of squares.

Fig.14 In fluence of pore distribution on the maximum stress revealing an increase of the maximum stress with pore distribution nonuniformity.

Fig.12 Schematics of pore models for porous composites.

Fig.13 Stress distributions in composites of different pore models.
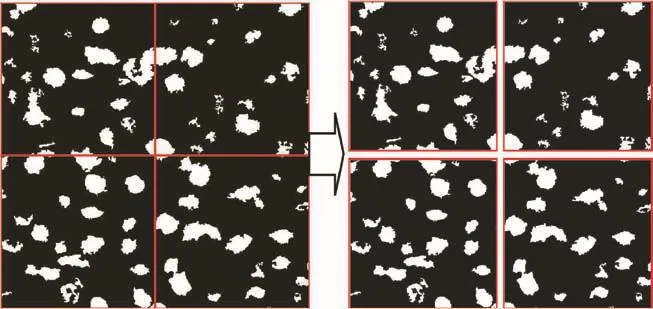
Fig.15 Binarization and segmentation images of Sample 4 showing four covering boxes(N×N=512 pixel×512 pixel).

Fig.16 Pore distributions of original pores and ordered pores of Sample 4 with approximately the same total white pixels.

Table 3 Values of lnI(N)and DIof porous alloy with different sizes of alumina hollow particles.
The value of information dimension DIof a certain covering square N is

The relationship between I(N)and N can be expressed as the following equation:

where c is a constant.
Eq.(9)can be rewritten in the form of

For different square sizes N used in the analysis,the value of information dimension DIcan be obtained from the slope of ln I(N)and ln(1/N)as

The values of information dimension DIof the original images and of the manipulated ordered pore images are compared to investigate the pore uniformity effect.
4.Discussion
In the current study,the steps of fractal analysis are as follows:(A)a sobel operator-based segmentation algorithm has been used to set a binarization image as 1024 pixel×1024 pixel32;(B)the square sizes N used are 512,256,128,64,32,16,and 8,respectively.An example of N=512(binarization image of Sample 4)is shown in Fig.15.It renders four covering boxes where MN=4.After all different square sizes N have been calculated,the value of information dimension DIcan be obtained,which contains the total information of white pixels distribution and concentration.
In order to investigate the pore distribution effect on the composites strength,the pores are standardized and ordered as shown in Fig.16(b).a reveals the space between the ordered pores.Compared to Fig.16(a),the total white pixels of the pores are kept the same.In particular,the ordered pores size is selected consistently as the average size of alumina hollow particles,for example,0.275 mm for the 0.25–0.30 mm particles.The distances between two pores and the edge pore position are shown in Fig.16(b),and then totally 49 pores are analyzed in this sample.
The values of lnI(N)and ln(1/N)have been calculated and tabulated in Table 3 for different samples.From Eq.(11),least square linear fitting is used to calculate the value of information dimension DI.The value of information dimensionof ordered pore images and the difference between DIandare also tabulated in Table 3.The range of DIof different samples is from 1.327 to 1.495.Fig.17(a)shows linear fitting for each case,while Fig.17(b)shows the values of DIfor different samples.The trend of the value of information dimension DIincreases with a decrease of the pore size and an increase of the porosity.As a result,the maximum DIoccurs at the smallest size of alumina hollow particles(0.25–0.30 mm)and the largest porosity(50 vol%).

Fig.17 Fractal analysis for different samples revealing that the maximum information dimension occurs at Sample 4.

Fig.18 Information dimensions of different distributions and in fluence of D0on flexural strength.
The DIandvalues are plotted in Fig.18(a),in which they present a similar trend,buthas a higher value.The difference between DIandhas been shown with the flexural strength σfls,as displayed in Fig.18(b),and a clear linear relationship shows up as

The information dimension difference D0represents a degree of nonuniformity of the pore distribution in the porous Cu-Sn-Ti alumina composites.It shows that the higher the degree of nonuniformity is,the lower the flexural strength σflsis.Several factors can induce the pore nonuniformity,namely(A)pore coalescence-induced local defect cluster,(B)graphite particles nonuniformity-induced pore growth,(C)alumina hollow particles non-uniform distribution,and(D)uneven sintering process.As known,a higher porosity will provide more space to contain chips from the grinding process.However,for the sample pore size and porosity concentration,a uniform pore distribution can bene fit the Cu-Sn-Ti alumina composites flexuralstrength,and theperformancewillbefurther investigated.
5.Conclusions
In the current study,new porous Cu-Sn-Ti alumina composites have been fabricated by sintering Cu-Sn-Ti alloy powders,graphite particles,and alumina hollow particles as a poreforming agent.The microstructure,elemental composition,and topology-based pore structure and distribution have been investigated.Several interesting findings have been found as follows:
(1)Different sizes and concentrations of alumina hollow particleswillresultin differentporesizes(0.25–0.60 mm)and porosities from 20%to 50%in the Cu-Sn-Ti alumina composites.
(2)The effects of the pore structure and distribution on the lf exural strength of the composites show that a larger pore size and a higher pore concentration will reduce the composites strength(106–61.12 MPa),but will provide more space for chip formation as a bonding material during a grinding process.
(3)Finite element analysis shows that the body-centered pore structure presents the highest stress under a tension load using an isotropic material model with a pore size of 500 μm.The tensile strength increases with the composites uniformity attributing to the porous composites strength.
(4)A fractal analysis-based box-covering algorithm has been used to investigate the in fluences of the pore structure and distribution on the composites flexural strength.The linear relationship between the information dimension difference D0and the composites flexural strength σflsshows that the uniformity of the pore distribution will bene fit the porous Cu-Sn-Ti alumina composites flexural strength,and the performance will be further investigated.
In general,the current work will provide a better guidance to use porous Cu-Sn-Ti alumina composites as a bonding material for grinding wheels and the fractal analysis method can be used as an effective tool to research porous material topology.
Acknowledgements
This work was financially co-supported by the National NaturalScienceFoundation ofChina(Nos.51235004and 51375235)and the Fundamental Research Funds for the Central Universities(Nos.NE2014103 and NZ2016107).
1.Onishi H.Fabrication of new porous metal-bonded grinding wheels by HIP method and machining electronic ceramic.J Porous Mater 1997;4(3):187–98.
2.Li X,Ma S,Meng FJ.Surface integrity of GH4169 affected by cantilever finish grinding and the application in aero-engine blades.Chin J Aeronaut 2015;28(5):1539–45.
3.Tomino H,Tsukuda A,Kondo Y.In fluence of porosity on grinding performance of porous cast-iron bonded diamond grinding wheel made by pulse current sintering method.J Japan Soc Powder Powder Metall 1999;46(3):257–61.
4.Zhang ZY,Huo FW,Wu YQ,Huang H.Grinding of silicon wafers using an ultra fine diamond wheel of a hybrid bond material.Int J Mach Tools Manuf 2011;51:18–24.
5.Hasuda Y,Handa A,Kobori Y,Kinebuchi S,Furusawa T,Harigaya Y.Grinding of superalloys using metal-bonded CBN wheel.Key Eng Mater 2012;523–524:143–8.
6.Shiue RK,Buljan ST,Eagar TW.Abrasion resistant active braze alloys for metal single layer technology.Sci Technol Weld Join 1997;2(2):71–8.
7.Hsieh YC,Lin ST.Microstructural development of Cu-Sn-Ti alloys on graphite.J Alloys Comp 2008;466(1):126–32.
8.Buhl S,Christian L,Ralph S,Konrad W.Microstructure,residual stresses and shear strength of diamond-steel-joints brazed with a Cu-Sn-based active filler alloy.Int J Refract Met Hard Mater 2012;30(1):16–24.
9.Li WC,Lin ST,Liang C.Interfacial segregation of Ti in the brazing of diamond grits onto a steel substrate using a Cu-Sn-Ti brazing alloy.Metall Mater Trans A 2002;33(7):2163–72.
10.Lin CC,Chen RB,Shiue RK.A wettability study of Cu/Sn/Ti active braze alloys on alumina.J Mater Sci 2001;36(9):2145–50.
11.Li BK,Li CH,Zhang YB,Wang YG,Jia DZ,Yang M.Grinding temperature and energy ratio coef ficient in MQL grinding of hightemperature nickel-base alloy by using different vegetable oils as base oil.Chin J Aeronaut 2016;29(4):1084–95.
12.Ding WF,Xu JH,Chen ZZ,Miao Q,Yang CY.Interface characteristics and fracture behavior of brazed polycrystalline CBN grains using Cu-Sn-Tialloy.MaterSciEng:A 2013;559:629–34.
13.Neslusan M.Grinding of Ni-based alloys with grinding wheels of high porosity.Adv Prod Eng Manage 2009;4(1–2):29–36.
14.Mao C,Zhou X,Yin LR,Zhang MJ,Tang K.Investigation of the lf ow field for a double-outlet nozzle during minimum quantity lubrication grinding.Int J Adv Manuf Technol 2016;85:291–8.
15.Su HH,Xu HJ,Xiao B.Microstructure and performance of porous Ni-Cr alloy bonded diamond grinding wheel.Mater Sci Forum 2006;532:373–6.
16.Cai R,Rowe WB,Morgan MN.The effect of porosity on the grinding performance of vitri fied CBN wheels.Key Eng Mater 2009;238–239:295–300.
17.He YH,Jiang Y,Xu NP,Zou J,Huang BY,Liu CT,et al.Fabrication ofTi-Almicro/nanometer-sized porousalloys through the Kirkendall effect.Adv Mater 2007;19(16):2102–6.
18.Jiang Y,He YH,Xu NP,Zou J,Huang BY,Liu CT.Effects of the Al content on pore structures of porous Ti-Al alloys.Intermetallics 2008;16(2):327–32.
19.Krüger C,Mortensen A.In filtrated Cu8Al-Ti alumina composites.Composites Part A 2014;66:1–15.
20.Ding WF,Xu JH,Chen ZZ,Yang CY,Song CJ,Fu YC.Fabrication and performance of porous metal-bonded CBN grinding wheels using alumina bubble particles as pore-forming agents.Int J Adv Manuf Technol 2013;67(5–8):1309–15.
21.ZhangDK,LiCH,JiaDZ,ZhangYB,ZhangXW.Speci ficgrinding energyandsurfaceroughnessofnanoparticlejetminimumquantity lubrication in grinding.Chin J Aeronaut 2015;28(2):570–81.
22.Chen ZZ,Xu JH,Ding WF,Ma CY.Grinding performance evaluation of porous composite-bonded CBN wheels for Inconel 718.Chin J Aeronaut 2014;27(4):1022–9.
23.Ma CY,Ding WF,Xu JH,Fu YC.In fluence of alumina bubble particles on microstructure and mechanical strength in porous Cu-Sn-Ti metals.Mater Des 2015;65:50–6.
24.Florindo JB,Backes AR,Castro MD,Bruno OM.A comparative study on multiscale fractal dimension descriptors.Pattern Recogn Lett 2012;33(6):798–806.
25.Liang XH,Lin B,Han XS,Chen SG.Fractal analysis of engineering ceramics ground surface.Appl Surf Sci 2012;258(17):6406–15.
26.Wei DJ,Liu Q,Zhang HX,Hu Y,Deng Y,Mahadevan S.Boxcovering algorithm for fractal dimension of weighted networks.Scient Rep 2013;3:3049.
27.Zhang HS,Li YZ,Wang SN,Liu Y,Zhang ML.Ground experimental investigations into an ejected spray cooling system for space closed-loop application.Chin J Aeronaut 2016;29(3):630–8.
28.Ferna´ndez-Martı´nez M,Sa´nchez-Granero MA.Fractal dimension for fractal structures:a Hausdorff approach revisited.J Math Anal Appl 2014;409(1):321–30.
29.Li J,Du Q,Sun CX.An improved box-counting method for image fractal dimension estimation.Pattern Recogn 2009;42(11):2460–9.
30.Wei DJ,Wei B,Hu Y,Zhang HX,Deng Y.A new information dimension of complex networks.Phys Lett A 2014;378(16–17):1091–4.
31.Cha SI,Lee KH,Ryu HJ,Hong SH.Effect of size and location of spherical pores on transverse rupture strength of WC-Co cemented carbides.Mater Sci Eng A 2008;486(1–2):404–8.
32.Jin S,Kim W,Jeong J.Fine directional de-interlacing algorithm using modi fied sobel operation.IEEE Trans Consum Electron 2008;54(2):857–62.
14 March 2017;revised 3 May 2017;accepted 13 May 2017
Available online 9 September 2017
Ⓒ2017 Chinese Society of Aeronautics and Astronautics.Production and hosting by Elsevier Ltd.This is an open access article under the CCBY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).
*Corresponding author.
E-mail address:dingwf2000@vip.163.com(W.DING).
Peer review under responsibility of Editorial Committee of CJA.
杂志排行
CHINESE JOURNAL OF AERONAUTICS的其它文章
- A general method for closed-loop inverse simulation of helicopter maneuver flight
- Numerical simulation of a cabin ventilation subsystem in a space station oriented real-time system
- Parametric analyses on dynamic stall control of rotor airfoil via synthetic jet
- Effect of particle size and oxygen content on ignition and combustion of aluminum particles
- Effects of axial gap and nozzle distribution on aerodynamic forces of a supersonic partial-admission turbine
- Effect of a transverse plasma jet on a shock wave induced by a ramp
