表面活性剂对树形银纳米结构影响的蒙特-卡洛模拟

王元元　徐群星　谢华清　吴子华　邢姣娇

(上海第二工业大学环境与材料工程学院，上海201209)

表面活性剂对树形银纳米结构影响的蒙特-卡洛模拟

王元元徐群星谢华清*吴子华邢姣娇

(上海第二工业大学环境与材料工程学院，上海201209)

采用偏压受限扩散聚集模型研究溶液中银树形纳米结构的生长。模拟中，在二维正方形格子中引入了等腰直角三角形粒子进行模拟，同时运用不同的粘贴概率来描述表面活性剂的效果。模拟结果表明树形纳米结构随着偏压的增大而变得更密。表面活性剂的加入使得树形纳米结构变得更加对称和规则。更进一步，当表面活性剂的效果足够强且外加偏压很小的时候，银纳米颗粒聚集成了银纳米片。模拟结果有利于定性解释相关的实验结果。

蒙特-卡洛模拟；树形银纳米结构；表面活性剂

In this paper,we investigate the growth of the silver dendritic nanostructures with the surfactants by applying the biased diffusion-limited aggregation(DLA)method25,26.To study the influence of the surfactant on the morphology of the dendritic nanostructures,the isosceles right-angled triangle particles are applied in the two-dimensional(2D)square grids and the sticking possibilities of different particle sides are introduced.

2　Simulation method

The growth of the dendritic nanostructure is an out-of-equilibrium process.As we know,when a bias voltage is applied,a local space charge and thus a large electric field are formed in the vicinity of the negative electrode12.This space charge area is the diffusion layer and it thickens with the deceasing ion concentration18.At first,Ag+moves freely and performs random Brownian motion and walks to the diffusion layer randomly.When Ag+moves into the diffusion layer,it will be driven to move toward the reaction surface by the electric field in the diffusion layer.When Ag+moves to the reaction surface,it gains an electron and is reduced to a silver atom.Then the reduced silver atom deposits on the reaction surface.We apply the 2D biased DLA model27to simulate the growth of the silver dendritic nanostructures in the silver nitrate solution.When the area of the reaction plane is large enough and the isotropy holds true in the plane,the threedimensional(3D)problem can be simplified to the quasi-2D problem in the plane perpendicular to the reaction plane(see Fig.1 (a)).At this time,the 2D simulation is acceptable.It is also noted that extending the DLA simulation to 3D is relatively straightforward but there are some important differences and options not available in two dimensions28,29.One of the significant improvements of the 3D algorithm is not to form the DLA on a grid of finite resolution but rather on a continuum.A particle adheres to the existing structure if it comes within some minimum distance of any part of the existing structure.In our simulation,a particle denotingAg+is released at a random site on the top horizontal line. This particle performs a bias random walk until it reaches the bottom horizontal line and then deposits.The next particle is then released,and so on.Two velocities are introduced to describe the particle motion:longitudinal velocity vyand the transverse velocity vx,which are perpendicular and parallel to the bottom line,respectively18.The velocity ratio between vyand vxis denoted as p (p=vy/vx).The longitudinal velocity is determined by the electric field in the diffusion layer,which is affected by the bias voltage. The transverse velocity is determined by the Brownian motion, which is only related to the solution temperature.In the simulation,we need to consider the effect of the surfactant.Since the capping agents change the free energies for different crystallographic planes and thus their relative growth rates24,we apply the square and isosceles right-angled triangle particles(see Fig.1(b)) instead of the round particles applied in the literature18,so that we can distinguish different sides of the particles.It should be noticed that there are four types of triangle particles(see Fig.1(b))in the 2D square grids when the rotation of the particles is neglected.In order to simulate different growth rates of different sides,the sticking rates26,30of different sides are introduced:The sticking rates for the two arms of the right angle and the hypotenuse of the triangle particles are paand phrespectively,while for the sides for the square particles,the sticking rates are all pe.When a particle walks to the neighbor position of one side with the sticking rate pi(i=a,h,e),it has a pipossibility to be stuck and 1－pipossibility to continue walking.Introducing the sticking possibility is actually adding an inhomogeneous perturbation,which weakens the screening effect3and makes the grown nanostructures become much regular and compacter.

3　Results and discussion

We now study how the morphology of the dendritic nanostructures changes with the bias voltage and the surfactant.Biased DLA Monte-Carlo method27is applied to obtain the simulation images.To compare the results simulated in different conditions, the image sizes are all 100×300 grids.

3.1Effect of bias voltage on growth

Fig.1　Schematic diagram of biased DLAmodel

We first study the influence of the voltage on the growth.The results with square and triangle particles applied are shown in Fig.2 and Fig.3,respectively.The results of(a),(b)and(c)in Fig.2 and Fig.3 are obtained with p=0.25,1,4,which means that the longitudinal velocity increases induced by the increasing bias voltage.It is seen that when the bias voltage is small,the fractal trees are separate without overlapping each other(see Fig.2(a)and Fig.3(a)),no matter that the square or the triangle particles are applied.Then the dendritic nanostructures become denser with the increasing voltage.When the bias voltage is large enough(see Fig.2(c)and Fig.3(c)),the fractal trees connect together and jointinto a whole shape in which single trees cannot be separated out. At the same time,the sizes of branches decrease with the increasing voltage.This can be understood as follows.When p is small,which means that vxis larger than vy,each particle tends to be captured by the tip of the branches,instead to aggregate on the hollow sites.However,when p is large,which means that vyis larger than vx,the particle has much larger possibility to walk into the hollow position of the branches.Therefore,the bias voltage affects the walk property in the diffusion layers and thus modifies the whole morphology of the dendrites.It is also noticed that, although the simulation results with both square and triangle particles have similar features,the simulation images with the square particles are very rough in comparison with those with the triangle particles.This is because the area of the square particle is larger than that of the triangle particle.Considering that the size of the 2D grids is keeping the same,the larger the particles are,the rougher the simulation images are.

Fig.2　Simulation images with the square particles applied

Fig.3　Simulation images with the triangle particles applied

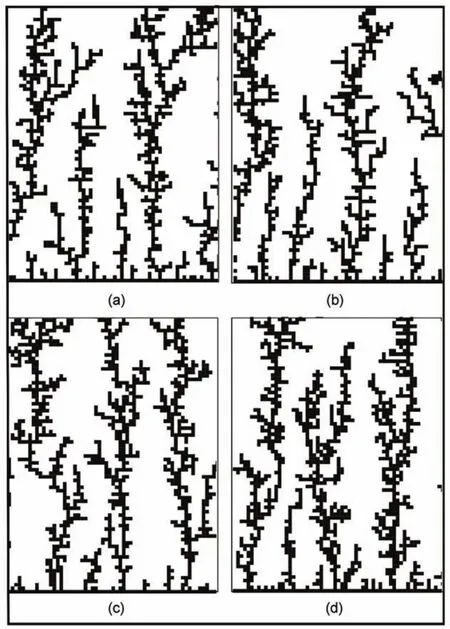
Fig.4　Simulation images with the square particles applied

Fig.5　Simulation images with the triangle particles applied for the equivalent sticking possibilities of the right-angle sides and the hypotenuse

3.2Effect of surfactant on the growth

We now turn to investigate the effect of the surfactant to the morphology of the silver dendritic nanostructures.The results with the square particles applied are shown in Fig.4.In the simulation, p=vy/vx=1.The sticking possibilities decrease gradually(pe=0.8, 0.6,0.4,0.2)for Fig.4(a)to(d),which implies that the growth rate becomes smaller and the effect of the surfactant becomes stronger. The simulation results in the four images do not have large dif-ference,except that the concentration of the branches increases a little.This comes from the fact that the four sides of the square are equivalent.The decrease of the growth rates of the four sides simultaneously can only lengthen the walking of the particles, which makes the particles prefer to walk toward the hollow of the branches.Therefore,changing the sticking possibilities of the four equivalent sides do not affect the whole morphology of the dendritic nanostructures strongly.This modification of the DLA model is similar to the multiple hits method.Therefore,it is not satisfying to explain the effect of the surfactant by applying the square particles in the modified DLAmodel.

In the following,we introduce the triangle particles in the DLA model.The sticking possibilities of the right-angle sides and the hypotenuse are set to be the same(pa=ph)and the results are shown in Fig.5,where Fig.5(a－d)are corresponding to pa=ph= 0.8,0.6,0.4,0.2,respectively,which means that the effect of the surfactant increases.It is seen that the morphology of the deposit changes strongly with the decrease of the sticking possibility due to the inequality of the three sides,although the sticking possibilities of all the sides are the same.When the effect of the surfactant is weak(Fig.5(a)),the grown structures is still fractal dendrites,which is consistent with the observation in almost every experimental attempt for silver dendrites31－34.When the effect of the surfactant increases,the morphology becomes regular and ordered.When the effect of the surfactant is strong enough(Fig.5 (d)),the fractal trees are joint into some pieces.This result can also be seen in the experimental work35,where 1.1 mmol CTAB in 15 mL water is used as the surfactant.This is because when the sticking possibilities decrease due to the effect of the surfactant, every particle has large possibility to escape from the site where it touches the deposit at the first time and continue walking. Therefore,the possibility it walks into the hollow of the branches increases,which makes the nanostructures become much denser.

We then continue to present the results when the sticking possibilities of the right-angle sides and the hypotenuse are different,which is in accordance with the effect of the surfactant in experiments.In Fig.6,the results when the surfactant only affects the hypotenuse of the triangle are shown,where ph=0.8,0.6,0.4, 0.2 for Fig.6(a),(b),(c),and(d),which implies that the effect of the surfactant increases gradually.At the same time,pa=1 and p= 1.It is seen that although phdecreases gradually,the dendritic structures do not have large difference.This implies that the surfactant that affects the growth rate of the hypotenuse does not influence the morphology strongly.Furthermore,we consider the cases when the surfactant only affects the right angle sides and the results are shown in Fig.7.In the simulation ph=1 and padecreases gradually.It is interesting that the fractal trees gradually joint together and become plates.It is also noticed that the results in Fig.7 are similar to those in Fig.5.It indicates that the surfactant that affects the growth rate of the right angle sides is in the leading role to modify the morphology of the dendrites.This can be understood as follows.There are two arms of the right angle,whereas only one hypotenuse in one triangle particles.When a particle touches the deposit,the possibility that the interface is the right-angle side is larger.Therefore,it is more efficient to change the sticking possibilities of the right angle sides than the hypotenuse.Our simulation results imply that different surfactants which influence different crystal faces have different effects on the morphology of the dendrites.

Fig.6　Simulation images with the triangle particles applied when the surfactant only affects the hypotenuse of the triangle

Fig.7　Simulation images with the triangle particles applied when the surfactant only affects the right angle sides

4　Conclusions

Inconclusion,wehaveinvestigatedthegrowthofthedendritic nanostructures.By introducing the isosceles right-angled triangle particles in the 2D square grids and the sticking possibilities of differentsidesoftheparticle,amodifiedbiasedDLAmodelisset up and applied to study the effect of the bias voltage and the surfactant to the morphology of the fractal trees.It is found that the dendriticnanostructuresbecomedenserandthesizesofthesingle branches decrease with the increasing bias voltage.What is interestingisthatthefractaltreesjointtogetherandbecomeplatesdue to the surfactant,which implies that the surfactant can make the structuresinthewholebecomemuchmoreregularandsymmetrical.

References

(1) Tarascon,J.M.;Armand,M.Nature 2001,414,359. doi:10.1038/35104644

(2) Shi,F.;Song,Y.;Niu,J.;Xia,X.;Wang,Z.;Zhang,X.Chem. Mater.2006,18,1365.doi:10.1021/cm052502n

(3) Vicsek,T.Fractal Growth Phenomena;World Scientific: Singapore,1992.

(4) Fang,J.X.;Ding,B.J.;Song,X.P.;Han,Y.Appl.Phys.Lett. 2008,92,173120.doi:10.1063/1.2888770

(5) Xiao,J.P.;Xie,Y.;Tang,R.;Chen,M.;Tian,X.B.Adv.Mater. 2001,13,1887.doi:10.1002/1521-4095(200112)13:24<1887:: AID-ADMA1887>3.0.CO;2-2

(6)Zheng,X.J.;Jiang,Z.Y.;Xie,Z.X.;Zhang,S.H.;Mao,B.W.; Zheng,L.S.Electrochem.Commun.2007,9,629.doi:10.1016/ j.elecom.2006.10.039

(7) Gutés,A.;Carraro,C.;Maboudian,R.J.Am.Chem.Soc.2010, 132,1476.doi:10.1021/ja909806t

(8)Wang,M.;Zhong,S.;Yin,X.B.;Zhu,J.M.;Peng,R.W.; Wang,Y.;Zhang,K.Q.;Ming,N.B.Phys.Rev.Lett.2001,86, 3827.doi:10.1103/PhysRevLett.86.3827

(9) Sun,B.;Zou,X.W.;Jin,Z.Z.Phys.Rev.E 2004,69,067202. doi:10.1103/PhysRevE.69.067202

(10) Cronemberger,C.M.;Sampaio,L.C.Phys.Rev.E 2006,73, 041403.doi:10.1103/PhysRevE.73.041403

(11)Wu,X.Z.;Pei,M.S.;Wang,L.Y.;Li,X.N.;Tao,X.T.Acta Phys.-Chim.Sin.2010,26,3095.[吴馨洲,裴梅山,王庐岩,李肖男,陶绪堂.物理化学学报,2010,26,3095.]doi:10.3866/ PKU.WHXB20101132

(13) Elezgaray,J.;Léger,C.;Argoul,F.J.Electrochem.Soc.1998, 145,2016.doi:10.1149/1.1838592

(14) Monroe,C.;Newman,J.J.Electrochem.Soc.2003,150, A1377.doi:10.1149/1.1606686

(15) Léger,C.;Elezgaray,J.;Argoul,F.J.Electroanal.Chem.2000, 486,204.doi:10.1016/S0022-0728(00)00143-1

(16)Wang,M.;van Enckevort,W.J.P.;Ming,N.B.;Bennema,P. Nature 1994,367,438.doi:10.1038/367438a0

(17) Nahal,A.;Mostafavi-Amjad,J.;Ghods,A.;Khajehpour,M.R. H.;Reihani,S.N.S.;Kolahchi,M.R.J.Appl.Phys.2006,100, 053503.doi:10.1063/1.2336493

(18)You,H.J.;Fang,J.X.;Chen,F.;Shi,M.;Song,X.P.;Ding,B. J.J.Phys.Chem.C 2008,112,16301.doi:10.1021/jp8042126

(19) Sawada,Y.;Dougherty,A.;Gollub,J.P.Phys.Rev.Lett.1986, 56,1260.doi:10.1103/PhysRevLett.56.1260

(20) Lee,G.J.;Shin,S.I.;Oh,S.G.Chem.Lett.2004,33,118. doi:10.1246/cl.2004.118

(21) Rashid,M.H.;Mandal,T.K.J.Phys.Chem.C 2007,111, 16750.doi:10.1021/jp074963x

(23) Zhou,Y.;Yu,S.H.;Wang,C.Y.;Li,X.G.;Zhu,Y.R.;Chen,Z. Y.Adv.Mater.1999,11,850.doi:10.1002/(SICI)1521-4095 (199907)11:10<850::AID-ADMA850>3.0.CO;2-Z

(24) Kang,Z.;Wang,E.;Lian,S.;Mao,B.;Chen,L.;Xu,L.Mater. Lett.2005,59,2289.doi:10.1016/j.matlet.2005.03.005

(25) Witten,T.A.;Sander,L.M.Phys.Rev.Lett.1981,47,1400. doi:10.1103/PhysRevLett.47.1400

(26) Witten,T.A.;Sander,L.M.Phys.Rev.B 1983,27,5686. doi:10.1103/PhysRevB.27.5686

(27) Nagatani,T.;Sagués,F.Phys.Rev.A 1991,43,2970. doi:10.1103/PhysRevA.43.2970

(28) Sander,L.M.;Cheng,Z.M.;Richter,R.Phys.Rev.B 1983,28, 6394.doi:10.1103/PhysRevB.28.6394

(29) Xiong,H.L.;Yang,Z.M.;Li,H.Acta Phys.-Chim.Sin.2014, 30,413.[熊海灵,杨志敏,李航.物理化学学报,2014,30, 413.]doi:10.3866/PKU.WHXB201401203

(31) Qin,Y.;Song,Y.;Sun,N.;Zhao,N.;Li,M.;Qi,L.Chem. Mater.2008,20,3965.doi:10.1021/cm8002386

(32)Hong,X.;Wang,G.Z.;Wang,Y.;Zhu,W.;Shen,X.S.Chin.J. Chem.Phys.2010,23,596.doi:10.1088/1674-0068/23/05/596-602

(33) Ye,W.;Shen,C.;Tian,J.;Wang,C.;Bao,L.;Gao,H. Electrochem.Commun.2008,10,625.doi:10.1016/j. elecom.2008.01.040

(34) Liao,F.;Wang,Z.F.;Hu,X.Q.Colloid J.2011,73,504. doi:10.1134/s1061933x11040053

(35) Zhang,L.;Ai,Z.;Jia,F.;Liu,L.;Hu,X.;Yu,J.C.Chemistry 2006,12,4185.doi:10.1002/chem.200501404

Monte-Carlo Simulations of the Effect of Surfactant on the Growth of Silver Dendritic Nanostructures

WANG Yuan-YuanXU Qun-XingXIE Hua-Qing*WU Zi-HuaXING Jiao-Jiao
(School of Environmental and Materials Engineering,Shanghai Second Polytechnic University,Shanghai 201209,P.R.China)

The bias diffusion-limited aggregation model is used to study the growth of silver dendritic nanostructures in solution.In the simulation,right-angled isosceles triangle particles are introduced in twodimensional square grids and the sticking possibilities of different particle sides are introduced to describe the effect of the surfactant.Our simulation results show that the dendritic nanostructures become denser with increasing bias voltage.It is also found that the dendritic nanostructures become much more symmetrical and regular when the surfactant is applied.Furthermore,if the effect of the surfactant is strong enough and the bias voltage is small,the branches of the nanostructures are assembled into silver plates.Our simulation results are helpful to explain the experimental results qualitatively.

Monte-Carlo simulation;Silver dendritic nanostructure;Surfactant

1　Introduction

Electrodeposition of metals and alloys,which has been performed for more than a century,is very flexible to manufacture a large number of metallic objects with very different morphologies such as pulverulent deposits,dendrites,needles,rough or porous deposits by changing,sometimes slightly,the experimental conditions.Among different morphologies,dendritic nanostructures have attracted much more attention recently.First,the dendritic growth is sometimes harmful in the electrochemical industry,for example,a serious problem in battery technology1.Secondly,the dendritic nanostructures have potential applications due to its special properties,such as catalysis and superhydrophobicity2. Moreover,dendritic growth in electrodeposition has also been considered as one of the typical out-of-equilibrium phenomena, in which several basic physics can be investigated3.So far,many experimental4－11and theoretical works12－14have been reported.These works mainly focused on different factors which affect the fractal dendritic shape15,such as convection16,ion concentration17,18,voltage18,19,surfactant20－23,and so on.Among these factors, one special factor is the surfactant.The presence of such capping agents can change the free energies for different crystallographic planes and thus their relative growth rates24.However,there still lacks convincing theoretical works to investigate the effect of the surfactant so far.

March 30,2016;Revised:May 26,2016;Published online:May 27,2016.

.Email:hqxie@sspu.edu.cn;Tel:+86-21-50214461.

O647.1

10.3866/PKU.WHXB201605272

The project was supported by the National Natural Science Foundation of China(51406111),Shanghai Natural Science Foundation,China

(14ZR1417000),Scientific Innovation Project of Shanghai Education Committee,China(15ZZ100),and Young Eastern Scholar of Shanghai,China (QD2015052).

国家自然科学基金(51406111),上海市自然科学基金(14ZR1417000),上海教委科研创新项目(15ZZ100)和上海市青年东方学者(QD2015052)资助项目©Editorial office ofActa Physico-Chimica Sinica

(12) Chazalviel,J.N.Phys.Rev.A 1990,42,7355.10.1103/ PhysRevA.42.7355

(22) Sun,X.;Hagner,M.Langmuir 2007,23,9147.10.1021/ la701519x

(30) Meakin,P.Phy.Rev.A 1983,27,1495.10.1103/ PhysRevA.27.1495