一类Caputo分数阶微分方程边值问题多解的存在性*
2016-11-02郭彩霞任玉岗郭建敏
郭彩霞,任玉岗,郭建敏
(山西大同大学数学与计算机科学学院,山西大同 037009)
一类Caputo分数阶微分方程边值问题多解的存在性*
郭彩霞,任玉岗,郭建敏
(山西大同大学数学与计算机科学学院,山西大同 037009)
研究一类Caputo分数阶微分方程边值问题:
分数阶微分方程 边值问题 Leggett-Williams不动点定理
0 引言
分数阶微分方程在工程、化学、物理、生物等领域有着广泛应用,例如热传导领域和流体学领域[1-3],而且分数阶导数模型克服了经典整数阶微分模型理论与实验结果不吻合的缺点[4],因此研究分数阶微分方程边值问题有着重要的意义.近年来,大量文献报道微分方程[5-6]和分数阶微分方程[4,7-10]边值问题解的存在性.2005年,当1<α≤2时,Bai等[7]推导了分数阶微分方程边值问题
目前研究分数阶微分方程边值问题的主要工具有锥拉伸与锥压缩不动点原理、Krasnoselskii不动点原理、Schauder不动点原理上下解等.本文利用Leggett-Williams不动点定理,参照文献[9]中的方法研究Caputo分数阶微分方程边值问题
(0.1)
正解的存在性,其中1<α≤2,f:[0,+)×→[0,+)是连续的,是标准的Caputo微分.一方面,边值问题(1)包含了文献[9]的整数阶微分方程边值问题,推广了文献[9]的结果;另一方面,非线性项f范围有所扩大.
1 预备知识
定义1.1[11]一个连续函数u:(0,+)→的α阶Caputo导数定义为
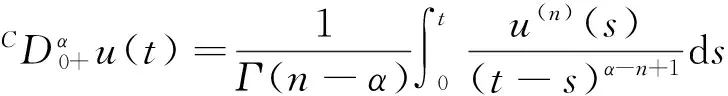
其中α>0,n=[α]+1,[α]代表实数α的整数部分。上式右边在(0,+)内逐点有定义.
引理1.1[11]令α>0,若u∈ACn[0,1]或u∈Cn[0,1],则

引理1.2 令α∈(1,2],给定h∈C[0,1],则
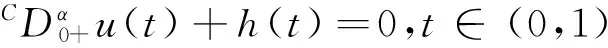
(1.1)
u′(0)=u(1)=0,
(1.2)
因此,(1.1)~(1.2)式的唯一解是

引理1.3 引理1.2中的G(t,s)有下列性质:
(i)G(t,s)∈C([0,1]×[0,1],)且G(t,s)>0,t,s∈(0,1);

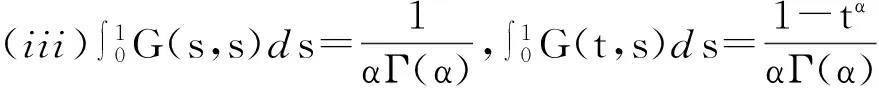
0≤t≤1,
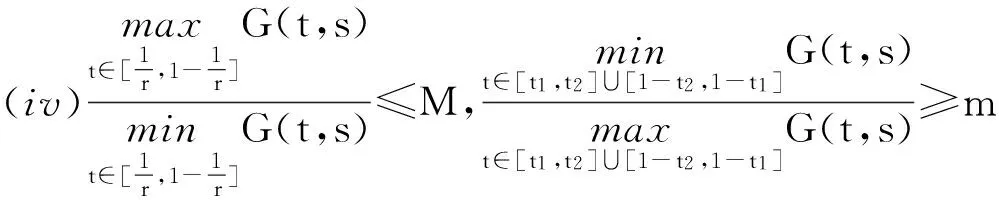
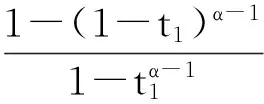
证明 (i)~(iii)显然可得,只需证明(iv).

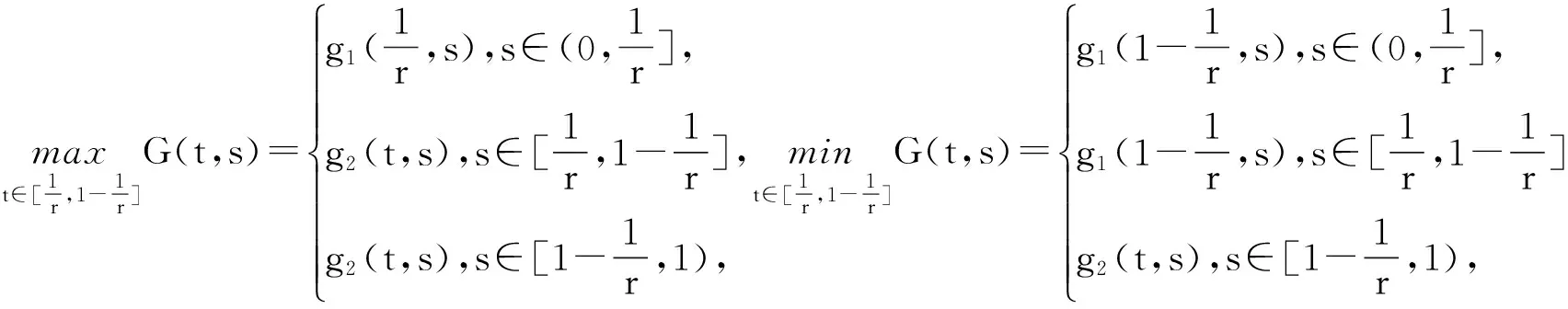

又
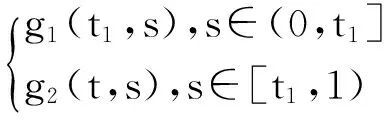

令γ,β,θ是锥P上的非负连续凸函数,α,ψ是锥P上的非负连续凹函数,那么对非负实数h,a,b,d和c,定义下列凸集:
P(γ,c)={u∈P:γ(u) Q(γ,β,d,c)={u∈P:β(u)≤d,γ(u)≤c}, P(γ,θ,α,a,b,c)={u∈P:a≤α(u),θ(u)≤b,γ(u)≤c}, Q(γ,β,ψ,h,d,c)={u∈P:h≤ψ(u),β(u)≤d,γ(u)≤c}. 定理1.1[12]令E是一个实Banach空间,且P⊂E是一个锥.假设存在正数c和M,使锥P上的非负连续凹函数α,ψ及非负连续凸函数γ,β,θ满足 (B1){u∈P(γ,θ,α,a,b,c):α(u)>a}≠∅且α(F(u))>a,u∈P(γ,θ,α,a,b,c); (B2){u∈Q(γ,β,ψ,h,d,c):β(u) (B3)若u∈P(γ,α,a,c)且θ(F(u))>b,则α(F(u))>a; (B4)若u∈Q(γ,β,d,c)且ψ(F(u)) β(u1) α(u)≤β(u), (2.1) (2.2) (H3)f(t,u(t))≤c αΓ(α),t∈[0,t3]∪[1-t3,1],u(t)∈[0,c]. 那么,边值问题(1)至少有3个正解u1,u2和u3,满足 证明 在锥P上定义算子A为 因为 所以A:P→P连续.由Arzela-Ascoli定理易证A:P→P是全连续的. ds=b; α(u1)>b,β(u2) 本文研究了一类Caputo分数阶微分方程边值问题多解的存在性.证明时,将微分方程边值问题转化为积分方程,进一步转化为讨论积分算子不动点的问题,然后通过运用Leggett-Williams不动点定理该分数阶微分方程边值问题至少有3个正解存在的结果,其中格林函数的性质和非线性项的条件至关重要. [1] PODLUBNY I.Fractional Differential Equations,Mathematics in Science and Engineering[M].New York:Academic Press,1999. [2] ADOMIAN G, ELROD M,RACH R.A new approach to boundary value equations and application to a generalization of Airy’s equation[J].J Math Anal Appl,1989,140(2):554-568. [3] AGARWAL R P,MEEHAN M,O’REGAN D.Fixed Point Theory and Applications[M].Cambridge:Cambridge University Press,2001. [4] ABDELJAWAD T, BALEANU D.Fractional differen- ces and integration by parts[J].Journal of Computational Analysis and Applications,2011,13(3): 574-582. [5] 王勇,韦煜明.二阶非线性时滞微分方程边值问题正解的存在性[J].广西科学,2012,19(1):40-43. WANG Y,WEI Y M.Existence of positive solutions for boundary value problems of nonlinear second-order delay differential equations[J].Guangxi Sciences,2012,19(1):40-43. [6] 严建明.中立型微分方程的正解存在性及非振动解的渐近性[J].广西科学,2008,15(1):7-9. YAN J M.Existence of asymptotic behavious of positive solution of neutral differential equation[J].Guangxi Sciences,2008,15(1):7-9. [7] BAI Z B,LU H S.Positive solutions for boundary value problem of nonlinear fractional differential equation[J].Journal of Mathematical Analysis and Applications, 2005,311(2): 495-505. [8] BAI Z B,QIU T T.Existence of positive solution for singular fractional differential equation[J].Applied Mathematics and Computation,2009,215(7):2761-2767. [9] DOGAN A.On the existence of positive solutions for the second-order boundary value problem[J].Applied Mathematics Letters,2015,49:107-112. [10] XIE W Z,XIAO J,LUO Z G.Existence of extremal solutions for nonlinear fractional differential equation with nonlinear boundary conditions[J].Applied Mathematics Letters,2015,41:46-51. [11] OLDHAM K B,SPANIER J.The Fractional Calculus [M].New York:Academic Press,1974. [12] AVERY R I.A generalization of the Leggett-Williams fixed point theorem[J].Math Sci Res Hot-Line, 1999,3(7):9-14. (责任编辑:尹 闯) Existence of Multiple Solutions for a Caputo Fractional Difference Equation Boundary Value Problem GUO Caixia,REN Yugang,GUO Jianmin (School of Mathematics and Computer Science,Datong University,Datong,Shanxi,037009,China) We investigate the existence and multiplicity of positive solutions for nonlinear Caputo fractional differential equation boundary value problem fractional difference equation,boundary value problem,Leggett-Williams fixed point theorems 2016-05-15 郭彩霞(1980-),女,讲师,主要从事基础数学方面的研究,E-mail:iris-gcx@163.com(C.Guo)。 *国家自然科学基金项目(No.11271235),大同大学青年科研基金项目(2014Q10)和河南省高等学校重点科研计划项目(15A110047)资助。 网络优先数字出版时间:2016-09-13 【DOI】10.13656/j.cnki.gxkx.20160913.002 http://www.cnki.net/kcms/detail/45.1206.G3.20160913.0948.004.html 多解的存在性,其中1<α≤2,f:[0,+∞)×→[0,+∞)是连续的,是标准的Caputo微分.先将微分方程边值问题转化为积分方程,再转化为积分算子不动点问题,最后利用Leggett-Williams不动点定理得出Caputo分数阶微分方程边值问题至少有3个正解存在,其中格林函数的性质和非线性项的条件至关重要. O175.8 A 1005-9164(2016)04-0374-04 Where 1<α≤2,f:[0,+∞)×→[0,+∞) is continuous,andis the standard Caputo differentiation.In the process of proof,we first transform it into integral equation,then differential equation boundary value problem is further converted to discuss the problem of integral operator fixed point. Finally,by means of Leggett-Williams fixed point theorems on cone,existence results of at least three positive solutions are obtained.The properties of the Green function and the conditions of the nonlinear term is very important. 广西科学Guangxi Sciences 2016,23(4):374~3772 主要结果
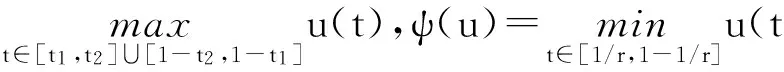



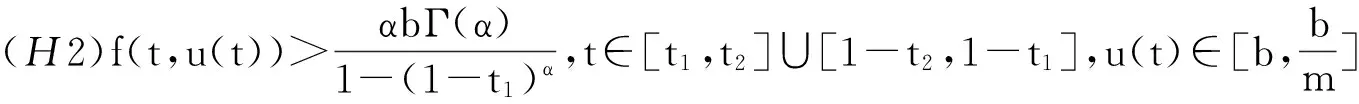
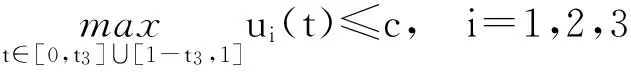
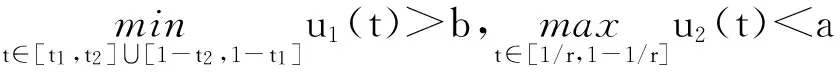

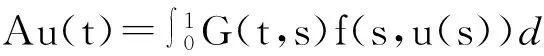


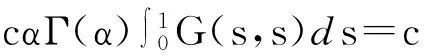
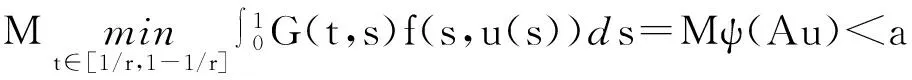
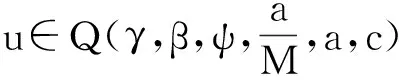

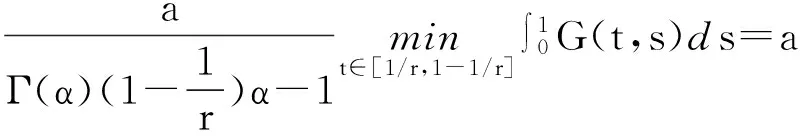
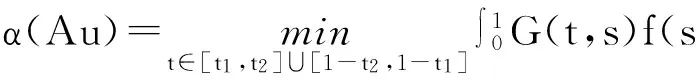


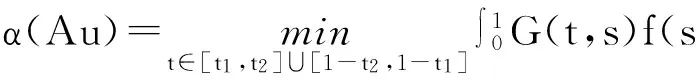

3 结论
