基于双线性对的有序多重签名

杨青　李文胜　吕敏红

摘 要： 本文对已有多重签名方案进行分析，提出快速和高效的基于双线性对的有序多重签名方案.并给出具体签名算法和验证算法，比较和分析改进方案的复杂度和安全性，改进方案的运算时间减少了32.016n+123.142毫秒.改进方案所需时间少，运算量低，安全性高且易于实现.

关键词： 超椭圆曲线 约化除子 双线性对 多重签名

引言

多重数字签名是指多个人合作对同一份消息进行签名.1994年，Harn L提出了基于Meta-ElGamal方案的多重签名方案[1].由于签名过程不同，可分为有序多重签名和广播多重签名.签名者按照串行的顺序进行签名称为有序多重签名，而广播多重签名对签名顺序没有要求.Harn L在2005年又提出了基于RSA的有序和广播多重签名方案[2].人们将椭圆曲线双线性对用于多重签名方案，例如，文献[4-6].

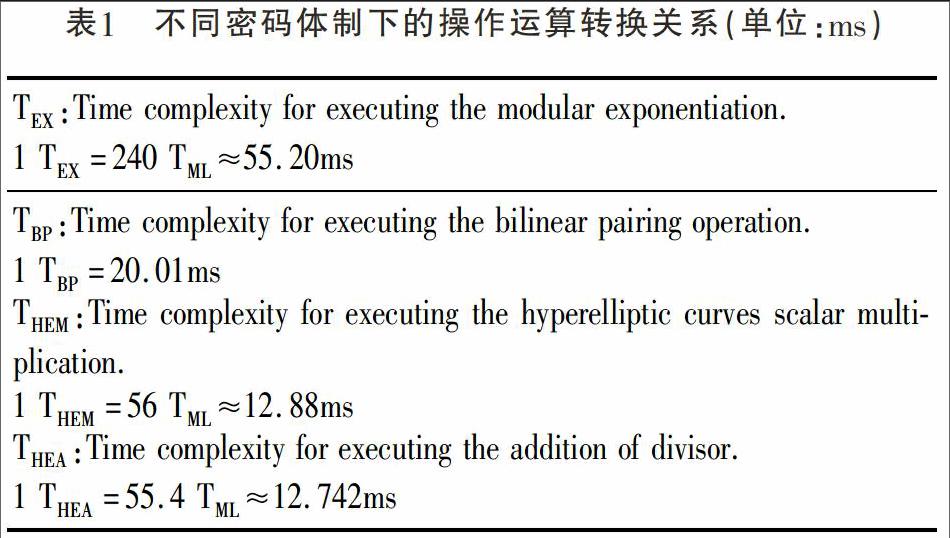
本文对文献的多重签名方案进行了改进，提出了更快速和高效的基于超椭圆曲线双线性对的多重签名方案.首先提出改进的有序多重签名方案，并给签名算法和验证算法.其次，证明算法的正确性和安全性.最后，比较和分析改进方案的安全性和复杂度，并应用于超椭圆曲线密码系统[3].该算法具有快速、高效且易于实现的特点.

（2）防止签名者内部人员伪造签名.若签名集合内部的某个签名者想伪造签名，他首先得通过后继签名者的验证，要求解前一个签名者的私钥.每个签者的公钥公开，对应的私钥是秘密的，想要求解私钥相当于求解超椭圆曲线的Jacobian群上的离散对数问题，这是不可行的，从而能够抵抗伪造攻击.

结语

本文改进了文献提出的多重签名方案，更符合实际应用中的多重签名.还分析和比较了改进方案和文献的计算效率，改进方案运算时间减少32.016n+123.142 （ms）.改进方案具有运算量低，所需时间少，且易于实现等优点.同时，改进方案具有高安全性能.

参考文献：

[1]Harn L.New digital signature scheme based on discrete logarithm[J].Electronics Letters.1994，30（5）：396-398.

[2]Harn L，Lin CY，Wu C T.Structured multisignature algorithms [J].IEE computers and digital techniques，2004，151（3）：231-234.

[3]Siman YANG，Hongfeng WU，Jiyou LI.Access structures of hyperelliptic secret sharing schemes[J].Finite Fields and Their Applications，2016，vol.37，46-53.

[4]Biao Wang，Xiao-dong Yang，Guang Yang.An Identity-Based multisignature scheme from the weil pairing[J].In：Proceedings of the 2010 international conference on computer design and applications（ICCDA 2010），2010，vol.5：585-587.

[5]Islam S.H.，Biswas G.P.Certificateless strong designated verifier multisignature scheme using bilinear pairings[J].In：Proceedings of the international conference on advances in computing Communications and informatics （ICACCI-2012），2012b：540-546.

[6]Islam S.H.，Biswas G.P.Certificateless short sequential and broadcast multisignature schemes using elliptic curve bilinear pairings[J].Journal of King Saud University--Computer and Information Sciences，2014，26：89-97.

[7]Fuw-Yi Yang，Jeng-Hung Lo，Cai-Ming Liao.Improvement of an efficient ID-based RSA multisignature[J].In：Proceedings of the International Conference on Complex，Intelligent and Software Intensive Systems，2010：822-826.

[8]Lange T.Formulae for arithmetic on genus 2 hyperelliptic curves[J].Applicable algebra in engineering，communication and computing，2005，15（5）：295-328.

[9]M Li，FY Kong，DM Zhu.Fast addition formulae for Montgomery Ladder scalar multiplication on hyperelliptic curves[J].Journal of Software，2013，24（10）：2275-2288.