一个（3＋1）维非线性发展方程的Bäcklund变换和解

郭婷婷

（山西大学商务学院,山西太原030031）

一个（3＋1）维非线性发展方程的Bäcklund变换和解

郭婷婷

（山西大学商务学院,山西太原030031）

通过运用多维二元Bell多项式,文中给出（3＋1）维非线性发展方程的双线性Bäcklund变换,这样可以避免Hirota双线性方法中恒等式的选取.除此之外,文中还构造出该非线性方程的N－波解.

双Bell多项式；（3＋1）维非线性发展方程；双线性表示；Bäcklund变换

1 概述

孤立子理论中,对非线性发展方程求解是比较重要的研究问题,经过长期的努力,现有的构造非线性方程精确解的方法有,Darboux变换法、对称约化法、反散射法、双线性方法［1］等.在研究过程中,为避免Hirota双线性方法中变换的构造,我们基于多维二元Bell多项式［2］,将非线性方程双线性化,在以往研究低维非线性方程的基础上,我们涉足高维的非线性演化方程双线性表示的探讨.

Bäcklund变换［3］是1883年瑞典数学家Bäcklund研究曲面时发现的,利用该变换,我们可以由非线性方程的已知解推导出新的孤子解.为了避免应用Hirota双线性方法推导Bäcklund变换时恒等式的推导和选用,基于多维二元Bell多项式,我们推导出（3＋1）维非线性发展方程的双线性Bäcklund变换,这将为非线性方程求解提供便利的条件.

2 多维二元Bell多项式

定义1多维Bell多项式［4］定义：h＝h（z1,…,zk）是一个C∞的多变量函数,bi≥0,i＝1,2,…,k是任意整数eh称为多维Bell多项式.当h＝h（x,y,t）时,

定义2多维二元Bell多项式：

其中pl＝0,1,…,al,l＝0,1,…,k.

我们给出文中所用到的多维二元Bell多项式,

定理1多维二元Bell多项式与经典的Hirota算子的关系［5］如下

当F＝G时,

这里给出文中所用的几个恒等式,

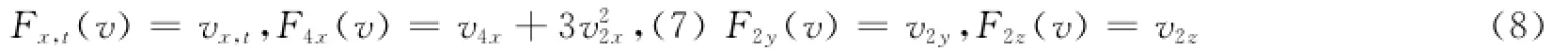
定理2（线性叠加原理［6］）Q（y1,…,yk）是满足Q（0,…,0）＝0的偶多项式,ξi＝l1,iy1＋…＋lk,iyk为N波变量,li,j为常数,则指数波eξi的线性组合为双线性方程的N－波解.求解双线性方程Q（y1,…,yk）G·G＝0当且仅当

3 （3＋1）维非线性发展方程的双线性表示

研究（3＋1）维非线性发展方程［7］

的双线性表示,首先引入势场变量v,使得

这样非线性方程（9）将变形为（cv2z,t＋6c2v2xv3x＋cv5x）x＋3cv2x,2y＋3cv2x,2z＝0,

为得到双线性表示需对x积分两次并取积分常数为0,且取c＝1,则

根据（7）（8）式方程将变形为F－多项式

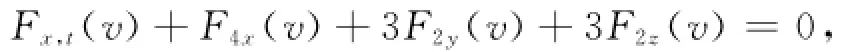
通过利用定理1和以下的变换,

我们可以得出（3＋1）维非线性发展方程的双线性表示

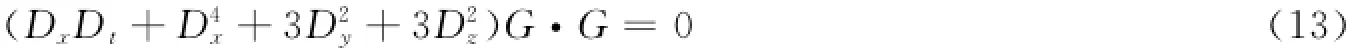
4 （3＋1）维非线性发展方程的N－波解

我们考虑该非线性发展方程的N－波解,首先引入N波变量

ξi＝aix＋biy＋ciz＋dit,1≤i≤N和N波指数函数Fi＝eξi＝eaix＋biy＋ciz＋dit,1≤i≤N,依据定理2的充分必要条件,求N－波解需满足以下关系式

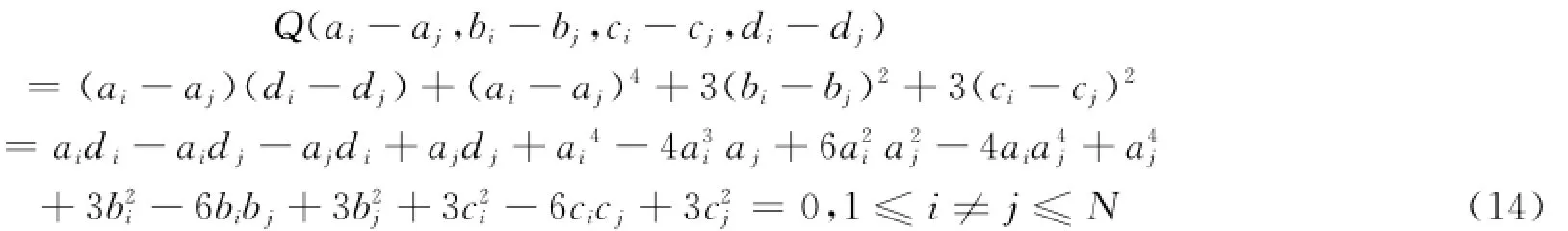
求解方程（14）可以得出ai,bi,ci,di之间的关系如下

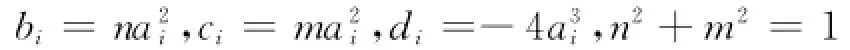
所以（3＋1）维非线性发展方程（9）存在N－波解

其中n2＋m2＝1,ai,ki为任意常数.

5 （3＋1）维非线性发展方程的双线性Bäcklund变换

为确定（3＋1）维KdV方程双线性的Bäcklund变换,我们首先假设p＝2lnG1和p′＝2lnG2是方程（11）的两个解,代入（11）式并做差得

取p－p′＝2u,p＋p′＝2v,方程（16）将化为

运用定义2中公式（3）（4）,上式将转化为

这里k（u,v）＝6u2xv2x－6uxv3x－6u2xu2x＝6Wronskian［Q2x（u,v）,Qx（u）］

现取Q2x（u,v）＝λQx（u）,则（18）式可化为Q多项式型的耦合系统

其中λ为任意常数,结合定理1,我们获得（3＋1）维非线性发展方程（9）的双线性Bäcklund变换

综上,我们对（3＋1）维非线性发展方程进行研究,给出该非线性方程的双线性表示（13）和N－波解（15）,并给出其双线性Bäcklund变换（19）,这将为非线性方程求解奠定基础.

［1］Zhang Yu－feng,Tam Honwah and Zhao Jing.Higher－dimensional KdV equations and their soliton solutions［J］.Commun.Theor.Phys,2006,45（3）：411－413

［2］Hirota R.The direct method in soliton theory［M］.New York：Cambridge University Press,2004：30－35

［3］Guo Fukui,Feng Binlu,Guo Tingting.A Few Notes on Lax Integrability,Integrable Couplings and Computing Formula of the Constant r［J］.Journal of Applied Nonlinesr Dynamics,2012,1（4）：401－406

［4］Lambert,F.,Springael,J.Soliton equations and simple combinatorics［J］.Acta Applicandae Mathematicae,2008,102（2－3）：147－178

［5］Fan,Engui,The integrability of nonisospectral and variable－coefficient KdV equation with binary Bell polynomials［J］.Physics Letters A,2011,375（3）：493－497

［6］Ma,Wenxiu,Fan,Engui.Linear superposition principle applying to Hirota bilinear equations［J］.Computer and Mathematics with Applications,2011,61（4）：950－959

［7］郭冠平.（3＋1）维非线性方程新的精确解［J］.四川师范大学学报,2002,25（2）：159－163

The Bäcklund Transformations and Solutions of a（3＋1）Dimensional Nonlinear Evolution Equation

GUO Tingting
（Business College of Shanxi University,Taiyuan 030031,China）

The approach can avoid selections of the transformations in the Hirota bilinear method.the bilinear Bäcklund transformations of the（3＋1）dimensional nonlinear evolution equation are obtained using the multi dimensional binary Bell polynomials.The way can avoid the deduction of the identities in the Hirota bilinear method.In addition,the N－wave solutions of this nonlinear evolution equation are obtained.

binary Bell polynomials；（3＋1）dimensional nonlinear evolution equation；bilinear representation；Bäcklund transformations.

1672－2027（2016）04－0001－03

O129.35

A

2016－06－21

山西大学商务学院科研基金（2016028）.

郭婷婷（1983－）,女,山西太原人,硕士,主要从事应用数学孤立子理论研究.