形状记忆合金-摩擦串联复合阻尼器对偏心结构振动控制的研究
2016-01-12任文杰,姚会哲,马志成等
第一作者任文杰女,博士,教授,1972年12月生
形状记忆合金-摩擦串联复合阻尼器对偏心结构振动控制的研究
任文杰1,2,姚会哲1,2,马志成1,2,宋娃丽1,2
(1.河北工业大学土木工程学院,天津300401; 2. 河北省土木工程技术研究中心,天津300401)
摘要:采用自行研制的SMA-摩擦串联复合阻尼器控制偏心结构的平扭耦联振动。SMA-摩擦串联复合阻尼器能根据结构的地震响应自动调节耗能单元工作状态,且构造简单、经济实用。建立了SMA-摩擦串联复合阻尼器控制下偏心结构在双向水平地震作用下的运动方程,并编写程序计算结构的时程响应。以一六层剪切型偏心钢框架为例,计算结果表明:合理布置的阻尼器能有效抑制结构质心位移和质心层间位移,且对扭转振动的控制效果更佳;阻尼器对结构质心平移加速度的影响不大,但显著改变了扭转加速度。
关键词:形状记忆合金-摩擦串联复合阻尼器;偏心结构;振动控制
基金项目:国家自然科学基金面上项目(50978081);中国博士后科学基金面上项目(2013M541165);住宅和城乡建设部科学技术项目(2014-K4-037)
收稿日期:2013-08-08
中图分类号:TU352.1;TG139+.6文献标志码:A
Vibration control of an eccentric structure using SMA-friction tandem dampers
RENWen-jie1,2,YAOHui-zhe1,2,MAZhi-cheng1,2,SONGWa-li1,2(1. School of Civil Engineering, Hebei University of Technology, Tianjin 300401, China; 2. Civil Engineering Technology Research Center of Hebei Province, Tianjin 300401, China)
Abstract:Self-made shape memory alloy (SMA)-friction tandem dampers were used to control the translational-torsional coupled vibration of an eccentric structure. The SMA-friction tandem damper could adjust the working status of energy consumption elements automatically according to seismic responses of the structure, and had advantages of simple structure and small cost. The motion equations of the eccentric structure with the SMA-friction tandem dampers subjected to the bidirectional horizontal seismic actions were established, and the time history responses of the structure were computed with a procedure programmed. Taking a six-story shear-type eccentric steel frame as an example, the numerical results showed that the dampers deployed on the structure reasonably can attenuate the displacements and the inter-story displacements of the mass center of the structure, their control effects on torsional vibrations are better; their control effects on the translational accelerations of the mass center of the structure are slight, but they change the torsional aeeclerations obviously.
Key words:shape memory alloy (SMA)-friction tandem damper; eccentric structure; vibration control
复杂建筑结构由于质心与刚心的不重合会在地震作用下发生平扭耦联振动,从而加重结构的破坏[1-3]。传统的预防措施是调整结构平面布置或增加结构的抗扭刚度,但前者在建筑功能已确定的情况下调整的余地很小,后者不经济,强震时也达不到预期效果[4]。耗能减震技术通过在结构某些部位设置阻尼器来耗散地震能量从而减轻结构的振动,是解决这一难题的有效途径。
超弹性形状记忆合金(Shape Memory Alloy,SMA)具有大应变恢复能力,且强度高,抗腐蚀、抗疲劳性好,是理想的耗能材料。近几十年,国内外学者开发了各种超弹性SMA基阻尼器,如:Li等[5]研制了拉伸型阻尼器和剪刀型阻尼器,Parulekar等[6]制造了双筒式阻尼器,Asgarian等[7]设计了不同构造的SMA拉索,钱辉等[8]提出了SMA-摩擦并联复合阻尼器,等等。这些阻尼器在应用中存在着共同的弊端,即:阻尼器中的耗能单元(SMA或SMA与其它耗能材料)同时工作,不能根据结构的地震响应自动调节耗能状态,且SMA用量较多,小震和中震时其功能未充分发挥,这显然是不经济的。
为了克服前述阻尼器的不足,任文杰等[9]设计了一种SMA-摩擦串联复合阻尼器,并对阻尼器的力学性能进行了试验和理论研究。本文在此基础上拟将该阻尼器用于偏心结构中,以控制结构在地震作用下的平扭耦联振动。
1SMA-摩擦串联复合阻尼器及其力学模型[9]
SMA-摩擦串联复合阻尼器是将SMA丝的超弹性与摩擦耗能机理串联起来,能够根据结构的地震响应自动调节耗能单元的工作状态:荷载较小时,仅SMA丝发挥耗能和复位功能;荷载较大时,SMA丝和摩擦单元共同工作,消耗大量能量的同时具有一定的变形回复能力。该阻尼器用丝量少,构造简单,更加经济实用。制作的阻尼器模型如图1所示。
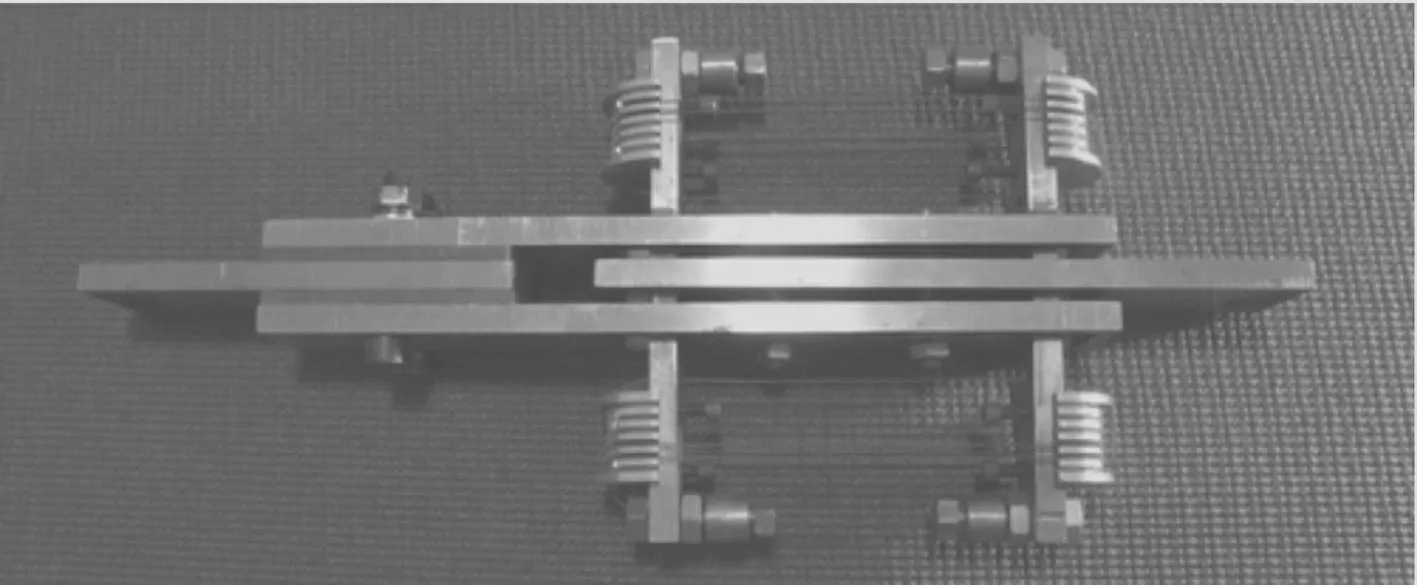
图1 SMA-摩擦串联复合阻尼器模型 Fig.1 Model of the SMA-friction tandem damper
SMA-摩擦串联复合阻尼器的力学模型如下:
(1)
式中:fd是阻尼器的输出力;x是阻尼器的位移;xmax和xmin是临界位移,当阻尼器残余位移为0时,xmax=-xmin=xe,xe是SMA丝的最大变形量,当残余位移不为0时,xmax、xmin分别为拉、压加载后开始卸载时对应的位移值,满足xmax-xmin=2xe;fFRI是滑动摩擦力;fSMA是SMA丝的输出力,其值根据Graesser本构模型计算,即:
(2)
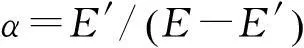
(3)

(4)
2SMA-摩擦串联复合阻尼器控制偏心结构振动的运动方程
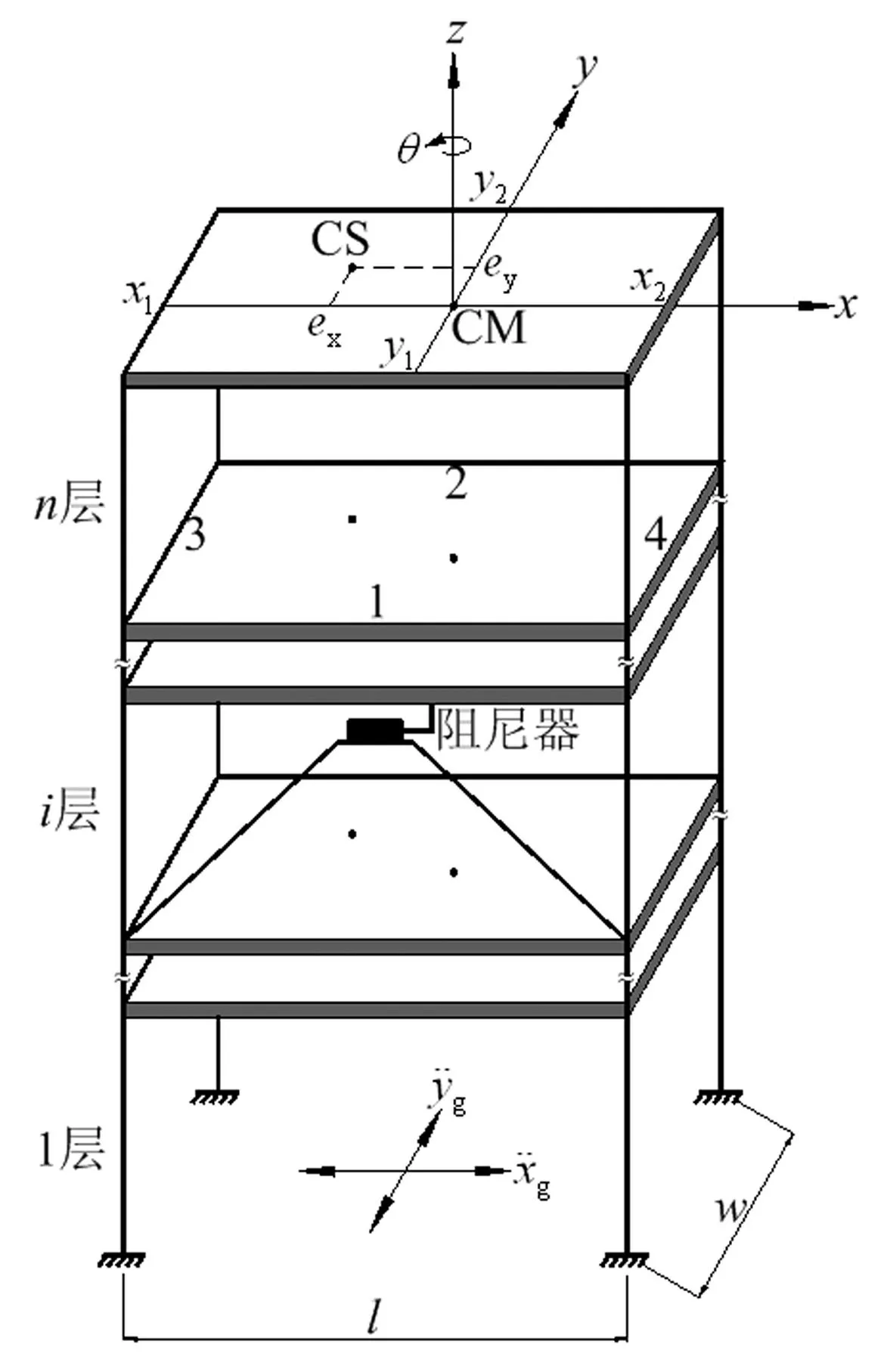
图2 偏心结构模型 Fig.2 Model of the eccentric structure
偏心结构的运动方程可写为:

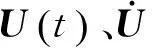
U(t)=[ux1…uxnuy1…uynuθ1…uθn]T
(6)
(7)
(8)
Fd(t)=
[fd11…fd1nfd21…fd2nfd31…fd3nfd41…fd4n]T
(9)
M=diag[m1,…,mn,m1,…,mn,J1,…,Jn]
(10)
(11)
式中:
(12)
(13)
Kyy和Kθθ与Kxx相似,仅需将后者元素的下标x换成y和θ即可。

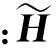

(15)
需说明的是:若i层j位置处无阻尼器,则Fd(t)的元素fdji所在行删除,H的对应列亦删除。

(16)
在Simulink环境中编写程序,求解运动方程(5),以获得结构地震时程响应。
3数值分析
3.1 模型概况
一六层剪切型钢框架结构:首层层高4.8 m,其它层层高4.2 m,l=15 m,w=24 m,x1=-7.5 m,x2=7.5 m,y1=-12.5 m,y2=11.5 m,ex=-0.2 m,ey=0.5 m。各层结构参数相同:mi=3.456×105kg,Ji=2.37×107kg·m2,kxi=kyi=3.404×108N/m,kθi=3.840×1010N·m/rad,i=1,…,6。一阶和二阶振型阻尼比均为0.04。计算得到的结构前三阶自振周期依次为0.833s、0.831 s和0.645 s。
SMA-摩擦串联复合阻尼器力学参数:SMA丝长度200 mm,A=5.03 mm2,E=30.9 kN/mm2,Y=0.435 kN/mm2,α=0.13,λ=5,fT=0.23,a=197,c=0.09,fFRI=6.9 kN,xe=12 mm。阻尼器在结构中的布置如图2所示,假设有控结构的最大层间位移角不超过1/250,则不同位置处阻尼器的数量确定如下:第一、二和三层的位置1和位置4处均为40个,位置2和位置3处均为30个;第四、五和六层的位置1和位置4处均为30个,位置2和位置3处均为20个。
地震动记录选用El-Centro波(1940.5.18),截取0 s~10 s的强震部分:沿x向的加速度峰值被调整为2.2 m/s2,沿y向的加速度峰值被调整为1.87 m/s2。
3.2 计算结果与分析
计算得到有控结构的最大层间位移发生在首层位置1处,为19.23 mm,满足层间位移角要求。
表1列出了结构质心位移峰值及其减震率,表2列出了结构质心层间位移峰值及其减震率,表3列出了结构质心加速度峰值及其减震率,图3绘出了结构顶层质心位移时程曲线。可见:
(1)阻尼器能有效抑制结构质心位移,且对扭转响应的控制效果更为显著,各方向减震率分别为:x向17.26%~21.59%;y向16.62%~20.44%;θ向49.52%~53.16%。
(2)结构质心层间位移得到有效控制:x向减震率为5.21%~21.59%;y向减震率为6.33%~20.55%;θ向减震率更高,为30%~50%。
(3)阻尼器对不同楼层质心加速度的影响,或是放大或是减小:平移加速度的变化不大,x向和y向减震率分别不超过6.80%和4.90%;扭转加速度变化显著,其值在第一层~第四层大大增加,涨幅为225.00%~23.60%,在第五层和第六层减小,幅度分别为21.21%和13.51%。

表1 结构质心位移峰值及其减震率

表2 结构质心层间位移峰值及其减震率

图3 结构顶层质心位移时程曲线 Fig.3 Displacement time history of the top floor of the mass center of the structure
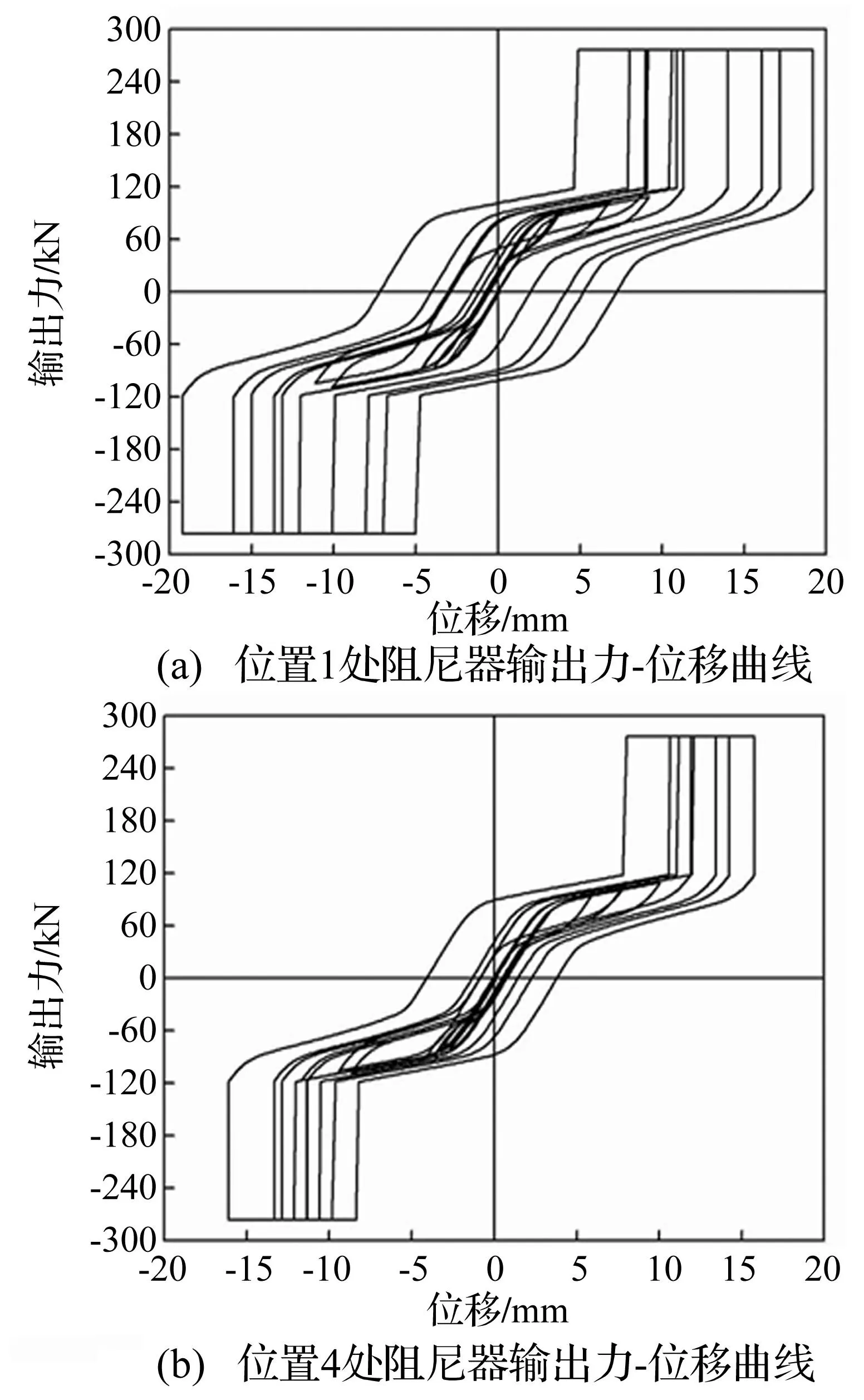
图4 结构首层SMA-摩擦复合阻尼器的输出力-位移曲线 Fig.4 Force-displacement curve of the SMA-friction tandem damper on the first floor of the structure

楼层x向/(m·s-2)y向/(m·s-2)θ向/(rad·s-2)无控有控减震率/%无控有控减震率/%无控有控减震率/%13.2023.264-1.942.7322.734-0.070.0400.130-225.0024.1834.207-0.573.5733.607-0.950.0700.164-134.2934.4044.2174.253.7613.7061.460.0830.148-78.3144.4054.2613.273.713.789-2.130.0890.110-23.6055.0424.6996.804.2554.0624.540.0990.07821.2165.4025.0716.134.5894.3644.900.1110.09613.51
注:表1~表3中的减震率=(无控值-有控值)/无控值×100%
为了更好地反映阻尼器的工作性能以及结构的减震机理,计算了阻尼器的输出力-位移曲线。篇幅所限,图4仅绘出结构首层位置1和位置4处阻尼器的合输出力-位移曲线,可见:根据结构的地震响应,阻尼器自动调节着SMA丝伸缩和摩擦滑移运动,消耗能量的同时也驱使结构向初始位置靠近。
4结论
本文采用自行研制的SMA-摩擦串联复合阻尼器控制偏心结构的平扭耦联振动。SMA-摩擦串联复合阻尼器能根据结构的地震响应自动调节耗能单元工作状态,且构造简单、经济实用。建立了SMA-摩擦串联复合阻尼器控制的偏心结构在双向水平地震作用下的运动方程,并编写程序计算结构的时程响应。以一六层剪切型偏心钢框架为例,计算结果表明:合理布置的阻尼器能有效抑制结构质心位移和质心层间位移,且对扭转振动的控制效果优于平移振动;阻尼器对结构质心平移加速度的影响不大,但显著改变了质心扭转加速度。
参考文献
[1]任晓崧, 翁大根, 吕西林. 四川灾区砌体结构房屋震害与中小学建筑的抗震设计[J]. 工程抗震与加固改造, 2008, 40(8): 30(4):71-76.
REN Xiao-song, WENG Da-gen, LÜ Xi-lin. Earthquake damage of masonry buildings in Sichuan province and discussion on seismic design for primary and middle school buildings [J]. Earthquake Resistant Engineering and Retrofitting, 2008, 30(4): 71-76.
[2]Kim Y, Kabeyasawa T, Igarashi S. Dynamic collapse test on eccentric reinforced concrete structures with and without seismic retrofit [J]. Engineering Structures,2012,34:95-110.
[3]Kilar V, Petrovǒiǒ S, Koren D,ilih S, et al. Seismic analysis of an asymmetric fixed base and base-isolated high-rack steel structure [J]. Engineering Structures, 2011, 33: 3471-3482.
[4]程光煜, 叶列平, 朱兴刚. 偏心结构消能减震技术的分析研究[J]. 工程抗震与加固改造, 2006, 28(2): 78-83.
CHENG Guang-yu, YE Lie-ping, ZHU Xing-gang. Analysis on energy dissipation control for eccentric structure [J]. Earthquake Resistant Engineering and Retrofitting, 2006, 28(2): 78-83.
[5]Li H, Mao C X, Ou J P. Experimental and theoretical study on two types of shape memory alloy devices [J]. Earthquake Engineering and Structural Dynamics, 2008, 37(3): 407-426.
[6]Parulekar Y M, Reddy G R, Vaze K K, et al. Seismic response attenuation of structures using shape memory alloy dampers [J]. Structural Control and Health Monitoring, 2010, 19(1): 102-119.
[7]Asgarian B, Moradi S. Seismic response of steel braced frames with shape memory alloy braces [J]. Journal of Constructional Steel Research, 2011, 67(1): 65-74.
[8]钱辉, 李宏男, 任文杰, 等. 形状记忆合金复合摩擦阻尼器设计及试验研究[J]. 建筑结构学报, 2011, 32(9): 58-64.
QIAN Hui, LI Hong-nan, REN Wen-jie, et al. Experimental investigation of an innovative hybrid shape memory alloys friction damper [J]. Journal of Building Structures, 2011, 32(9): 58-64.
[9]任文杰, 王利强, 马志成, 等. 形状记忆合金-摩擦复合阻尼器力学性能研究[J]. 建筑结构学报, 2013, 34(2): 83-90.
REN Wen-jie, WANG Li-qiang, MA Zhi-cheng, et al. Investigation on mechanical behavior of innovative shape memory alloy-friction damper [J]. Journal of Building Structures, 2013, 34(2): 83-90.

