An effective method to predict oil recovery in high water cut stage*

LIU Zhi-bin （刘志斌）， LIU Hao-han （刘浩翰），2. School of Science， Southwest Petroleum University， Chengdu 60500， China，E-mail： liuzhibinswpi@vip.sina.com2. Department of Information Engineering， Sichuan College of Architectural Technology， Deyang 68000， China

An effective method to predict oil recovery in high water cut stage*

LIU Zhi-bin （刘志斌）1， LIU Hao-han （刘浩翰）1，2
1. School of Science， Southwest Petroleum University， Chengdu 610500， China，E-mail： liuzhibinswpi@vip.sina.com
2. Department of Information Engineering， Sichuan College of Architectural Technology， Deyang 618000， China

（Received April 13， 2013， Revised August 10， 2013）

The water flooding characteristic curve method based on the traditional regression equation between the oil and water phase permeability ratio and the water saturation is inappropriate to predict the oil recovery in the high water cut stage. Hence， a new water flooding characteristic curve equation adapted to the high water cut stage is proposed to predict the oil recovery.The water drive phase permeability experiment s show that the curve of the oil and water phase permeability ratio vs. the water saturation， in the semi-logarithmic coordinates， has a significantly lower bend after entering the high water cut stage， so the water flooding characteristic curve method based on the traditional regression equation between the oil and water phase permeability ratio and the water saturation is inappropriate to predict the oil recovery in the high water cut stage； therefore， a new water flooding characteristic curve equation based on a better relationship between ln（kro/krw）and Swis urgently desirable to be established to effectively and reliably predict the oil recovery of a water drive reservoir adapted to a high water cut stage. In this paper， by carrying out the water drive phase permeability experiment s， a new mathematical model between the oil and water phase permeability ratio and the water saturation is established，with the regressionanalysis method and an integration of the established model， the water flooding characteristic curve equation adapted to a high water cut stage is obtained. Using the new water flooding characteristic curve to predict the oil recovery of the GD3-block of the SL oilfield and the J09-block of the DG oilfield in China， results with high predicted accuracy are obtained.

high water cut stage， phase permeability equation， water flooding characteristic curve， predicting， oil recovery

Introduction0F

The enhanced oil recovery （EOR） is a difficult problem facing us today. Its study is important for the effective development of oilfields. Juttner and Rajkovic［1］discussed the implementation of the enhanced oil recovery from the aspects of economy and environment. Al-Sulaimani et al.［2］tested the potential of the microbially enhanced oil recovery as an effective alternative in Omani oil fields. Hou et al.［3］established a pseudo-three-dimensional model to predict the potentiality of the oil recovery. To further study the application and the potential of the oil recovery. Al Shalabi et al.［4］studied the application of the direct current potential to enhancing the recovery efficiency. Many influencing factors of the oil recovery are involved. In Refs.［5-7］， the influence of the displacement pressure on the oil recovery was studied， the effects of the injected-water salinity on the water flood sweep efficiency were studied by Lemon et al.［8］， the influences of the heterogeneity， the wetting， and the viscosity ratio on the oil recovery were comprehensively studied by Salimi and Bruining［9］based on previous studies. Correspondingly， many oil recovery prediction methods were proposed， Zhang et al.［10］established a new well test model from the point of view of the well test analysis to improve the oil recovery， by experiments with respect to Alkali/Surfactant/Polymer flooding in porous media， Shen et al.［11］studied the mechanism of the enhanced oil recovery， to further understand the mechanism of the enhanced oil recovery， Ju et al.［12］theoretically analyzed the LHP by changing the wettability of the porous media. There aremany other oil recovery predicting methods， such as the water flooding characteristic curve method［13-15］，and the cash flow method［16］. Although many methods can be used to predict［17］the oil recovery［18］， few oil recovery prediction methods can be used to predict the oil recovery during high or ultra-high water cut stages of a water drive oilfield. Accordingly， we propose a new water flooding characteristic curve method to effectively predict the oil recovery adapted to the high water cut stage in the water drive oilfield.

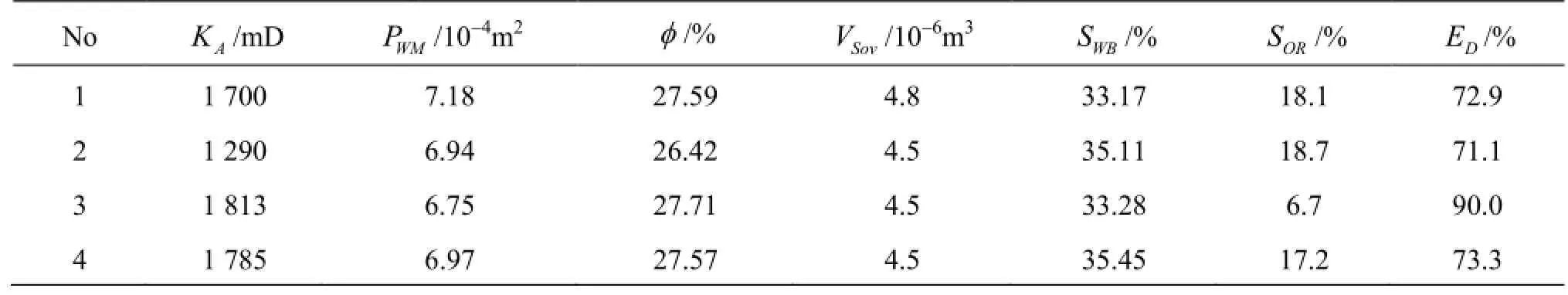
Table 1 Parameters of rock samples

1. New features of phase permeability in high water cut stage

1.1 Traditional phase permeability equation

There is a regression equation between the oil and water phase permeability ratio and the water saturation in many books of petro-physics in China， which takes the form

The coefficient a0and b0in Eq.（1） can be determined by a graphical method. This equation assumes the linear relationship between ln（kro/krw）and Sw. Here， fwis the water cut，krothe oil phase relative permeability，krwthe water phase relative permeability，Swthe water saturation，a0the intercept， and b0the slope.

1.2 Phase permeability experiment in high water cut stage

The water drive phase permeability experiments show that the curve of the oil and water phase permeability ratio against the water saturation， in the semilogarithmic coordinates， has an obvious lower bend after entering the high water cut stage. Hence， the traditional phase permeability equation cannot be used to describe permeability characteristics in the high water cut stage， and a better relational equation between the ratio of the oil and water phase permeability and the water saturation should be established to take account of the “lower bend”， as shown in Table 1 and Figs.1-4.

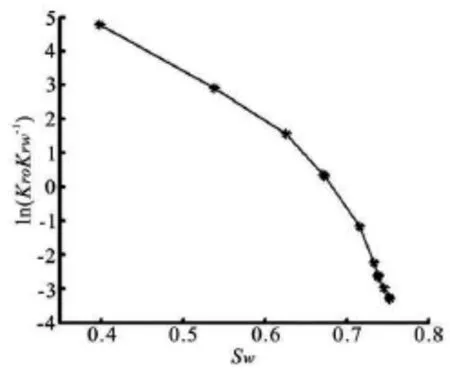
Fig.1 The curve of oil and water phase permeability ratio vs. water saturation of rock sample-1

Fig.2 The curve of oil and water phase permeability ratio vs. water saturation of rock sample-2

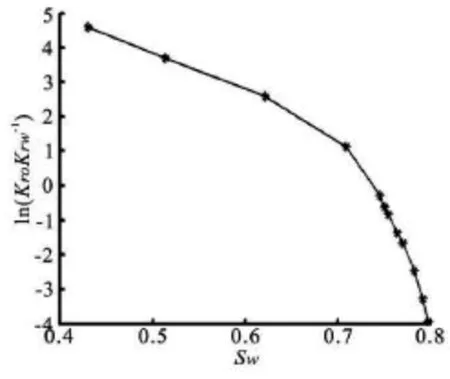
Fig.3 The curve of oil and water phase permeability ratio vs. water saturation of rock sample-3

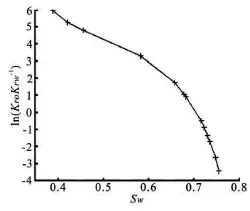
Fig.4 The curve of oil and water phase permeability ratio vs. water saturation of rock sample-4

1.3 Modified relational equation adapted to high water cut stage

Figures 1-4 show the curves of the oil and water phase permeability ratio against the water saturation，in the semi-logarithmic coordinates， where an obvious lower bend can be observed when the water saturation is larger than 0.6. With this lower bend feature， the rational relation between the oil and water phase permeability ratio and the water saturation should be a curve starting with a straight line and ending with a lower bend. The inverse-function between Kro/Krwand Swseems to be in this form. Meanwhile， when entering the high water cut stage， the water saturation is larger than that in the low water cut stage. When Sw→1，Kro→0. The traditional phase permeability equation cannot satisfy this condition， but the inverse function is in the following form

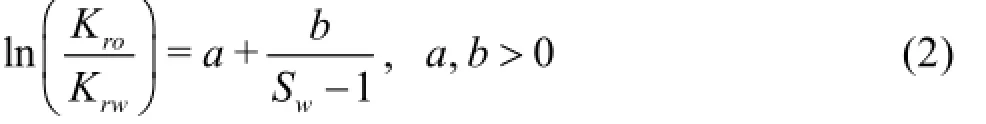
When entering the ultra-high water cut stage，Sw→1， the right hand side of Eq.（2） approaches-∞， so the left hand side must tend to-∞. Actually， the left hand side does tend to-∞， because when Sw→1，Kro→0， ln（Kro/Krw）→-∞. So Eq.（2） can meet the high water cut feature. With the regressionanalysis method， the values of coefficients a， bin Eq.（2） can be determined. Here，a is the intercept， dimensionless， andb， the slope， dimensionless.

2. Predictions of recoverable reserves and oil recovery in high water cut stage

The traditional water flooding characteristic curve method to calculate the oil recovery is inaccurate， because it is based on the traditional phase permeability equation. In the high water cut stage， one sees an obvious lower bend in the semi-logarithmic coordinates. In order to accurately and effectively predict the recoverable reserves and the oil recovery in the high water cut stage， a new water flooding characteristic curve equation based on the modified relational equation is urgently needed.

2.1 New water flooding characteristic curve adapted to high water cut stage

The Darcy equation takes the form：

It is

Substituting Eq.（3） into Eq.（1） gives

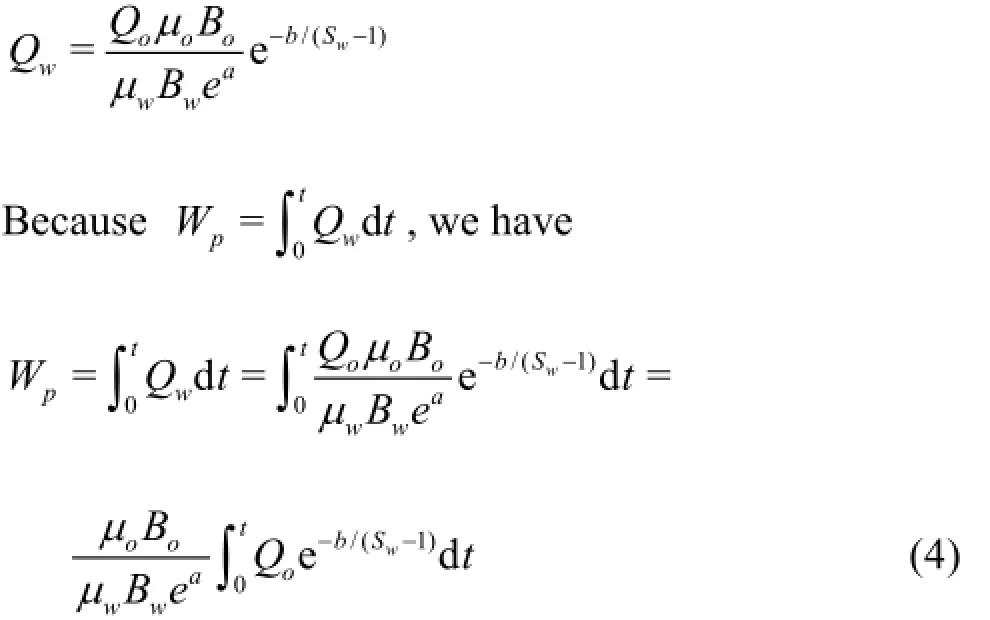
To maintain the reservoir pressure through the water injection，Bo=Boi，Np=100Fhφ（ γo/Boi）（Sw-Swi）. where Np，Wpare the cumulative oil and water productions，F is the oil bearing area，hthe effective reservoir thickness，Sorthe residual oil saturation，Swithe irreducible water saturation，φthe porosity，Soithe initial oil saturation，Swthe average water saturation.Qothe oil production，Qwthe water production， µothe oil viscosity，µwthe water viscosity，Bothe oil formation volume factor and Bwthe water formation volume factor.

According to the 3ΦPOC experiment theory， by using the Buckley-Leverett linear displacement theory and the Welge equation， when the underground oilwater viscosity ratio is in the interval［1，10］， Chen （1985）derived the following equations：

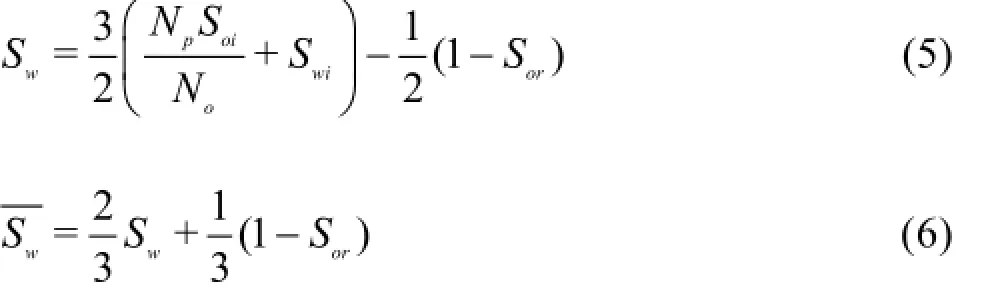
Using the expression

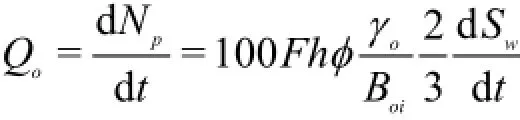
we have

By substituting Eq.（7） into Eq.（4）， we have

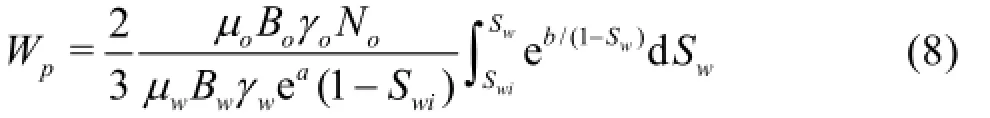
Solving Eq.（8） gives

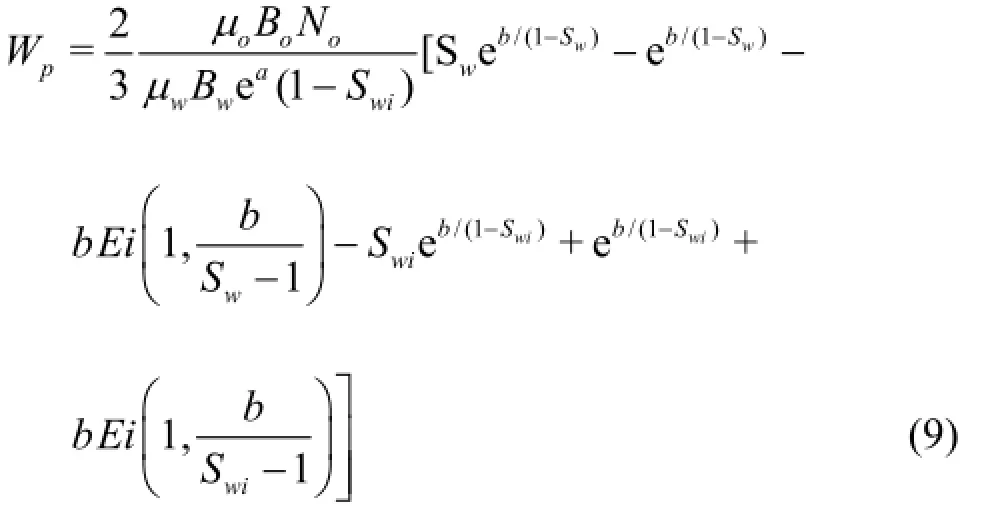
where Ei（·）is the exponential integral function.

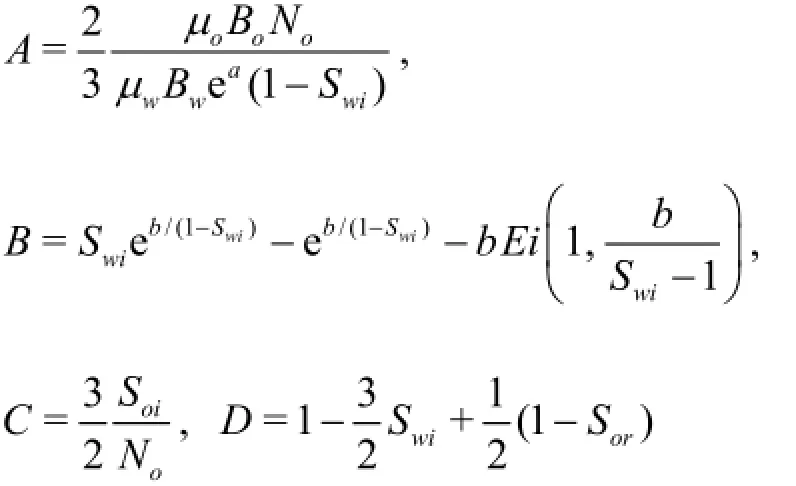
and simplifying Eq.（9）， we obtain

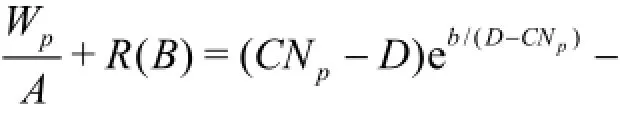
By substituting Eq.（5） into Eq.（9） and denoting

By using the mathematical statisticsmethod for data of more than two hundreds oil field blocks， we have

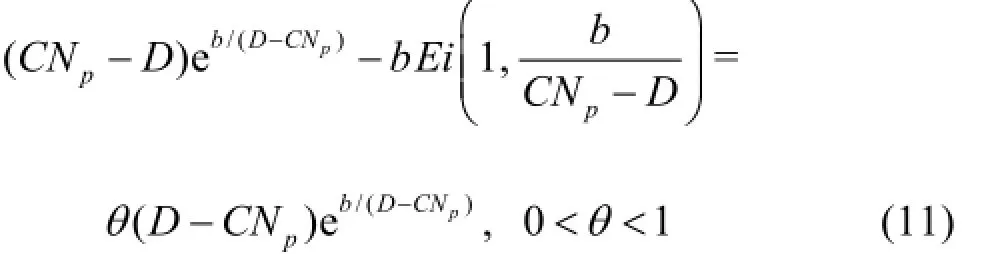
Hence， Eq.（10） becomes

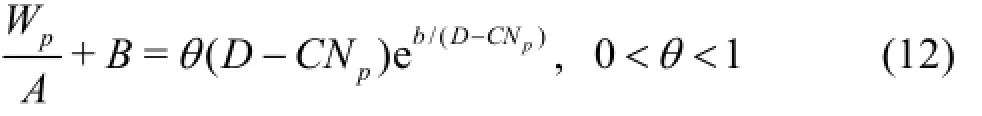
In Eq.（12）， let

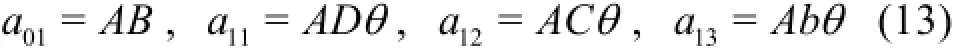
Substituting Eq.（13） into Eq.（12） gives

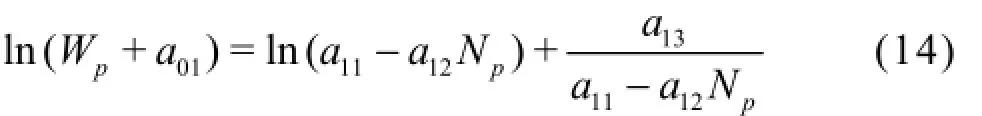
In the high water cut stage， the cumulative water production becomes larger and larger， and because a01takes a fixed value， so the influence of the constants a01can be ignored. Then Eq.（14） becomes

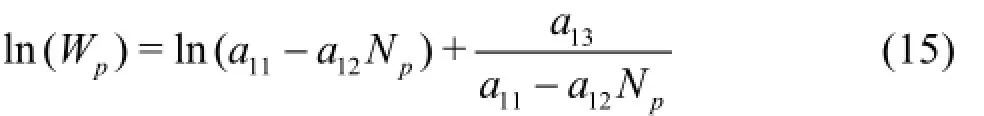
Equation （15） is the new water flooding characteristic curve equation adapted to the high water cut stage.

2.2 Prediction of recoverable reserves and oil recovery in high water cut stage

2.2.1 Study of Np～fwrational equation

The differentiation of Eq.（15） gives

Substututing equations

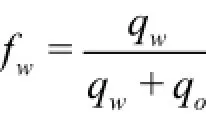
Table 2 Phase permeability data of two different blocks

Table 3 Historical data of two different blocks

and

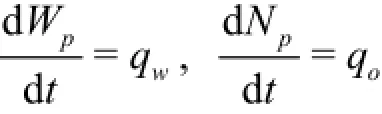
into Eq.（16） gives

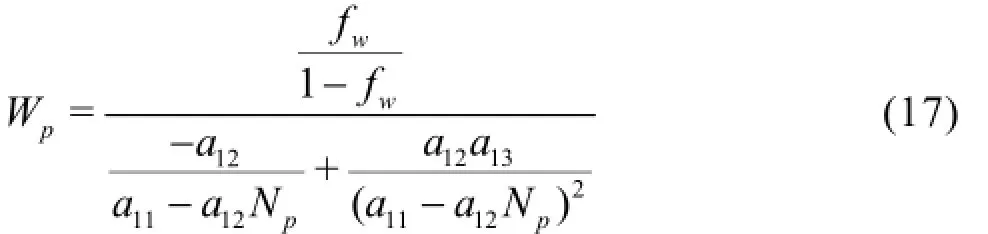
Substituting Eq.（17） into Eq.（15） gives

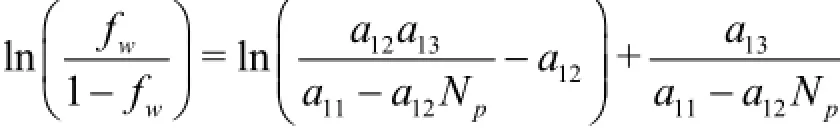
Solving this equation with the mathematical software-MATLAB7.0 gives

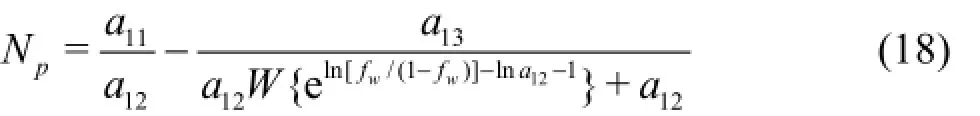
where W（·）is the LambertWfunction.

2.2.2 Prediction of recoverable reserves and oil recovery

Let the economic limit water cut be fw=fw0in the water drive oil field， the recoverable reserves can be predicted by Eq.（18） ， which can be expressed as

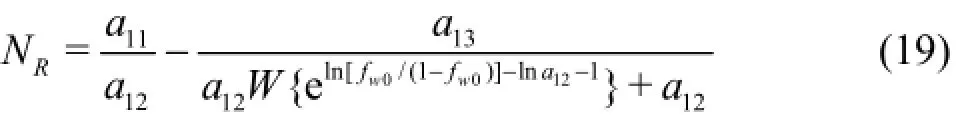
By use of the geological reserves No， the oil recovery can also be expressed as

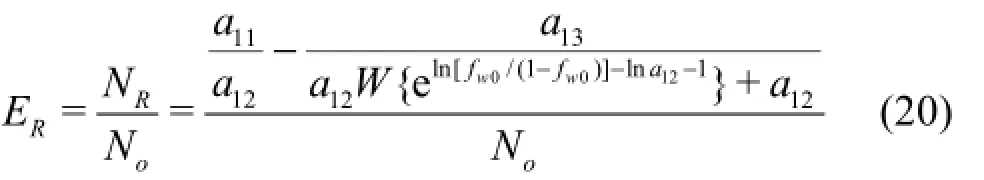
where EDis the displacement efficiency，ERthe recoverable reserves， and NRthe oil recovery. Note： there is a mature method to calculate the economic limit water cut， once the parameters a11，a12，a13are determined， the recoverable reserves and the oil recovery can be predicted.

3. Applications and discussions

3.1 Applications

Here， two water drive reservoir blocks in China are chosen to predict the oil recovery in the high water cut stage. The historical data are shown in Table 2 and Table 3.

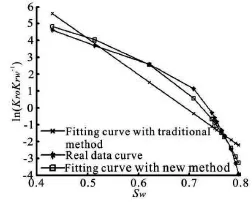
Fig.5 Fitting curve with different phase permeability equations of GD3-block

Fig.6 Fitting curve with different phase permeability equations of J09-block

By using the regression analysis method to Eq.（2）， the values ofaandb can be determined， and the value ofθcan be evaluated according to the statistic characteristics. Here we take θ=0.5（average value）， then the constants a11，a12，a13are determined. If the prediction accuracy is not good enough， the value ofθshould be redefined.

By using the regression analysis method to the data of two different blocks in Table 1， two group values of aandb are obtained， which are （9.3049， 2.5458）for the GD3-block of the SL oilfield and（10.5466，3.1235）for the J09-block of the DG oilfield， as shown in Figs.5-6.

Then the constants a11，a12，a13are determined：

In the GD3-block of the SL oilfield

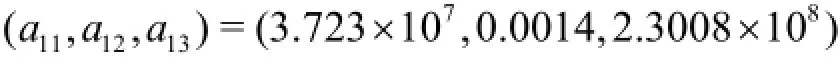
In the J09-block of the DG oilfield

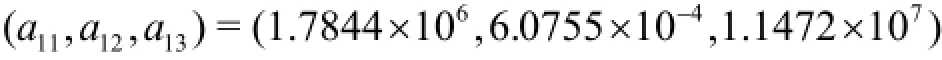
See Fig.7-8.

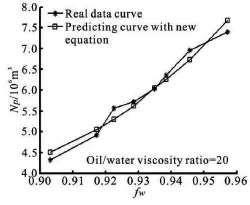
Fig.7 Predicted curve of cumulative oil production of GD3-block

Fig.8 Predicted curve of cumulative oil production of GD3-block

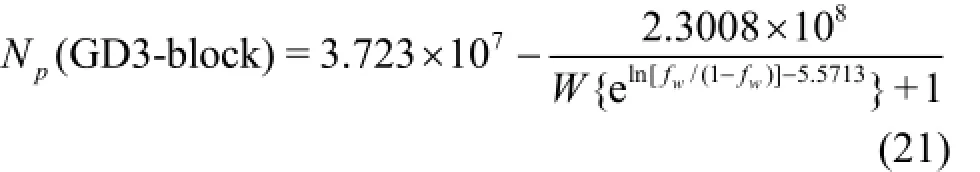
Equation （21） is the Np～fwrelational equation ofthe GD3-block of the SL oilfield.

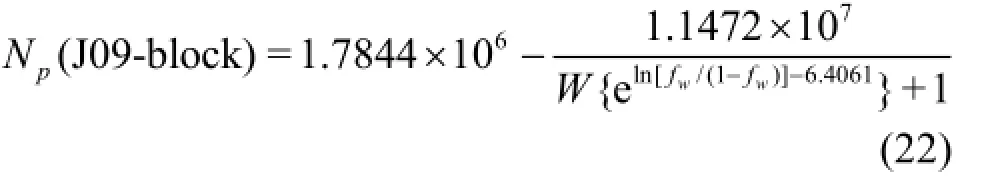
Let the economic limit water cut fw0=0.98， then the oil recovery of these two blocks can also be predicted as：

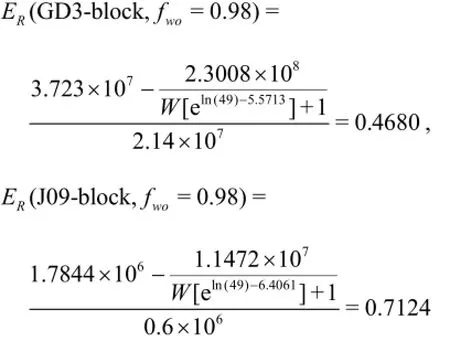
When fw0=0.98， the predicted value of the oil recovery of the GD3-block of the SL oilfield is 46.8% and the predicted value of the oil recovery of the GD3-block of the SL oilfield is 71.24%.

3.2 Discussions

There are near 70 kinds of water flooding characteristic curves， which can be used to forecast the parameters such as the oil recovery， the water cut， and the recoverable reserves and so on， if the coefficients of the equations are reconstructed in the high water cut stage. However， we cannot use them to predict the oil recovery in the high water cut stage， because they are based on the traditional permeability equation for predicting the oil recovery. After entering the high or ultra-high water cut stage of the water drive oilfield，the new water flooding characteristic curve shows that when fw→1，Np→a11/a12， no matter how much the water is injected， the value of the cumulative oil production keeps constant.

Note 1： this new method is just adapted to the high or middle water cut stage of the water drive reservoir， and it cannot be used to predict the recoverable reserves and oil recovery in the low water cut stage， especially at the beginning of the development.

Note 2： six sand rock samples in the high water cut stage are used in the paper to validate the method， including sample DG3 and sample J09， they are DG3 GB361-1， CB32， CB38， L35-5， and J09.

Note 3： the water cut stage is determined by the water cut. The first inflection point reflects the changes of the flooding variatione. Entering the high water cut stage， the first inflection point ensures the new feature.

4. Conclusions

（1） The phase permeability experiments in the high water cut stage shows that the water flooding characteristic curve method based on the traditional regression equation between the oil and water phase permeability ratio and the water saturation is inappropriate to predict the oil recovery in the high water cut stage.

（2） New water flooding characteristic curve equations adapted to the high water cut stage are proposed to effectively predict the recoverable reserves and the oil recovery.

（3） The new oil recovery prediction method is not only adapted to the high water cut stage， but it also reflects the lower bend feature of the phase permeability curve.

［1］JUTTNERI.，RAJKOVICD.Economic and environmental aspects of enhanced oil recovery implementation［J］.Minerals and Energy-Raw Materials Report， 2001， 16（1）： 32-37.

［2］ AL-SULAIMANI H.， AL-WAHAIBI Y. and ALBAHRY S. N. et al. Optimization and partial characterization of biosurfactants produced by bacillus species and their potential for exsitu enhanced oil recovery［J］. SPE Journal， 2011， 16（3）： 672-682

［3］ HOU Jian， ZHANG Shun-kang and DU Qing-jun. A streamline-based predictive model for enhanced-oilrecovery potentiality［J］. Journal of Hydrodynamics， 2008，20（3）： 314-322

［4］AL SHALABI E. W.， GHOSH B. and HAROUNM. et al. The application of direct current potential to enhancing water flood recovery efficiency［J］.Petroleum

［5］ SHAO Chang-guo， GAO Yong-li and LIN Guang-rong. Experimental study on enhancing oil displacement efficiency of waterflooding in ultra-low permeability reservoir［J］. Journal of Xi’an Shiyou University （Natural Science Edition）， 2004， 19（3）： 23-25， 28（in Chinese）.

［6］ SUN Wei， HE Juan. Displacing efficiency and affecting factors of reservoirs in Yan'an formation， Jiyuan region［J］. Oil and Gas Geology， 1999， 20（1）： 26-29（in Chinese）.

［7］ REN Xiao-juan， QU Zhi-hao and SHI Cheng-en et al. Micro-flowing behaviors of water displacing oil in low permeability weak oil-wet reservoirs， Xifeng Oilfield［J］. Science and Technology， 2012， 30（11）： 2160-2168. Journal of Northwest University， 2005， 35（6）： 766-769（in Chinese）.

［8］ LEMON P.， ZEINIJAHROMI A. and BEDRIKOVETSKY P. et al. Effects of injected-water salinity on water flood sweep efficiency through induced fines migration［J］. Journal of Canadian Petroleum Technology，2011， 50（9）： 82-94.

［9］ SALIMI H.， BRUINING J. The Influence of heterogeneity， wetting， and viscosity ratio on oil recovery from vertically fractured reservoirs［J］. SPE Journal， 2011，16（2）： 411-428.

［10］ ZHANG Lie-hui， GUO Jing-jing and LIU Qi-guo. A new well test model for stress-sensitive and radically heterogeneous dual-porosity reservoirs with non-uniform thicknesses［J］. Journal of Hydrodynamics， 2011，23（6）： 759-766

［11］ SHEN P.， WANG J. and YUAN S. et al. Study of enhanced-oil-recovery mechanism of alkali/surfactant/polymer flooding in porous media from experiments［J］. SPE Journal， 2009， 14（2）： 237-244

［12］ JU Bin-shan， FAN Tai-liang and MA Ming-xue. Enhanced oil recovery by flooding with hydrophilic nanoparticles［J］. China Particulology， 2006， 4（1）： 41-45.

［13］ CHEN Y. Q. Calculation methods of recoverable reserves of oilfields［J］. Xinjiang Petroleum Geology，2000， 21（2）： 130-137（in Chinese）.

［14］ WANG Jun， HUANG Sui-liang. Effect of soil water characteristic models on numerical modeling of unsaturated flow［J］. Chinese Journal of Hydrodynamics， 2010， 25（1）： 16-19（in Chinese）.

［15］ LIU Ying， Gao Wen-jun. A systematic classification of water flood characteristic curve methods［J］. Xinjiang Petroleum Geology， 2007， 28（5）： 618-621（in Chinese）.

［16］MUSTAFA V. K. Recovery calculations of oil fields using predictive models［J］.Energy Sources， Part B： Economics， Planning， and Policy， 2006， 1（4）： 335-339.

［17］LIU H.，LIU Z.and DING X.A new oilfield production prediction method based on GM（1，n）［J］. Source： Petroleum Science and Technology， 2014， 32（1）： 22-28.

［18］ LIU H. New water-oil displacement efficiency prediction method［J］. Open Petroleum Engineering Journal， 2014， 7： 88-91.

* Project supported by the China Postdoctoral Science Foundation （Grant No， 2014M562509XB） the Scientific Project of Sichuan Provincial Education Department （Grant No. 15ZB0447）.

Biography： LIU Zhi-bin （1962-）， Male， Ph. D.， Professor

LIU Hao-han，

E-mail： tsinghua616@163.com