重组竹的单轴与纯剪应力应变关系
2015-02-18盛宝璐,周爱萍,黄东升

盛宝璐,周爱萍,黄东升
(南京林业大学 土木工程学院,南京 210037)
摘要:重组竹是将竹丝束平行组坯、经高压胶合而成的一种生物质复合材料,是一种极具潜势的建筑结构材料。研究重组竹的基本力学性能和应力应变关系,是建立此类材料本构关系和进行重组竹结构非线性分析的基础。将重组竹理想化为横向各向同性复合材料,通过试验,给出了重组竹各主轴方向的单轴与各主平面的纯剪力学参数,建立了各种应力状态下的应力应变关系。结果表明,重组竹力学性能优于常用的结构用木材,且变异性较小。重组竹顺纹受拉强度约是顺纹受压强度的2倍;横纹受拉强度远远低于横纹受压强度;横切面内的剪切模量及强度远远低于另外两个方向,且横纹剪切强度是顺纹剪切强度的3倍。重组竹的应力应变关系和破坏模式与纤维参与受力程度密切相关。顺纹受拉时,拉应力完全由纤维承担,破坏表现为纤维的脆性拉断,强度最高,应力应变为完全线性关系;其他应力状态下,破坏均发生在基体或纤维-基体界面,若裂纹的扩展受到纤维限制,破坏呈渐进性,强度较低,应力应变曲线由早期的线性关系转入后期的非线性关系;当裂纹的扩展未受到纤维限制,破坏强度最低,应力应变呈线性关系。
关键词:重组竹;应力应变关系;破坏模式;横观各向同性复合材料
Received:2015-07-30
Foundation item:National Natural Science Foundation of China (No.51378263);National Promotion Program of Forestry Science and Technology Achievements(No.[2015]21).
竹材是一种可再生、可降解的天然生物质复合材料,一般3~5年就可以成材,具有硬阔叶树材的诸多优良特性,其比强度和比刚度高于钢材,是一种理想的绿色高强建筑材料。竹结构建筑的环境负荷远小于钢材、混凝土等传统建筑。但原竹材壁薄中空,几何变异性大、含糖高易虫蛀、质地不均匀、耐久性较差,不能满足现代工程结构对构件的力学性能和几何构形要求。原竹剖割成约长2 m、宽15 mm、厚3 mm的竹蔑,经80 ℃恒温烘干至含水率低于11%,再将竹篾碾压成竹丝束,同方向平行组坯并浸渍酚醛树脂胶,通过高温热压胶合,制成重组竹[1-3]。由于在工业化制造的过程中,竹材经过筛选,剔除了原竹的缺陷,且含水率低,故重组竹力学性能均匀、变异性小、强度高、耐久性好,很少出现收缩、翘曲、开裂等现象。重组竹可以加工成不同截面的型材,适用于房屋的梁、柱及跨度较大的构件[4-6]。因此,重组竹可以满足现代建筑结构对材料的力学、环保与耐久性等方面的性能要求。研究表明,重组竹结构在节能环保、工业化生产、装配式施工等方面有着传统材料不可替代的优势,以重组竹作为结构材料建造多层甚至高层建筑是极具潜势的发展方向。

此外,结构服役期内,材料常常处于复杂应力状态。作为复杂应力状态下纤维定向复合材料的破坏准则,常包含多个材料常数。如,Hill[11]提出的正交各向异性材料破坏准则,包涵了6个弹性常数,由于Hill准则忽略了材料拉、压性质的差异,故不适用于重组竹材料强度分析。Hoffman[12]考虑了材料拉、压力学性能的差异,提出了如式(1)的破坏准则。
(1)


1重组竹力学性能的描述
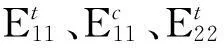
度S23、S31和S12。上述所有弹性常数和强度参数均可通过重组竹3个主方向的单轴拉、压和3个主平面纯剪试验得到。
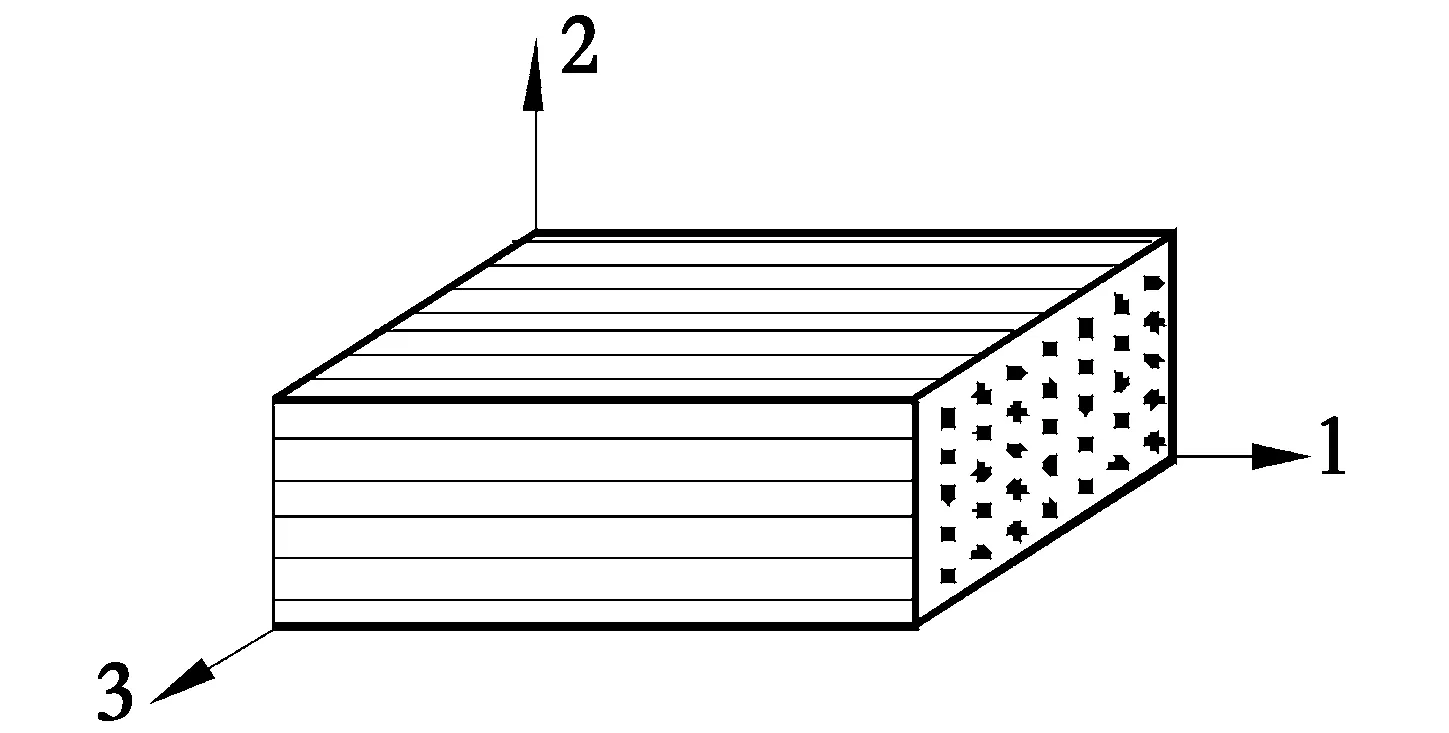
图1 重组竹3个材料主方向Fig.1 Main direction of PSB
2重组竹基本力学性能试验
试验竹材取自浙江安吉5年生毛竹(phyllostachys),按照重组竹标准制作工艺制成试验材料,试件的密度和含水率分别为11.0 kN/m3和 8.0%。每个试验类型的试件为30个,试件构形见表1。单轴试验参照ASTM D143-09[17]、剪切试验参照ASTM D7078标准[18]进行。采用TDS-530多通道数据采集箱同时采集荷载、变形和应变,采集频率为1 Hz。各类型试验过程与现象详见表2。

表1 试件的尺寸、形状和相关参数计算公式
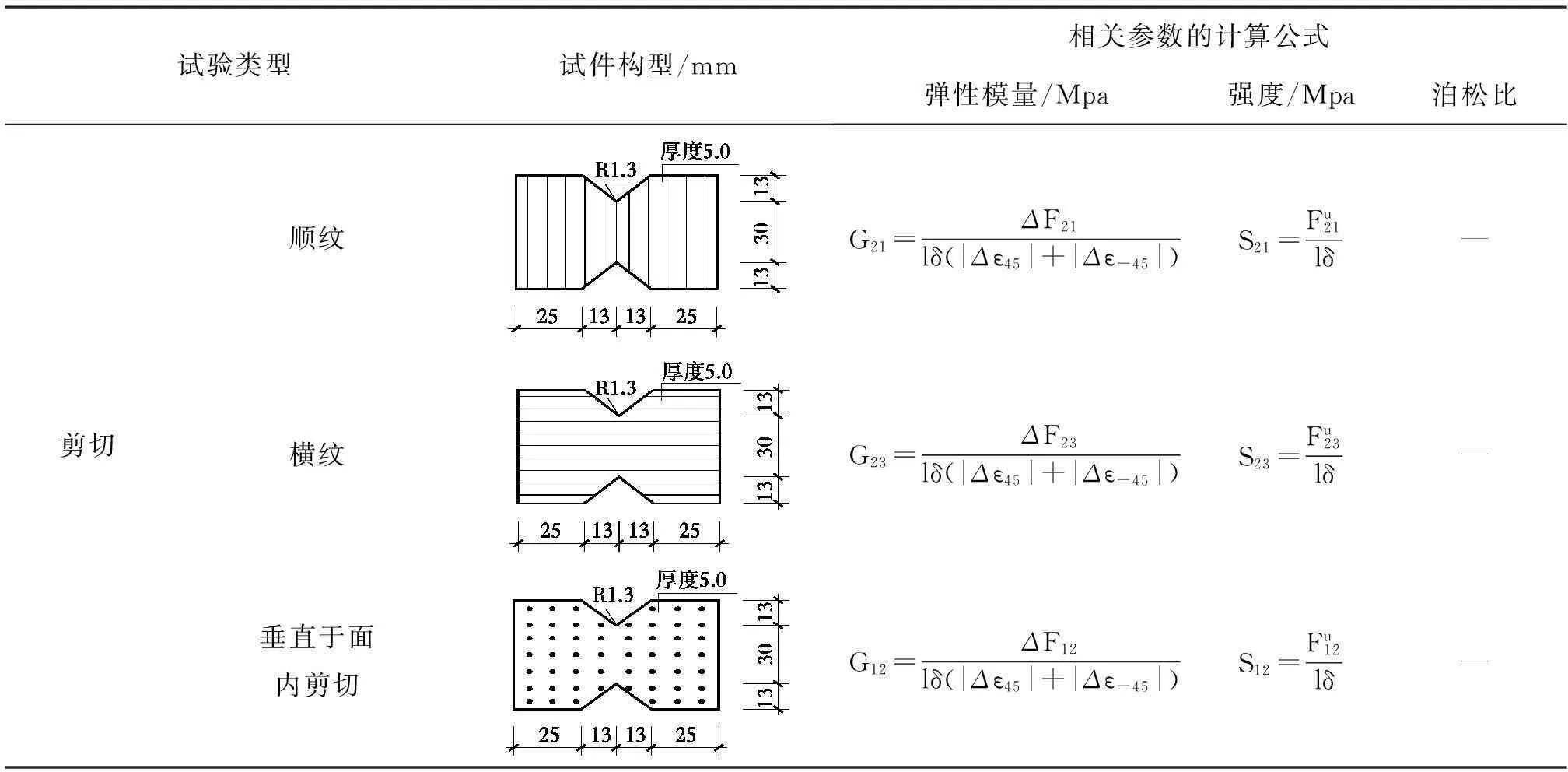
续表1
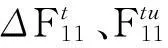

表2 试验过程与现象

续表2
3试验结果与分析
3.1 主要力学性能参数
统计分析表明,各类型试验的力学参数基本呈正态分布,图2给出了横纹受压力学参数的正态检验结果。表3为试验测得的力学参数统计分析结果。可以看出,重组竹顺纹拉、压模量差异很小,顺纹受拉强度约为顺纹受压强度的两倍;横纹受拉弹性模量约是横纹受压弹性模量的两倍,横纹受拉强度远远低于横纹受压强度;顺纹剪切弹性模量与横纹剪切弹性模量非常接近,顺纹剪切强度远远低于横纹剪切强度,而横切面内的剪切模量及强度远远低于另外2个方向。
表4将重组竹与常用的结构用木材性能[19]进行了比较,可以看到,重组竹顺纹弹性模量高于大部分的常用的结构用木材,而强度远高于所有常用的
结构用木材;重组竹材料力学参数的变异性比木材小。因此,重组竹的性质优于木材,是一种理想的建筑结构材料。

图2 横纹受压正态分布检验图Fig.2 Gaussian distribution test for compressionin

试验类型弹性模量均值/MPaCV/%泊松比均值/MPaCV/%比例极限应力应变均值/MPaCV/%均值/MPaCV/%峰值强度应力应变均值/MPaCV/%均值/MPaCV/%极限强度应力应变均值/MPaCV/%均值/MPaCV/%顺纹受拉受压受剪横纹受拉受压受剪垂直于平面内剪切10296140.37118.4201.26131189081733.0930.28665.5391.401955.7682.07221361188.21190.77253066280.34.43150.28271365147.68100.59923.14153.50151446139.73150.78523.44103.7634746143.64100.6214
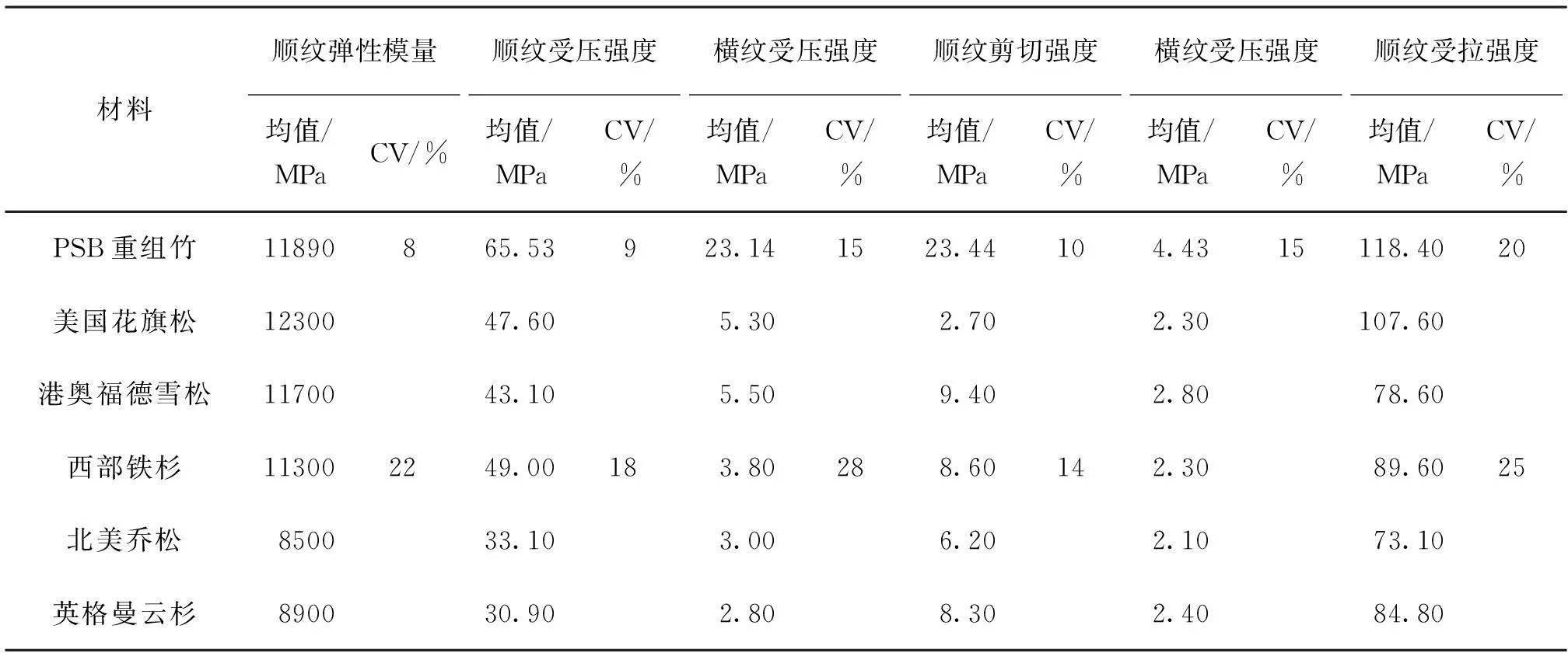
表4 重组竹与结构木材的力学性能比较
3.2 应力-应变关系
(2)
(3)
(4)
式中:系数λi和βi(i=1, 2, 3), 可以由下式确定
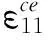
(5)

图3表示上述解析公式得到的曲线与试验曲线的比较。可以看出,两者吻合良好。
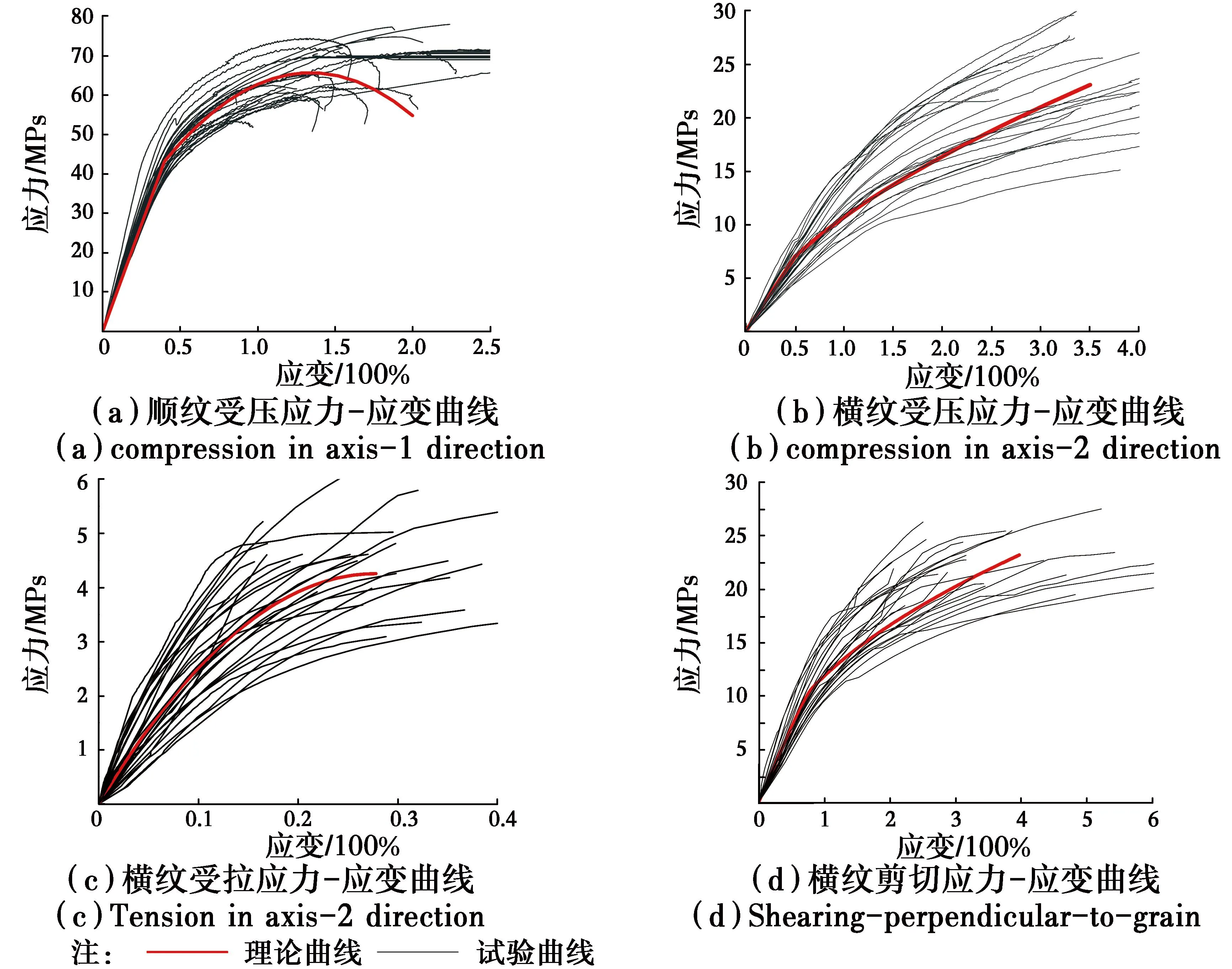
图3 应力应变曲线曲线的比较Fig.3 Compare the calculating stress-strain curves to those obtained by
4结论

重组竹顺纹拉、压弹性模量相差无几,顺纹抗拉强度大约是横纹抗压强度的2倍,横纹抗拉强度低于横纹抗压强度;顺纹、横纹剪切弹性模量相近,大约是顺纹抗拉弹性模量的1/8;顺纹抗剪强度大大低于横纹抗剪强度,横切面内的抗剪弹性模量与强度都低于另外两个方向的剪切强度与剪切模量。
参考文献:
[1] 张宏健, 李君, 叶喜. 竹条重组枋生产工艺的研究开发[J]. 建筑人造板, 1998(3):24-26.
Zhang H J,Li J,Ye X.Research on the fabricated technique of reformed bamboo[J].Building Artificial Boards,1998(3):24-26.(in Chinese)
[2] 李琴, 汪奎宏. 重组竹生产工艺的初步研究[J]. 人造板通讯, 2001(7): 6-9.
Li Q ,Wang K H.Research on the manufacture process of reformed bamboo[J].China Wood-based Panels,2001(7):6-9.(in Chinese)
[3] 叶良明, 姜志宏, 叶建华. 重组竹板材的研究[J]. 浙江林学院学报, 1991, 8(2):133-140.
Ye L M,Jiang Z H,Ye J H.Study on the laminated bamboo panel[J].Journal of Zhejiang A & F University,1991,8(2):133-140.(in Chinese)
[4] Shigeyasu A D, Sun U T. Fracture properties of bamboo[J]. Composites: Part B. 2001, 32: 451-459.
[5] Zhou A P, Huang D S, Li H T,et al. Hybrid approach to determine the mechanical properties of fibers and matrixes of bamboo [J]. Construction and building materials, 2012, 35: 191-196.
[6] Zheng M H . Micromechanical prediction of ultimate strength of transversely isotropic fibrous composites[J]. Solids and Structures, 2011, 38: 4147-4172.
[7] Amada S, Lchikawa Y, Munekata T, et al. Fiber texture and mechanical graded structure of bamboo[J].Composites Part B, 1997,28(1):13-20.
[8] Abdul H P S, Bhat I U H, Jawaid M, et al. Bamboo fiber reinforced biocomposites: A review[J]. Materials and Design,2012, 42: 353-368.
[9] Yokozeki T, Ogasawara T, Ishikawa T. Effects of fiber nonlinear properties on the compressive strength prediction on unidirectional carbon-fiber composites[J]. Composite Science and Technology, 2005, 65: 2140-2147.
[10] Canadian Wood Council. Wood design manual[S]. Ottawa, Ontario, Canada,2010.
[11] Hill R. The mathematical theory of plasticity [M]. Oxford University Press, Lodon, 1950.
[12] Hoffman O. The brittle strength of orthotropic materials[J]. J. Compos. Mater. 1967, 2: 200-206.
[13] Naik N K,Kumar R S. Compressive strength of unidirectional composites: evaluation and comparison of prediction modes[J]. Composite Structures, 1999, 46: 299-308.
[14] Shioya M, Takaku A. Estimation of fiber and interfacial shear strength by using a single-fiber composite[J].Composites Science and Technology. 1995: 33-39.
[15] Gundel D B, Waner F E. Experimental and theoretical assessment of the longitudinal tensile strength of unidirectional Sci-Fiber / Titanium-matrix[J]. Composite Science and Technology. 1997, 57: 471-481.
[16] Matzenmiller A, Lubliner J, taylor R L. A constitutive model for anisotropic damage in fiber-composites[J]. Mechanics of Materials. 1995, 20: 125-152.
[17] American Society For Test Materials (ASTM). Standard test method for small clear specimen of timber, D143-09[S].
[18] American Society for Test Materials (ASTM). Standard test method for shear properties of composite materials by V-notched rail shear method. D7078/D7078M-12[S].
[19] United States Department of Agriculture, Forest Products Laboratory. Wood Handbook:Wood as an Engineering Material[M].Centennial Edition, 2010.
(编辑胡玲)
Author brief:Sheng Baolu(1990-),PhD candidate,main research interest:mechanics performance analysis of bamboo/wood structure,(E-mail)baolu.sheng@yahoo.com。
Zhou Aiping(corresponding author),associate professor,PhD,(E-mail)zaping2007@163.com。
Stress-strain relationship of parallel strand bamboo under uniaxial or pure shear load
Sheng Baolu, Zhou Aiping, Huang Dongsheng
(College of Civil Engineering, Nanjing Forestry University, Nanjing 210037, P.R. China)
Abstract:Parallel Strand Bamboo (PSB) is a biocomposite composed of long narrow parallel bamboo strands which are adhesively bonded under high pressure. It has more and more attractive structural applications in building and construction engineering. It’s important to well understand the stress-strain relationship to develop the constitutive law and conduct the nonlinear analysis of PSB structures. The PSB was treated as an transversely isotropic composite in the experiment and the uniaxial parameters in each main material axis and the pure shearing parameters in each main material plane were proposed, and the corresponding stress-strain relationships of each stress state were also established. The results show that compared with common used woods in construction engineering, PSB has higher strengths with less variability. Strength of tension parallel to grain is nearly as twice as that of compression parallel to grain. In perpendicular to grain direction, the strength of tension is much lower than the that of compression. Shearing in transverse-to-grain plane presents lowest modulus and strength than those shearing in other two directions. The shearing strength in perpendicular to grain is as about 3 times as that of shearing in parallel to grain direction. The stress-strain relationships and the failure modes of PSB are significantly depended on the way in which the fiber participated. In parallel to grain direction, tensile damage almost entirely contributes to the broken of fibers, which shows the highest strength and brittle behavior among all stress states. In other cases, when the expending of failure cracks are restricted by fibers, the damage presents progressive process and higher strength, and the stress-strain relationships exhibit linearity in the earliear life while turn to nonlinearity in the later life of the specimens. When damages take place in matrix or in fiber-matrix interface without fibers involved in, the material shows lower strength, and the stress-strain curves present linear and brittle behavior.
Key words:Parallel strand bamboo;stress-strain relationship;failure mode;transversely isotropic composite
通信作者周爱萍(),副教授,博士,(E-mail)zaping2007@163.com。
作者简介:盛宝璐(1990-),博士生,主要从事现代竹木结构研究,(E-mail)baolu.sheng@yahoo.com。
基金项目:国家自然科学基金(51378263);林业科学技术成果国家级推广项目([2015]21号)。
收稿日期:2015-07-30
中图分类号:TU502
文献标志码:A
文章编号:1674-4764(2015)06-0024-08
doi:10.11835/j.issn.1674-4764.2015.06.004
