The Distribution of Benefits for Players in Agricultural Industrial Chain

Wanming LI，Huibo SUN

1.School of Economics and Management，Shihezi University，Shihezi832003，China；2.The Research Centre of Oasis Development，Shihezi University，Shihezi832003，China

With the development of agriculture and rural economy，the building of agricultural industrial chain plays a key role in promoting agricultural industrialization，marketization process，farmers' income，agricultural efficiency and rural advances.Fu Guohua［1］developed the concept of agricultural industrial chain for the first time，and he believed that agricultural industrial chain was that around a＂competitive product＂，planting，processing，transportation and marketing industry relied on market resources，and gathered land，labor，capital and other production elements to implement large scale operation and chain rotation.Zuo Liangjunet al.［2］believe that the agricultural industrial chain includes the agricultural production，processing，circulation and consumption.The agricultural industrial chain is an organic whole combining the value chain，information chain，logistics chain and organizational chain，covering agricultural production，processing，marketing，transportation and many other links，involving various sectors and organizations during the whole process of agricultural production.In the course of economic operation，the players of agricultural industrial chain cooperate based on division of labor in order to adapt to market changes and complete unified strategic objectives，thus forming a strategic alliance relation and a marketoriented agricultural production and management mechanism［3］.This strategic alliance can integrate the resources owned by scattered members，improve the operational efficiency of the industrial chain，increase revenue and reduce risk.Meanwhile，the stable strategic alliance can make the agricultural industrial chain more stable and standardized，overcome the looseness and vulnerability of agricultural industrial chain，ensure the players' interests，expand and extend the industrial chain，and enhance the function of agricultural industrial chain.The key to maintaining and consolidating this strategic alliance is the distribution of interests among the players of industrial chain due to cooperation，but all members under this agricultural production mechanism are rational economic man，each pursuing profit maximization，inevitably leading to conflicts of interest，rupture of the alliance，and breaking of industrial chain.Only when the players get fair and equitable distribution of benefits under the cooperative game can a strong stable alliance be formed.Shapley proposed Shapley value method as an effective solution to the problem of distribution of benefits for the players of agricultural industrial chain under cooperative game.This distribution method based on Shapley value method is a mode based on the marginal contribution of each player involved in the production process，and it has certain rationality［4］.

1 Shapley profit distribution model

There is a cooperative game relationship between the players of agricultural industrial chain，and Shapley value method is just a mathematical method to solve the problem ofn-person game.The Shapley value is characterized by a collection of desirable properties.The setup is as follows：a coalition of players cooperates，and obtains a certain overall gain from that cooperation.Since some players may contribute more to the coalition than others or may possess different bargaining power（for example threatening to destroy the whole surplus），what final distribution of generated surplus among the players should arise in any particular game？Or phrased differently：how important is each player to the overall cooperation，and what payoff can he or she reasonably expect？The Shapley value provides one possible answer to this question.Shapley value method is a program to distribute the maximum benefit stemming fromn-person cooperation［5］.

1.1 Overview of Shapley value modelAssuming the setR＝｛1，2，，3，…，n｝，and there is a real valued functionU（X）that corresponds to any subsetXofR.If U（∮）＝0，U（Xi∩Xj）≥U（Xi）＋U（Xj），Xi∩Xj＝∮，（Xi∈R，Xj∈R），then［R，U］is called multiplayer cooperative game，Uis characteristic function，andU（X）is the return value of cooperative allianceX.Pirepresents the income in the maximum benefitU（R）obtained by memberiinRdue to cooperation.On the basis of cooperationR，P＝（P1，P2，P3，…，Pn）is the allocation strategy of cooperative games.

Obviously，to ensure cooperation between members，it must have the following two characteristics：

whereU（i）is the profit when there is no alliance between members.

In the Shapley value method，the value of benefits obtained by players under cooperationRis called Shapley value，denoted as Φ（U）＝（P1（U），P2，P3（U），L，Pi（U）），wherePi（U）is the benefits obtained by cooperative memberi.Shapley value meets the following three axioms.

（i）Symmetry.Assumingφiis a permutation ofPφi（U）＝Pi（U），thenRis the correspondence of its own.Ifφiis the correspondence ofi，φXis the correspondence ofX（X⊂R），denoted asU（φX）＝V（S），then fori＝1，2，3，Ln，there isPφi（U）＝Pi（U）.The benefit of each player is nothing to do with the assigned serial numberi，that is，the relationship between the players is equal.

（ii）Effectiveness.If there isPi（U＋V）＝Pi（U）＋Pi（V）for the subsetXcontainingi，thenPi（U）＝0 and（R）.This means that if the players contribute nothing to the cooperative earnings，then the allocated benefit is zero，and the sum of benefit allocated to the players is equal to the whole cooperative benefit.

（iii）Additivity.For any two characteristic functionsUandVdefined onR，there isPi（U＋V）＝Pi（U）＋Pi（V），i＝1，2，3，L，n.This shows that when many players simultaneously conduct two kinds of cooperation，the benefit obtained by everyone should be the sum of benefits allocated from two kinds of cooperation.

Shapley value only exists for anyn-person cooperative game strategy，and in the cooperationR，the benefitPi（U）allocated to playerican be calculated as follows：

whereXiis the subset containingiinR；｜X｜is the number of elements contained in subsetX；w（｜X｜）is the probability，which can be seen as a weighting factor；U（X）－U（X－i）is the benefit stemming from playerijoining the alliance，namely the marginal contribution of playeri.

1.2 The application of Shapley value method in the distribution of benefits in agricultural industrial chainIn order to better use and understand Shapley value，assuming there are three companies A，B，C，operating independently，and they can obtain the benefit of 100000 yuan，respectively；if A cooperates with B，they can get benefit of500000 yuan；if A cooperates with C，they can get benefit of 700000 yuan；if B cooperates with C，they can get benefit of 400000 yuan；if the three companies cooperate，they can get benefit of 1000000 yuan.If the benefit of 1000000 yuan obtained by the three companies due to cooperation is equally distributed，then each company can get 333000 yuan，greater than the profit when operating alone，but it is difficult to mobilize the enthusiasm of some of the players.If the sum of benefit of A and C is less than the benefit of700000 yuan due to cooperation between the two，A and C must not participate in the three-player cooperation.Shapley value method can solve this problem，and according to Shapley value method，the benefit allocated to player AP1（U）is calculated as shown in Table 1.

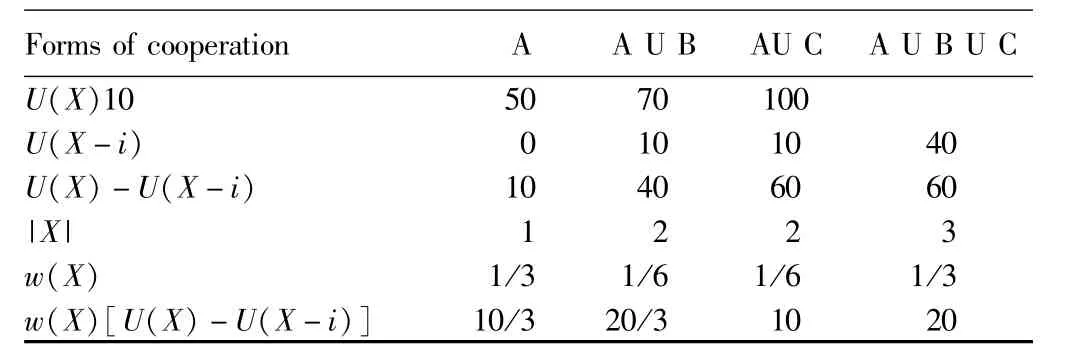
Table 1 Calculation of benefit allocated to A using Shapley value method

By totaling the figures in the last line of table according to formula，we can get A's benefit as follows：

Through the verification，the sum of benefits of A，B and C is equal to their cooperative benefits，namelyP1（U）＋P2（U）＋P3（U）＝100（104yuan），P1（U），P2（U）andP3（U）and are all greater than the income from independent operation 10（104yuan）；P1（U）＋P2（U）＞50（104yuan），P1（U）＋P3（U）＞70（104yuan），P2（U）＋P3（U）＞40（104yuan）.

Therefore，the distribution of benefits based on Shapley value method has made the benefits of three cooperative companies more than the benefits obtained from independent operation of one single company or the benefits obtained from the cooperation of any two companies.Consequently，the three companies are motivated to participate in cooperation，thereby ensuring the stable coalition.

2 Correction of Shapley value method

Through the above analysis，we can see that Shapley value method gives full consideration to the contribution of each player of agricultural industrial chain to cooperation in varying degrees，which is conducive to mobilizing the enthusiasm of members for partici-pating in cooperation.However，Shapley value method does not consider the risk tolerance，technological innovation capacity and cooperation players，and these factors will have an impact on the distribution of benefits，so there is a need to adjust the distribution of benefits based on Shapley value method to make it more reasonable.

2.1 RiskfactorsDuring the agricultural industrial chain operation，players will always face environmental risks，decision risks，market risks and other uncertainties，and the risks faced by players are different.In Shapley value method，the risks borne by players are seen as the same（1/n），and the differences in the distribution of benefits are ignored when distributing benefits，which will inevitably lead to a mismatch between benefits and risks.Therefore，in accordance with the risk-sharing principle，it is necessary to increase benefits for the players assuming great risks and reduce benefits for the players bearing small risks.In the evaluation of risk，we can use fuzzy comprehensive evaluation method，AHP，relative risk allocation method and other methods.Riis used to represent risk factor，and the normalization is performed.The risk factor can be expressed as：

2.2 TechnologicalinnovationcapabilityFacing complex and volatile market environment，the development of new products，introduction of new technologies and technological innovation of one link in the agricultural industrial chain，will have an impact on the whole industrial chain，and increase the overall benefits of cooperation.Therefore，the agricultural industrial chain must have core competitiveness，and the technological innovation is a critical factor.There is a need to take into account the technological innovation capacity during the distribution of benefits，rewarding the players for introducing new technologies or carrying out technological innovation，and punishing the players for the lack of new technology introduction or technological innovation.It is necessary to consider the player's technological innovation capacity status in the whole industrial chain，and the level of collaboration，as well as the contribution of player's technical innovation to the overall cooperation earnings.Wican be used to represent the value added for playeridue to the technological innovation，andWiis normalized.We get the share of contribution of each player to technological innovation as follows：

2.3 ThedegreeofcooperationCooperative members may show different levels of enthusiasm and effort in the process of participation and cooperation，or withdraw from cooperation at any time，which is bound to affect the stability of cooperation，and cause great losses to the entire agricultural industrial chain.During the distribution of benefits，it is necessary to ensure the stability of cooperation，giving appropriate incentives for the players with high enthusiasm for participating in cooperation，and punishing the players with low enthusiasm.We can make assessment from players' in put，information openness and level of trust，conduct quantitative analysis of the assessment results，and score each player in terms of the level of cooperation.fi（i＝1，2，3，L，n）is used to represent the assessment result about cooperation level of playeri.Through the normalization，we can geas the influence coefficient of cooperation level.

2.4 CorrectionmodelThe above three factors play different roles in the distribution of cooperation benefits，and we can use scientific and rational approaches to give a certain weight.According to the different roles of players in the agricultural industrial chain，the Delphi method is used to set the weight，and the weight of three factors can be expressed asc＝（c1，c2，c3），then the correction model can be expressed as follows：

wherePi（U）′is the benefit allocated to playeriafter correction；diis the actual influencing factor of playeri；di＝－is the difference between the actual influencing factor of playeriand theoretical sharing factor（If it is greater than 0，it means that after taking into account the comprehensive factors，the players' performance is good，and it is necessary to increase interest allocation；if it is less than 0，it means that the players' performance level is less than the average performance level of players in the industrial chain，and it is necessary to lower interest allocation.）；U（R）×（－）is the compensation value of profit distribution.

After verification，the corrected actual distribution of benefitsis used to correct the above cases.Assumingai＝（0.1，0.3，0.3），si＝（0.3，0.4，0.3），bi＝（0.3，0.5，0.2）the weight of the three factors isci＝（0.4，0.4，0.2）.The comprehensive factor after integrating the three factors is as follows：

Based on the calculation results using Shapley value method，we can get the adjusted A，B，C's benefit distribution amount as follows：

After adjustment，the benefits allocated to A and B increase，while the benefits allocated to C decrease.It is the result of comprehensive consideration of risk factors，technological innovation capacity and level of cooperation，and the distribution of benefits is more equitable.

3 Conclusions

The benefit distribution mechanism of agricultural industrial chain is an important factor affecting the stability of industrial chain.For common interest，the players interact and collaborate and enter into a strategic alliance，ultimately forming a complete chain.The fundamental goal of players' participation in agricultural industrial chain is to rely on cooperation to create greater overall benefits while seeking to maximize their own interests.The nature of members' maximization of their own interests will be bound to make them concerned about the mutual distribution of benefits，and if the distribution of benefits is unreasonable，it will affect the enthusiasm of players for participating in cooperation，thereby affecting the overall interests of the entire chain，or even breaking the industrial chain.Therefore，the reasonable distribution of benefits can ensure the stable operation of agricultural industrial chain.In the cooperative game relationship，Shapley value method provides a reasonable allocation strategy for the distribution of benefits between cooperative members.On the basis of Shapley value，this paper considers the risk factors，technological innovation capacity and level of cooperation faced by the players in agricultural industrial chain，and corrects the Shapley model to make the benefit distribution strategy lay equal emphasis on efficiency and fairness.In short，Shapley value method can provide a theoretically and practically feasible benefit distribution program for the distribution of benefits among players in agricultural industrial chain，reduce the irrational factors in the distribution of benefits，and lay a solid foundation for the stable and continuous cooperation.

［1］FU GH.Operating agricultural products industry chain，improving agricultural system benefit［J］.China State Farms Economy，1996（11）：24－25.（in Chinese）.

［2］ZUO LJ，ZHANG LJ.Analysis on the influence of the management of agricultural products in supermarkets on agricultural products industry chain［J］.Rural Economy，2003（3）：31－32.（in Chinese）.

［3］ZHAO XF，WANG YP.On the difference and relation among agricultural chain，industrialization and industry system［J］.Rural Economy，2004（6）：44－45.（in Chinese）.

［4］ZHANGRH，LUORG.Research on benefit assignment of common delivery based on Shapley valuemethod［J］.Journal ofWuhan University of Technology，2008，30（1）：150－153.（in Chinese）.

［5］CHEN HH，TIAN ZH，ZHOU J.Study on the profitallocation of traceability system for vegetables based on the Shapley value——Taking the case of T company of Beijing City［J］.Journal of Agrotechnical Economics，2011（2）：56－65.（in Chinese）.