一类半无穷区间上分数阶非线性微分方程边值问题多个正解的存在性
2014-09-06张海斌
张海斌, 贾 梅, 陈 强
(上海理工大学 理学院, 上海 200093)
一类半无穷区间上分数阶非线性微分方程边值问题多个正解的存在性
张海斌, 贾 梅, 陈 强
(上海理工大学 理学院, 上海 200093)
考虑一类具有Caputo导数的分数阶非线性微分方程在半无穷区间上的边值问题, 用Schauder不动点定理和Leggett-Williams不动点定理分别得到了该边值问题至少1个正解和至少3个正解的存在性定理.
Caputo导数; 正解; 不动点定理; 半无穷区间
0 引 言
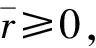
半无穷区间上的微分方程边值问题及分数阶微积分在应用数学和物理等领域应用广泛.文献[1-5]讨论了半无穷区间上整数阶边值问题解或正解的存在性; 文献[6-10]给出了分数阶微积分方程的相关结果; 文献[10]研究了一类半无穷区间上具有Riemann-Liouville分数阶导数α阶微分方程边值问题正解的存在性, 其中1<α≤2.但目前对于半无穷区间上具有Sturm-Liouville边界条件的分数阶微分方程边值问题正解的存在性研究报道较少.
本文考虑半无穷区间上分数阶Sturm-Liouville边值问题:

注1由p(s)的定义可知,M>0.
假设如下条件成立:
(H1) 存在函数h∈C([0,+∞),(0,+∞)),v∈C((0,+∞),(0,+∞)), 满足
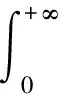

1 引 理
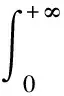
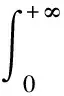
证明: 在式(3)两边同时积分得
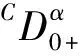
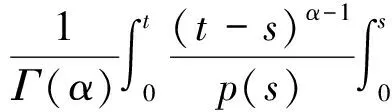
根据边界条件(4)可得
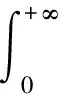
令
则
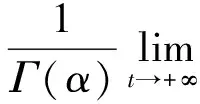
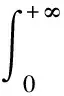
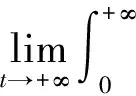
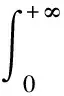
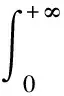
引理2设假设条件(H0)成立, 则对任意的t,s∈[0,+∞)有G(t,s)≥0, 且G(t,s)是连续的, 并满足:
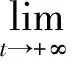
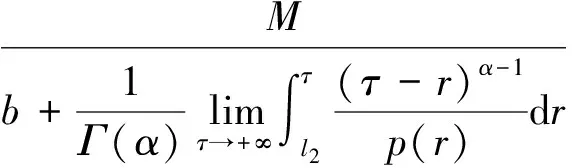
证明: 当0≤t 所以对任意的t,s∈[0,+∞)有G(t,s)≥0. 由G(t,s)的表达式易知G(t,s)在t,s∈[0,+∞)上是连续的. 1) 由G(t,s)的表达式, 易得结论成立. 显然0<γ0<1.所以, 对任意的t∈[l1,l2]⊂(0,+∞)和s∈[0,+∞),G(t,s)≥γ0G(s,s). 引理3[6]若V⊂E, 且如下条件成立: 1)V在E中一致有界; 3)V在+∞处等度收敛, 即对任意的ε>0, 存在T(ε)>0, 使得对任意的z∈V, 当t≥T(ε)时, 成立|z(t)-z(+∞)|<ε. 则V是E中相对紧集. 定义算子A为 引理4若假设条件(H0),(H1)成立, 则算子A:E→E是全连续算子. 若z∈E, 则存在r0>0, 使得‖z‖≤r0.设t∈[0,+∞), 则 所以A:E→E连续. 设Ω⊂E是任意一个有界集, 则存在r>0, 使得当z∈Ω时, 有‖z‖≤r.对任意的z∈Ω, 可得 所以A(Ω)是一致有界的. 因此,A(Ω)在+∞处是等度收敛的.由引理3知A是相对紧的, 故A:E→E是全连续的. 引理5(Schauder不动点定理)[11]设S是Banach空间E的有界凸闭集, 而A:S→S是全连续的, 则A在S中必有不动点, 即存在x*∈S, 使得A(x*)=x*. 设P是实Banach空间E中一个锥.考虑P上一个非负连续凹泛函φ(x), 记PK={x∈P: ‖x‖ 1) {y∈P(φ,L,K):φ(y)>L}≠Ø, 且当y∈P(φ,L,K)时, 恒有φ(Ay)>L; 3) 当y∈P(φ,L,R)且‖Ay‖>K时, 恒有φ(Ay)>L. 注2当K=R时, 条件1)包含条件3). 定理1若假设条件(H0)~(H2)成立, 则边值问题(1)-(2)至少存在一个正解z(t), 满足: 0≤z(t)≤R,t∈[0,+∞), 其中R在(H2)中定义. 证明: 记S={z:z∈E,‖z‖≤R}.对任意的z∈S, 有 即A(S)⊂S.由引理4知, 算子A:S→S是全连续算子. 定理2若假设条件(H0)~(H2)成立, 存在常数r,L>0, 0 3) 存在u∈C((0,+∞),(0,+∞))满足u(t)h(x)≤f(t,x),u(t)≥k0v(t), 0 所以, 引理6的条件2)满足. 下证引理6的条件1)满足.令z(t)=(L+R)/2,t∈[0,+∞).由P(φ,L,R)的定义知,z∈P(φ,L,R).所以{z∈P(φ,L,R):φ(z)>L}≠Ø.若z∈P(φ,L,R), 由条件2),3)和引理2得 因此引理6的条件1)满足.由注2知条件3)满足. 由Leggett-Williams不动点定理知, 边值问题(1)-(2)至少存在3个正解z1,z2,z3, 且满足 例1考虑如下边值问题: 例2考虑如下边值问题: 其中:b=2;p(t)=et;f(t,z)=v(t)h(z), [1]SUN Yan, SUN Yongping, Debnath L.On the Existence of Positive Solutions for Singular Boundary Value Problems on the Half Line [J].Appl Math Lett, 2009, 22(5): 806-812. [2]WANG Ying, LIU Lishan, WU Yonghong.Positive Solutions of Singular Boundary Value Problems on the Half Line [J].Appl Math Comput, 2008, 197(2): 789-796. [3]Yoruk F, Hamal N A.Existence Results for Nonlinear Boundary Value Problems with Integral Boundary Conditions on an Infinite Interval [J/OL].2012-01-05.http://www.boundaryvalueproblems.com/content/2012/1/127. [4]LIAN Hairong, GE Weigao.Existence of Positive Solutions for Sturm-Liouville Boundary Value Problems on the Half-Line [J].Math Anal Appl, 2006, 321(2): 781-792. [5]XI Shouliang, JIA Mei, JI Huipeng.Multiple Positive Solutions for Boundary Value Problems of Second-Order Differential Equations System on the Half-Line [J].Electron J Qual Theory Differ Equ, 2010, 2010(17): 1-15. [6]白占兵.分数阶微分方程边值问题理论及应用 [M].北京: 中国科学技术出版社, 2012.(BAI Zhanbing.Theroy and Applications for Boundary Value Problems of Fractional Differential Equations [M].Beijing: China Science and Technology Press, 2012.) [7]金京福, 刘锡平, 窦丽霞, 等.分数阶微分方程积分边值问题正解的存在性 [J].吉林大学学报: 理学版, 2011, 49(5): 823-828.(JIN Jingfu, LIU Xiping, DOU Lixia, et al.Existence of Positive Solutions for a Class of Fractional Differential Equation with Integral Boundary Value Problems [J].Journal of Jilin University: Science Edition, 2011, 49(5): 823-828.) [8]Podlubny I.Fractional Differential Equations [M].New York: Academic Press, 1999. [9]Kilbas A A, Srivasfava H M, Trujillo J J.Theory and Applications of Fractional Differential Equations [M].North-Holland Mathematics Studies.Vol.204.Amsterdam: Elsevier, 2006. [10]ZHAO Xiangkui, GE Weigao.Unbounded Solutions for a Fractional Boundary Value Problems on the Infinite Interval [J].Acta Appl Math, 2010, 109(2): 495-505. [11]郭大钧, 孙经先, 刘兆理.非线性常微分方程泛函方法 [M].2版.济南: 山东科学技术出版社, 2005.(GUO Dajun, SUN Jingxian, LIU Zhaoli.Functional Methods for Nonlinear Ordinary Differential Equations [M].2nd ed.Jinan: Shangdong Science and Technology Press, 2005.) ExistenceofMultiplePositiveSolutionsforaClassoftheBoundaryValueProblemsofFractionalOrderNonlinearDifferentialEquationontheHalfLine ZHANG Haibin, JIA Mei, CHEN Qiang We studied a class of the boundary value problems for fractional order nonlinear differential equation with Caputo derivative on the half line.Based on the Schauder fixed point theorem and the Leggett-Williams fixed point theorem, the existence theorems of at least one positive solution and at least three positive solutions have been established, respectively for this class of the boundary value problems. Caputo derivative; positive solutions; fixed point theorems; half infinite interval 2014-01-17. 张海斌(1989—), 男, 汉族, 硕士研究生, 从事常微分方程的研究, E-mail: 1042920826@qq.com.通信作者: 贾 梅(1963—), 女, 汉族, 硕士, 副教授, 从事常微分方程的研究, E-mail: jiamei-usst@163.com. 国家自然科学基金(批准号: 11171220)和沪江基金(批准号: B14005). O175.8 A 1671-5489(2014)06-1145-06 10.13413/j.cnki.jdxblxb.2014.06.07 赵立芹)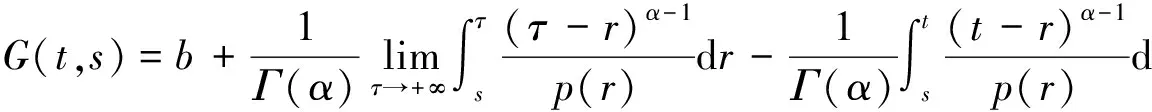
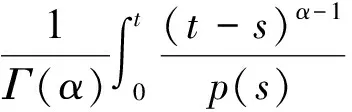
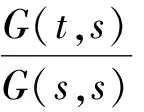
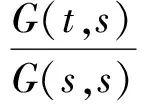
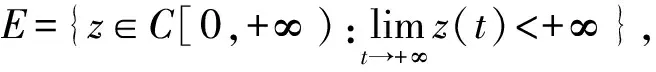
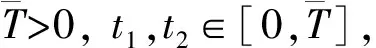
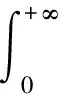

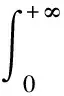
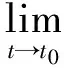

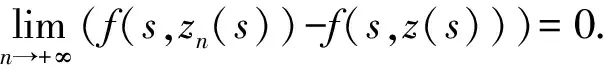
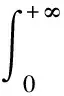
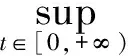
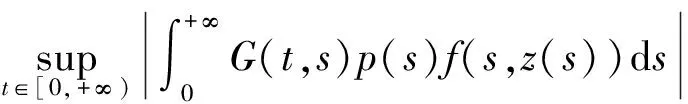
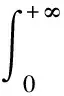
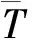


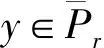
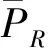
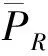
2 至少1个正解和3个正解的存在性
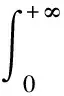
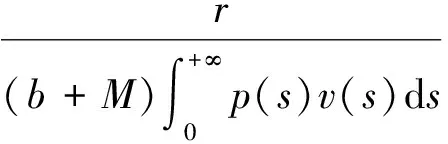

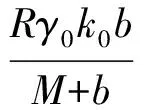

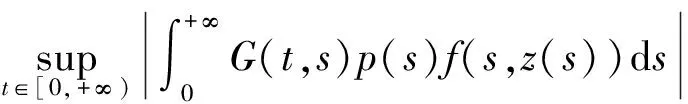
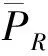
3 应用实例
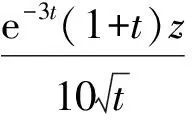
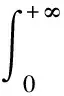
(CollegeofScience,UniversityofShanghaiforScienceandTechnology,Shanghai200093,China)
