(2+1)维非线性发展方程的对称约化及精确解
2013-10-26李宁刘希强张颖元
李宁, 刘希强, 张颖元
(2+1)维非线性发展方程的对称约化及精确解
*李宁, 刘希强, 张颖元
(聊城大学数学科学学院,山东,聊城 252059)
利用相容性方法,得到了(2+1)维mKdV-KP的非经典对称及相似约化,并进一步得到了该方程的一些新的精确解,包括双曲函数解,三角函数解,有理函数解,椭圆函数解等。
相容性方法;(2+1)维mKdV-KP方程;精确解;对称;相似约化
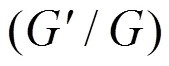
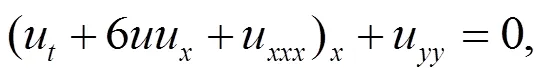
即
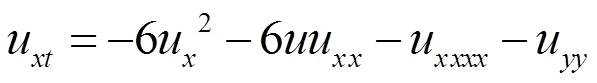
方程(2)的显式解。(2+1)维mKdV-KP方程是基于(2+1)维KdV和(2+1)维KP方程提出的推广。 A. S. Al-Fhaid利用F展开法[10]得到了该方程的雅可比双周期波解,N Taghizadeh等用首次积分法[11]得到了该方程的行波解。文献[4]中作者利用修正的CK直接方法得到了非线性发展方程的对称,从而约化方程,但此方法需要复杂的计算和转化过程。文献[6]中作者利用经典李群方法得到了非线性发展方程的李点对称,也达到约化方程的目的,但此方法在求解生成元时需大量的计算。虽然相容性方法的最终目的同样是寻找非线性发展方程的对称,但该方法能够简化计算过程,并能得到更多新的结果。本文的求解过程恰能说明相容性方法的优越性。
相容性方法的主要思想是假定方程(2)有如下形式
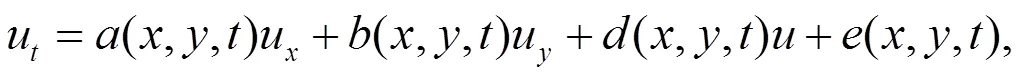
1 (2+1)维mKdV-KP方程的非经典对称
把(3)式带入(2)式,得到
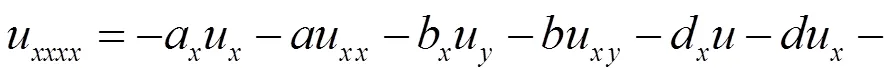

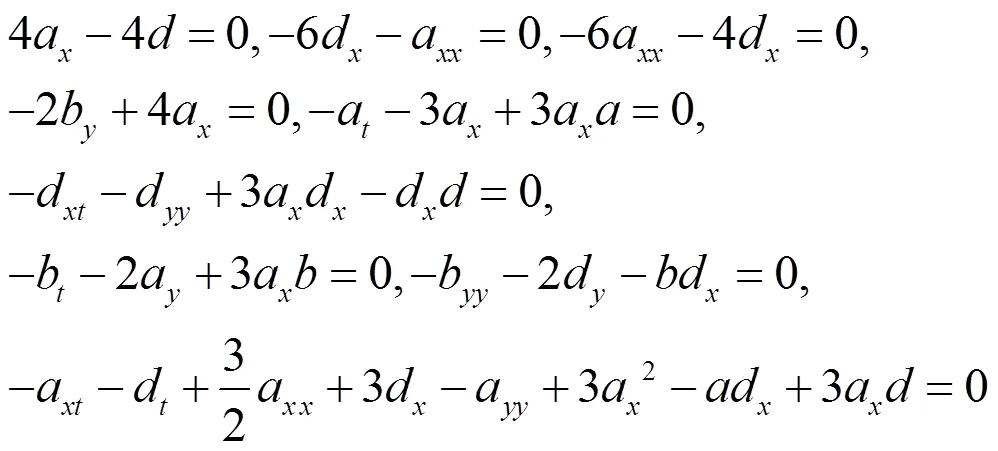
解上述超定方程组可得

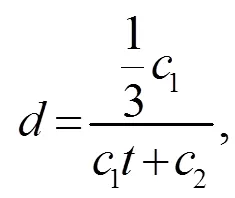
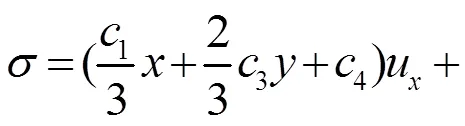
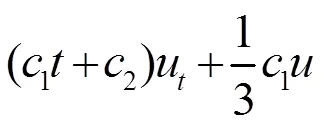
2 (2+1)维mKdV-KP方程的对称约化以及显式解
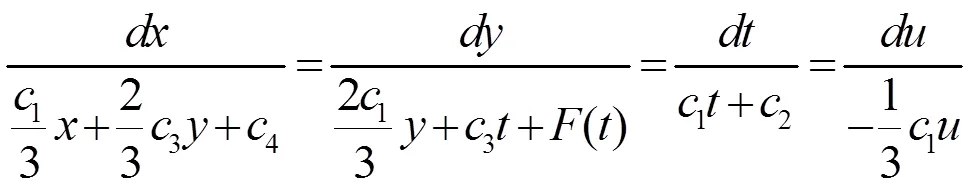
分以下3种情况讨论:
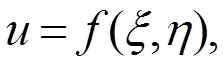


以下用扩展的tanh方法求解方程(12)。假设方程(12)有以下形式的解
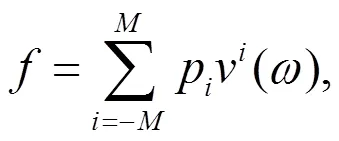
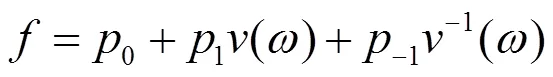
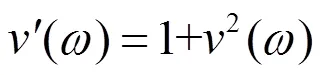
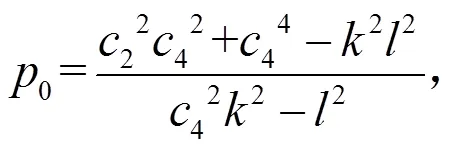
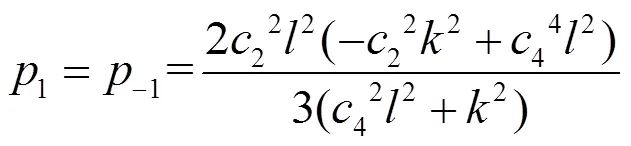
则方程(12)的解为

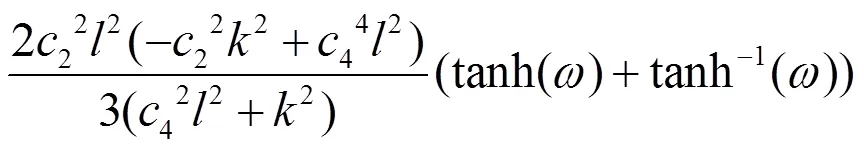
即原方程(2)的解为
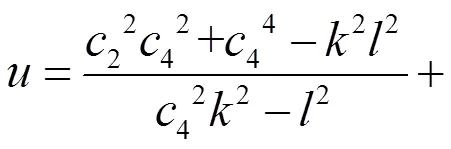
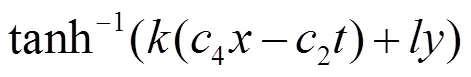
为了得到方程(2)更广泛的解,将(12)式积分两次可以得到
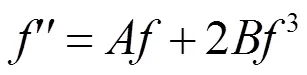
由(19)式容易得到
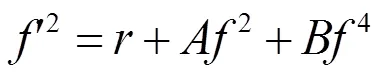

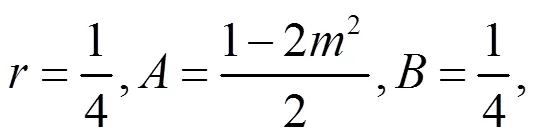
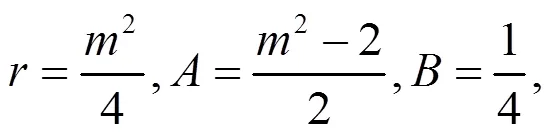
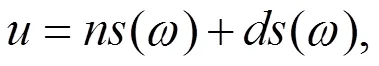
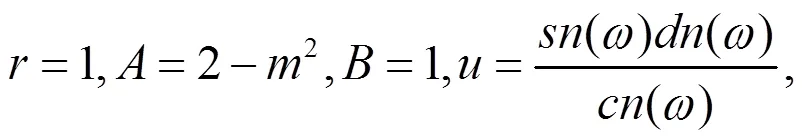

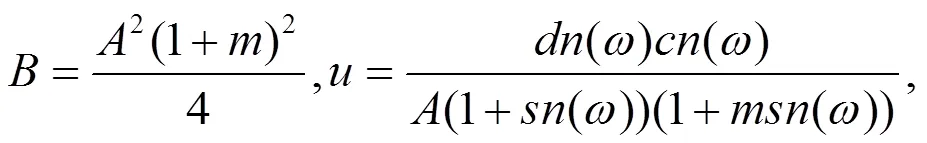
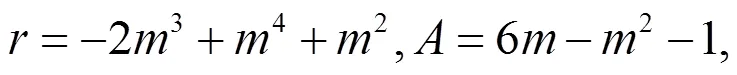
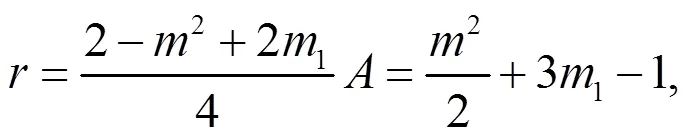
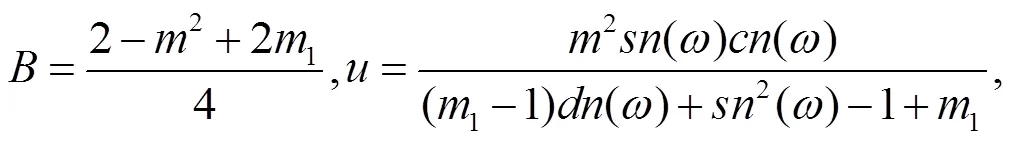
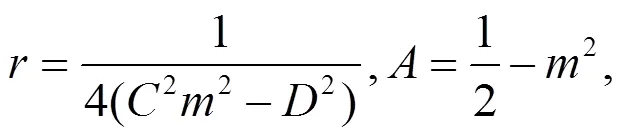
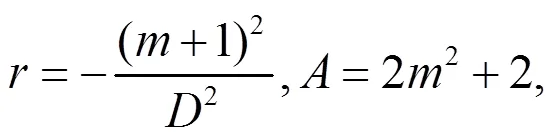
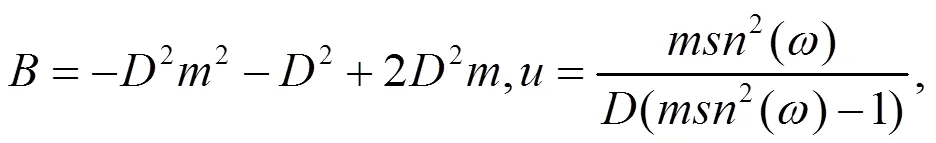


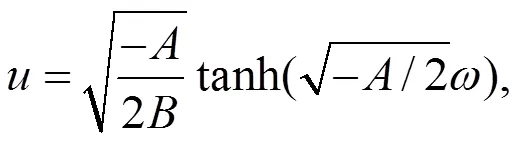
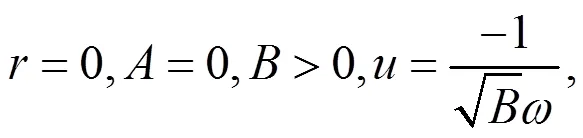
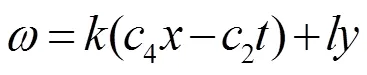
注1:情况1 中还有大量解,这里不再一一列出,而且都是新解。

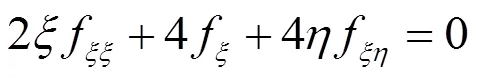
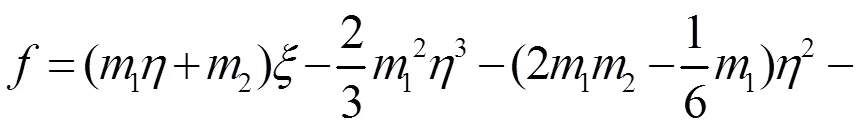
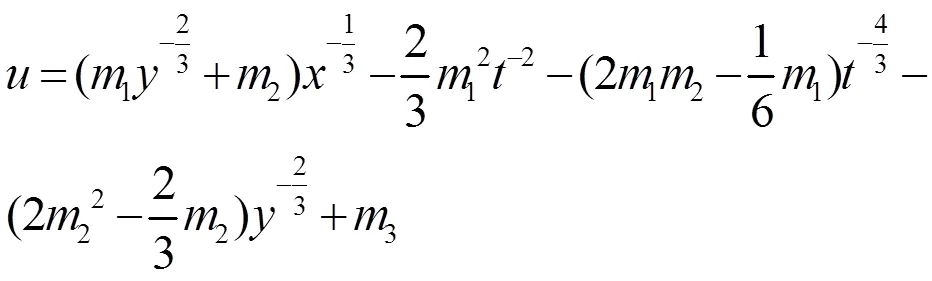
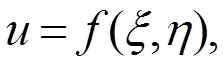
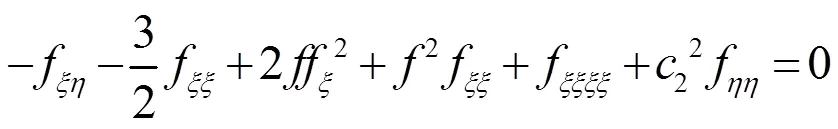
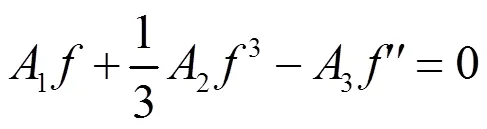
以下借助辅助函数[13]来求解方程(23)的解。设方程(23)有以下形式的解
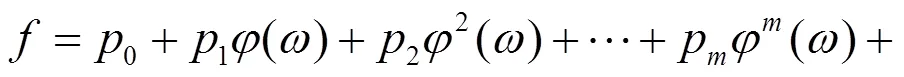
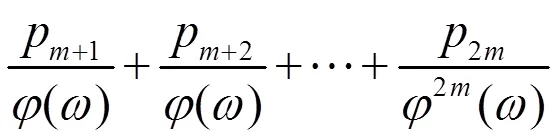
利用辅助方程
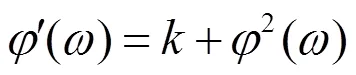
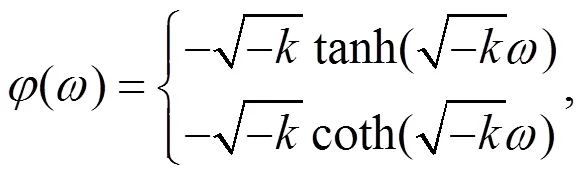

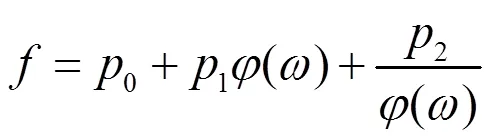
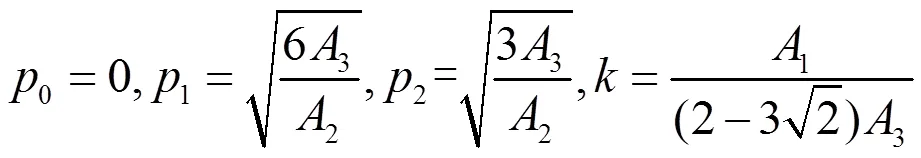
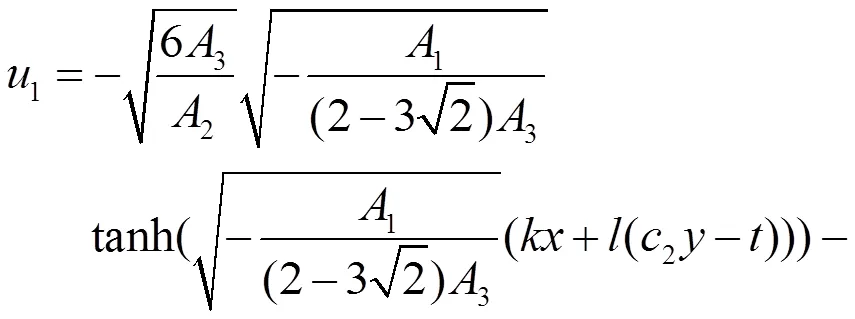
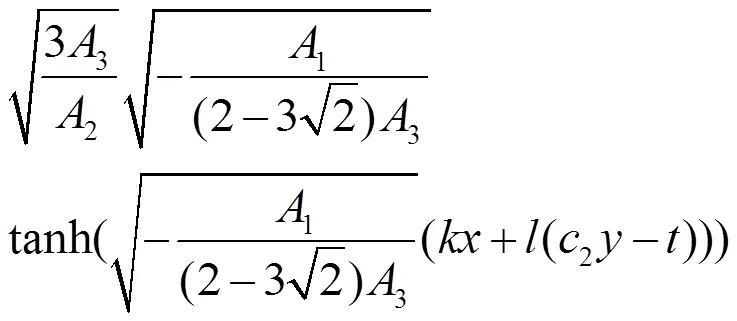
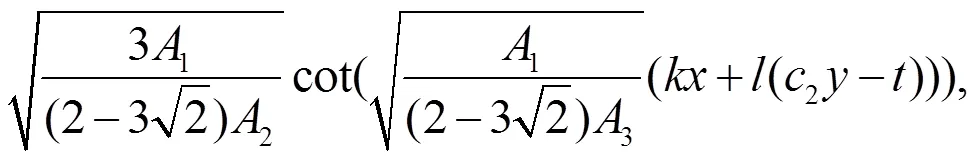
方程(22)也可以约化为
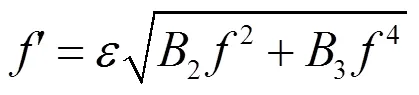
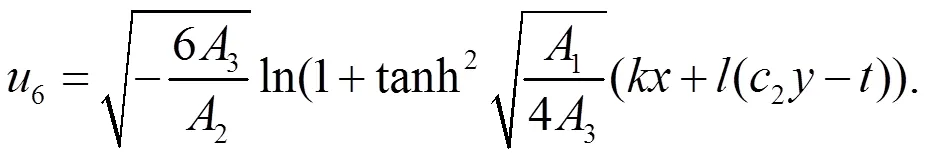
注2:经过Maple数学软件验证情况3中的6组解都是方程(2)的解,而且均是新解。
3 结论
利用相容性方法得到了mKdV-KP方程的非经典对称,并利用其得到了该方程的相似约化,通过解约化方程得到了mKdV-KP方程的一些精确解。
[1] Ye Caier. New Exact Solutions for the Generalized mKdV Equation with Variable Coefficients[J]. Applied Mathematical Sciences,2011,5(75): 3715-3721.
[2] Gai Xiao-ling, Gao Yi-tian, Meng De-xin, et al. Darboux Transformation and Soliton Solutions for a Variable Coefficient Modified Kortweg-deVries Model from Fluid Mechanics, Ocean Dynamics, and Plasma Mechanics [J]. Commun. Theor. Phys., 2010,53(4): 673- 678.
[3] Rady A S , Osman E S. Multiple soliton for the system of coupled KdV equations[J]. Appl.Math. Comput., 2009 (210): 177-181.
[4] 张琳琳,辛祥鹏.(2+1)维修正KP方程的精确解[J]. 聊城大学学报:自然科学版,2009,22(3):9-13.
[5] 刘文健,刘希强,桑波.非线性发展方程的新精确解[J].西北师范大学学报:自然科学版,2012,48(2):31-36.
[6] 王刚伟,刘希强,张颖元.变系数mKdV方程的精确解[J].井冈山大学学报:自然科学版,2012,33(5):1-5.
[8] Wazwaz A M. Solitons and singular solitons for the Gardner-KP equation[J]. Appl. Math. Comput, 2008 ,204:162- 169.
[9] Liu Na, Liu Xi-qiang, Similarity reduction and solutions of the (3+1)-dimensional Kadomtsev- petviashvili equation[J].Phy Lett A,2008,25:3527- 3530.
[10] Al-Fhaid A S. [PDF]New exact solutions for the modified KdV-KP equation using the extended F-expansion method[J]. Applied Mathematical Sciences,2012,6: 5315- 5332.
[11] Taghizadeh N, Mirzazadeh M , Farahrooz F. The first integral method for some time fractional differential equations[J]. Appl. Math. Model, 2011,35: 3991.
[12] Bai Cheng-lin, Bai Cheng-jie , Zhao Hong. A new generalized algebraic method and its application in nonlinear evolution equations with variable coefficients [J]. Z. Naturforsch, 2005, 60a: 211-220.
[13] Yan Zhen-ya. The Riccati equation with variable coefficients expansion algorithm to find more exact solutions of nonlinear differential equations Computer Physics Communications 2003,152:1-8.
[14] Huang Ding-jiang, Li Deng-sheng , Zhang Hong-qing. Explicit and exact traveling wave solutions for the generalized derivative Schrödinger equation [J]. Chaos, solitions and Fractals,2007,31:586-593.
SYMMETRY REDUCTIONS AND EXACT SOLUTIONS OF (2+1)-DIMENSIONAL NONLINEAR EVOLUTION EQUATION
*LI Ning,LIU Xi-qiang,ZHANG Ying-yuan
(School of Mathematical Sciences, Liaocheng University, Liaocheng, Shandong 252059, China)
Based on the compatibility method, we find the non-classical symmetry, reductions and new exact solutions of mKdV-KP equation including the hyperbolic functions, the trigonometric functions, the rational functions and the elliptic functions and so on.
the compatibility method; (2+1)-dimensional mKdV-KP equation; exact solutions; symmetry; similar reduction
O175. 2
A
10.3969/j.issn.1674-8085.2013.03.002
1674-8085(2013)03-0005-05
2012-11-21;
2013-03-14
国家自然科学基金和中国工程物理研究院联合基金项目(11076015)
*李 宁(1981-),男,山东枣庄人,硕士生,主要从事非线性偏微分方程解的研究(E-mail:ln1011@163.com);
刘希强(1957-),男,山东菏泽人,教授,博士,主要从事非线性微分方程系统研究(E-mail: liuxiq@sina.com);
张颖元(1987-),女,山东济南人,硕士生,主要从事非线性偏微分方程解的研究(E-mail:zhangyingyuanok@126.com).
