关于Lucas多项式平方和的恒等式

杨瑞妮,董晓茹

(西北大学数学系,陕西西安 710127)

关于Lucas多项式平方和的恒等式

杨瑞妮,董晓茹

(西北大学数学系,陕西西安 710127)

利用广义Lucas多项式Ln(x,y)的性质,通过构造组合和式Tn(x,y;tx2),结合Bernou lli多项式的生成函数和Euler多项式的生成函数,采用分析学中的方法,得到两个有关L2n(x,y)的恒等式.并从这一结果出发,得到了两个推论,推广了相关文献的一些结果.

Lucas多项式;Bernoulli多项式;Euler多项式

DO I：10.3969/j.issn.1008-5513.2013.04.014

1 引言及其结论

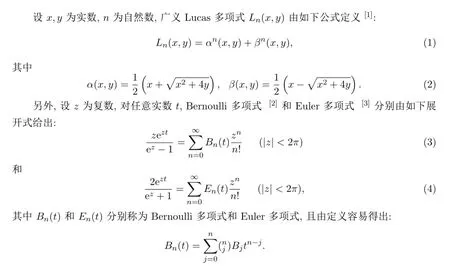
2 引理及其证明

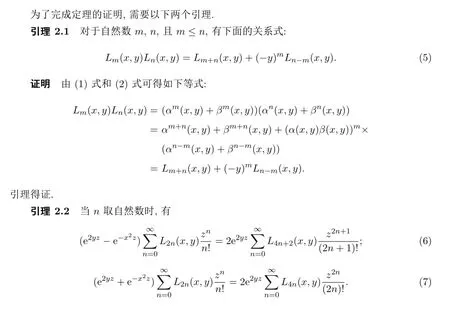
3 定理的证明

两个定理得证.

类似地,利用以上的方法和步骤还可以对有关Lucas多项式的更高次恒等式进行推导,进而获得更为一般的推广结果.巧妙的构造法和分析学中的方法值得在以后的研究中将其推广,应用到更多的多项式中去,例如切比雪夫多项式[6].

[1]Claudio de Jesus Pita Ruiz Velasco.A Note on Fibonacci&Lucas and Bernoulli&Eu ler Polynom ials[J]. Journal of Integer Sequences,2012,15:1-17.

[2]Ireland K,Rosen M.A classical introduction tomodern number theory[M].znd ed.New York:Sp ring-Verlag, 1990.

[3]Tom M Apostol.Introduction to Analytic Number Theory[M].New York:Spring-Verlag,1976.

[4]Sun Zhiwei,Pan Hao.Identities concerning Bernou lliand Eu ler polynom ials[J].Acta A rith,2006,125:21-39.

[5]Pan Hao,Sun Zhiwei.New identities involving Bernou lli and Eu ler polynom ials[J].Combin.Theory Ser. A,2006,125:156-175.

[6]刘国栋,罗辉.一些包含Chebyshev多项式和Stirling数的恒等式[J].纯粹数学与应用数学,2010,26(2): 177-182.

Som e iden tities on the quad ratic sum of Lucas polynom ials

Yang Ruini,Dong Xiaoru
(Department of Mathematics,Northwest University,X i′an 710127,China)

W ith the properties of generalized Lucas polynom ials Ln(x,y)and themethod of analysis,we obtain a list of identities connecting the sum of L2n(x,y)by work ing w ith Tn(x,y;tx2)and generating functions of Bernou lli polynom ials Bi(t)and Eu ler polynom ials Ei(t).Simultaneously,some corollaries of these resu lts can be easily estab lished.Thus some results obtained in some essays are generalized.

Lucas polynom ial,Bernou llipolynom ial,Eu ler polynom ial

O156.4

A

1008-5513(2013)04-0433-08

2013-05-12.

陕西省教育厅科学研究项目(11JK 0470).

杨瑞妮(1987-),硕士生,研究方向：数论.

2010 M SC:11B39